Isointerval chord

from Wikipedia, the free encyclopedia

Isointerval chords (also symmetrical chords , cyclical chords , infinite chords ) are chords that are completely made up of equal intervals . They are symmetrical in themselves , i.e. H. none of its tones stand out from any other; therefore they do not have a clearly definable keynote . This term, which was only established at the beginning of the 21st century, assumes that the octave is divided into twelve equal semitones, as in the case of equal tuning. Such chord types only exist within the major-minor tonality if individual notes of the chord are reinterpreted enharmonically . In doing so, however, they are no longer to be regarded as tonally identical. The intonation of the chords changes.

Basic structure of isointerval chords

An isointerval chord is shown here using the example with minor thirds:

diminished seventh chord

All tones are spaced three semitones apart (called minor thirds here). The chords consist of equal intervals. Adding one more of these intervals creates a note that is already present in the chord.

Example: The chord hdf-as consists of minor thirds. By adding another minor third (it is actually an excessive second), the note b is reached again, resulting in the identical chord hdf-a-flat-b .

This results in chords with identical intervals of three semitones each.

  • df as h
  • hdf as
  • as hdf
  • f as hd

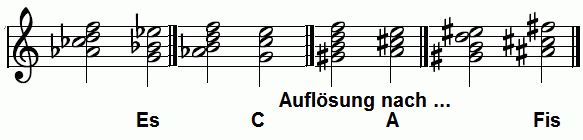
However, this only applies in the equal-grade mood. The piano etc. with its equal tuning is known to be a compromise in intonation. Strictly speaking, in major minor tonal music, these intervals - known as diminished seventh chords - are not isointerval chords. The individual tones of these chords are confused enharmonically when they are reinterpreted and therefore have (at least theoretically) a different intonation , namely:

  • df as ces (resolution to E flat major / minor)
  • hdf as (resolution to C major / minor)
  • gis hdf (resolution to A major / minor)
  • eis g sharp hd (resolution to F sharp major / minor)

In the same pitch as possible:

|aaa
Audio file / audio sample Listening in an equal mood ? / i       listening in a pure mood ? / iAudio file / audio sample

Types

If you consider intervals only with the same semitone steps, the following types of isointerval chords result:

  • Intervals with 6 semitones (tritone): For example: c-f sharp . Add c-f sharp-c to the octave. Enharmonic reinterpretation: tritone (excessive fourth) = diminished fifth (f sharp-c).
  • Intervals with 4 semitones (major thirds): For example: ce-g sharp . Added to the octave: ce-g sharp-c. Enharmonic reinterpretation: major third = diminished fourth (g sharp-c).
  • Intervals with 3 semitones (minor thirds): Example: c-es-f sharp-a . Added to the octave: c-es-fis-ac. Enharmonic reinterpretation: minor third = excessive second (es-f sharp). See also the detailed example hdf-as above .

In purely formal terms, isointerval chords can be formed with intervals of two or one semitone apart. However, these clusters are only significant in the 12-tone music of the 20th century. Enharmonic reinterpretations are not possible here.

Web links

  • Hans Hansen: Isocord Theory. Young Composers. 2009, accessed March 21, 2020 .

literature

  • Reinhard Amon: Lexicon of harmony. Reference work on major minor harmony with analysis codes for functions, levels and jazz chords. Doblinger et al. a., Vienna a. a. 2005, ISBN 3-900695-70-9 , pp. 129-131.