Planetary loop
A planetary loop or opposition loop describes the phenomenon that every upper planet ( Mars to Neptune ) in the months around its opposition , i.e. H. when it forms an angle of 180 ° with the sun when viewed from the earth, it appears to wander back in the starry sky in order to continue its right-hand orbit later. This happens because the earth is faster on the "inner orbit". As a result, the observed planet seems to stand still and its direction of movement then reverses. Every lower planet ( Mercury and Venus ) also shows this behavior in the area of its lower conjunction , because this then overtakes the earth.
In ancient Babylon , the backward movements of the planets were predicted by extrapolating their previous orbits. In Hellenism , astronomers developed a sophisticated theory of planetary motions , the so-called epicyclic theory , which allowed a very precise forecast of planetary loops. In the early modern era, in the heliocentric system of Nicolaus Copernicus , the planetary loops were finally explained in a way that we still believe to be correct today: when one of the upper planets is facing the sun (technical term: "is in opposition") the earth is between him and the sun. Due to its faster orbit, the earth overtakes the more distant planet inside, so that it seems to move backwards ("retrograde") for a few weeks .
If the earth moves exactly towards the planet before overtaking, it seems to stand still for a few days against the background of the fixed stars (in the picture point S1). A few months later, after the Earth has overtaken the planet, it runs away from the planet and a second deadlock occurs in S2.
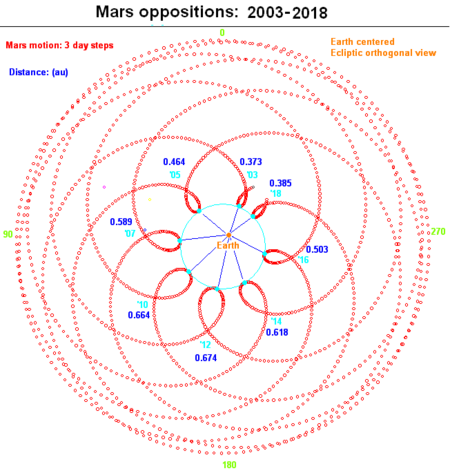
These turning points, at which a planet hardly moves under the stars for a few days, are called “standstill”. However, since the planets' orbital planes do not exactly coincide with the ecliptic , strictly speaking it is a standstill in ecliptical length. Changes in the ecliptical latitude during standstill give the apparent trajectory a loop shape instead of real turning points. Such opposition loops can be observed very well on Mars .
If two planets wander through the same constellation around their opposition , it can happen that their loops overlap and they meet not just once but three times ; this is called a threefold conjunction . Every few centuries this occurs for Jupiter and Saturn . It is sometimes suggested that such an event underlies the description of the Star of Bethlehem in the New Testament . Matthew chap. 2, the remark that “the star stood still” over Bethlehem could indicate exactly when the two planets reversed their course on almost the same day. This constellation can be traced back to the year 7 BC. Calculate back.
Such phenomena can be investigated with 2D methods in the discussion of curves (two-dimensional) or with 3D methods of path determination . In such a spatial process z. It is understandable, for example, that the earth moves away from or towards the other planet in the times of S1 or S2 .
Construction of a planetary loop
The adjacent figure shows the apparently loop-shaped path for the movement of the observer on the earth moving around the sun. The loop-shaped orbit results from a projection of the movement of the relatively close planet onto the background of the fixed stars, which are almost infinitely distant. It results from the parallax between the earth, planet and fixed star background.
In half a year the earth will cover the distance from points 2 to 7 (see figure below). In the same period of time, the planet that is further away from the Sun (i.e. upper) covers a shorter distance due to the lower mean orbital velocity , which is also significantly less curved due to the greater distance.
If you now connect the points at the corresponding points in time and extend the ray to the fixed star background, there is apparently a concurrent movement from points 1 to 3, from points 3 to 5 a retrograde movement and from points 5 to 7 again a concurrent movement. The corresponding projection onto the fixed star background results in the loop-shaped path.
Self-experiment
Two people can easily simulate the effect of the apparent direction reversal in open terrain. On a larger free space, mark a spot as a sun (e.g. with a stone). At a distance of a few meters from the sun, a person walks on a circular path around the sun. At a distance of, for example, 10 meters, a second person walks much more slowly on a circular path around the sun. The inner person then constantly looks at the outer while walking. The inner person also pays attention to objects at a great distance, such as trees or towers. For the inner person, when overtaking the outer person, it is then clearly visible how the outer person is first moving towards a church tower, for example, then backwards away from it, and then runs towards it again at increased speed.
See also
Web links
- Explanation in Meyers Konversationslexikon, 4th edition, 1885-1892, pages 108 and 109
- Demonstration of retrograde planetary motion (with Mathematica )