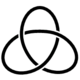
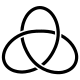
Amphichiral knot

In the mathematical field of knot theory , an amphichiral knot (also: achiral knot ) is a knot that is equivalent to its mirror image. A chiral knot is a knot that is not equivalent to its mirror image.

Amphichiral knots are important in biology and chemistry because the chirality or achirality of molecules is of great importance for their physical and chemical properties. Chirally knotted molecules in pharmaceutical products led to severe damage to newborns in the 1950s because mirror-inverted molecules often work in completely different ways. This problem does not occur with amphichirally knotted molecules.

Formal definition

A node is an equivalence class of embedding (or when), two embeddings are considered equivalent an orientation-preserving homeomorphism of (or are), which reflects the embedding into the other. ${\ displaystyle S ^ {1} \ to \ mathbb {R} ^ {3}}$ ${\ displaystyle S ^ {1} \ to S ^ {3}}$ ${\ displaystyle \ mathbb {R} ^ {3}}$ ${\ displaystyle S ^ {3}}$

The mirror image of a node is obtained by applying an orientation-reversing homeomorphism to the image of an embedding representing the node. Because all orientation-reversing homeomorphisms of the (or ) are homotopic to one another, this definition does not depend on the choice of the orientation-reversing homeomorphism. ${\ displaystyle \ mathbb {R} ^ {3}}$ ${\ displaystyle S ^ {3}}$

The mirror image of the node is denoted by. ${\ displaystyle K}$ ${\ displaystyle K ^ {*}}$

A knot is called amphichiral (or achiral) if it is equivalent to its mirror image, i.e. if there is not only an orientation-reversing, but also an orientation-preserving homeomorphism, which maps the knot onto its mirror image.

A knot is said to be chiral if it is not equivalent to its mirror image.

Examples of chiral and amphichiral knots

The left-handed shamrock loop.
The right-handed shamrock loop.

The trefoil loop is a chiral knot: the left-handed trefoil loop is not equivalent to the right-handed trefoil loop.

More generally, all torus knots are chiral knots.

The figure eight knot is amphichiral.

Invariants

The following invariants can distinguish a node from its mirror image:

Signature : the signature of the mirror image has the opposite sign, the signature of an amphichiral knot is therefore zero. With this invariant one can distinguish the mirror images of torus knots (e.g. the clover leaf loop) from the original knots.

Chern-Simons invariant : the Chern-Simons invariant of the mirror image has the opposite sign, the Chern-Simons invariant of an amphichiral knot is therefore zero.

Number of crossings : the modulo 2 reduction of the number of crossings of a node is opposite to that of the mirror image, so the number of crossings of an amphichiral node is an even number

Jones polynomial : the Jones polynomial of the mirror image is obtained by substituting into the Jones polynomial of , i. H. . The Jones polynomial of an amphichiral knot is therefore palindromic. ${\ displaystyle V_ {K ^ {*}}}$ ${\ displaystyle K ^ {*}}$ ${\ displaystyle t ^ {- 1}}$ ${\ displaystyle K}$ ${\ displaystyle V_ {K ^ {*}} (t) = V_ {K} (t ^ {- 1})}$

Rasmussen's s-invariant , see Khovanov homology .

τ-invariant from Ozsváth and Szabó, see Heegaard-Floer homology .

Related terms

Number of nodes of each chirality type for a given number of intersections
Intersection number	3	4th	5	6th	7th	8th	9	10	11	12	13	14th	15th	16
Chiral knots	1	0	2	2	7th	16	49	152	552	2118	9988	46698	253292	1387166
Reversible knot	1	0	2	2	7th	16	47	125	365	1015	3069	8813	26712	78717
Completely chiral knots	0	0	0	0	0	0	2	27	187	1103	6919	37885	226580	1308449
Amphichiral knots	0	1	0	1	0	5	0	13	0	58	0	274	1	1539
Positive amphichiral knots	0	0	0	0	0	0	0	0	0	1	0	6th	0	65
Negative amphichiral knots	0	0	0	0	0	1	0	6th	0	40	0	227	1	1361
Completely amphichiral knots	0	1	0	1	0	4th	0	7th	0	17th	0	41	0	113

literature

Gilbert, ND; Porter, T .: Knots and surfaces. Oxford Science Publications. The Clarendon Press, Oxford University Press, New York, 1994. ISBN 0-19-853397-7 .
Murasugi, Kunio: Knot theory & its applications. Translated from the 1993 Japanese original by Bohdan Kurpita. Reprint of the 1996 translation. Modern Birkhäuser Classics. Birkhauser Boston, Inc., Boston, MA, 2008. ISBN 978-0-8176-4718-6 .

Web links

Amphichiral Knot (MathWorld)

Individual evidence

^ Ross S. Forgan / Jean-Pierre Sauvage / J. Fraser Stoddart, "Chemical Topology: Complex Molecular Knots, Links, and Entanglements", in: Chemical Reviews 111 (2011), 5434-5464
↑ Stereochemistry: Determining Molecular Chirality

[1] Ross S. Forgan / Jean-Pierre Sauvage / J. Fraser Stoddart, "Chemical Topology: Complex Molecular Knots, Links, and Entanglements", in: Chemical Reviews 111 (2011), 5434-5464

[2] Stereochemistry: Determining Molecular Chirality