Exogenous and endogenous variables
In economic and econometric models , an exogenous variable denotes a variable that is determined outside the model and represents the input of a model. In other words: Exogenous variables are fixed at the moment they are introduced into the model. In contrast, endogenous variables are determined within the model and thus represent the output of a model. The model specified with the variables shows how the change in an exogenous variable, ceteris paribus, affects all endogenous variables.
In general, explanatory variables that are not correlated with the confounding variable of a regression function are also called exogenous variables.
In one-equation models, the terms "explained variable (regressand) - endogenous variable" and "explanatory variable (regressor) - exogenous variable" are identical. In contrast, in multi-equation models, endogenous variables can be both regressors and regressands; however, exogenous variables are only regressors.
Example: supply-demand model
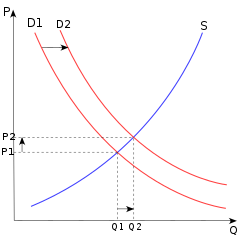
If an economist wants to find out what determines the price and quantity of bicycles sold, then he creates an economic model. This model should describe the behavior of the bicycle supplier, the bicycle customer and the interdependencies on the market. The economist could, for example, set up a model in which the Requested quantity or amount of bicycles from the bicycle price and the disposable income of consumers depends: . Here denotes the demand function . Here he could specify the model meaning that the bike offer bicycle shops by bicycle price and also on the prices of bicycle parts (Dynamo, reflectors, etc.) depends on: . Here is the supply function . Furthermore Des represents is believed that the bicycle market is in equilibrium : . The bicycle market model contains two exogenous variables (price of individual bicycle parts and disposable income of the consumer ) and two endogenous variables (bicycle price and the number of bicycles traded ). The model aims to explain the endogenous variables. On the other hand, the exogenous variables are not to be explained: they are taken for granted. This model can show how the change in one of the two exogenous variables (ceteris paribus) influences the two endogenous variables. If one assumes that the disposable income of the consumers increases, then the demand for bicycles increases. Simultaneously, the rising equilibrium price and the equilibrium quantity . Analogously: If the prices of the individual bicycle parts rise, then the bicycle supply decreases and the equilibrium price and the equilibrium quantity decrease.
Exogenous and endogenous explanatory variables
In regression analysis and econometrics, an exogenous explanatory variable is an explanatory variable that is uncorrelated with the confounding variable (so-called exogeneity ). An endogenous explanatory variable in a multiple regression model is an explanatory variable that is correlated with the disturbance variable either due to an omitted variable , a measurement error, or due to simultaneity (so-called endogeneity ).
Delayed exogenous and endogenous variables
Delayed exogenous and endogenous variables in simultaneous equation models are exogenous or endogenous variables whose values are one or more periods behind ( delay effect ). The delayed exogenous and delayed endogenous variables are among the predetermined variables .
Individual evidence
- ^ A b c Jeffrey Marc Wooldridge : Introductory econometrics: A modern approach. 5th edition. Nelson Education, 2013, p. 848.
- ↑ N. Gregory Mankiw : Macroeconomics: With many case studies , 6th, revised. and additional edition, Schäffer-Poeschel Verlag (2011), p. 9 ff.
- ↑ Jeffrey Marc Wooldridge: Introductory econometrics: A modern approach. 5th edition. Nelson Education, 2013, p. 851.
- ↑ Bernd Rönz and Erhard Förster: Regression and Correlation Analysis : Basics - Methods - Examples. , P. 258.












