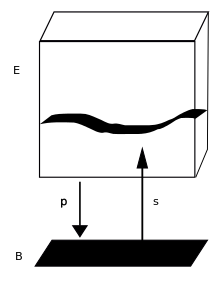Cut (fiber bundle)

Sections are mappings that are examined in algebraic topology , especially in homotopy theory . One is particularly interested in the conditions under which such images exist. Probably the best-known example of cuts are the differential forms .

motivation

A cut can be seen as a generalization of the graph of a function . The graph of a map can be identified with a function with values ​​in the Cartesian product . The function has the form ${\displaystyle g\colon B\to Y}$ ${\displaystyle s\colon B\to E}$ ${\displaystyle E=B\times Y}$ ${\displaystyle s}$

${\displaystyle s(x)=(x,g(x))\in E.}$

If the projection is on the first component, then applies . As the following definition will show, is a special case of a cut. ${\displaystyle \pi \colon E=B\times Y\to B}$ ${\displaystyle \pi (s(x))=x}$ ${\displaystyle s}$

With the help of cuts in fiber bundles, the above construction can also be generalized to sets that do not consist of Cartesian products. ${\displaystyle E}$

definition

The figure s is a cut in a fiber bundle p  : E → B . This intersection s allows the base space B to be identified with the subspace s ( B ) of E.

cut

Let it be a fiber bundle consisting of the total space , the base space , the bundle projection and the fiber . A (global) section in a fiber bundle is a continuous mapping such that ${\displaystyle (E,B,\pi ,F)}$ ${\displaystyle E}$ ${\displaystyle B}$ ${\displaystyle \pi \colon E\to B}$ ${\displaystyle F}$ ${\displaystyle s\colon B\to E,}$

${\displaystyle (\pi \circ s)(x)=\pi (s(x))=x}$

applies to all . The image is therefore a right inverse to the bundle projection . The set of (global) cuts is often referred to with or with . ${\displaystyle x\in B}$ ${\displaystyle s}$ ${\displaystyle \pi }$ ${\displaystyle \Gamma (B,E)}$ ${\displaystyle \Gamma (E)}$

Cut with a compact carrier

Let it be a vector bundle . A cut is called a cut with a compact carrier, in case there is a compact amount with for . The number of cuts with a compact carrier is indicated with or with . Instead of the addition , the addition is also used. ${\displaystyle (E,B,\pi ,F)}$ ${\displaystyle s\in \Gamma (B,E)}$ ${\displaystyle K\subset B}$ ${\displaystyle s(x)=(x,0)}$ ${\displaystyle x\notin K}$ ${\displaystyle s\in \Gamma _{c}(B,E)}$ ${\displaystyle s\in \Gamma _{c}(E)}$ ${\displaystyle c}$ ${\displaystyle 0}$

Smooth cut

If a smooth manifold , a smooth vector bundle above, and if the figure from the above section is smooth , then one calls a smooth (global) cut. To distinguish it from the previously defined cuts, note this amount of these cuts using . If there is no confusion between smooth and non-smooth cuts, then the addition is often dispensed with . ${\displaystyle B}$ ${\displaystyle E}$ ${\displaystyle B}$ ${\displaystyle s\colon B\to E}$ ${\displaystyle s}$ ${\displaystyle \Gamma ^{\infty }(E)}$ ${\displaystyle \infty }$

Examples

1. Be a trivial bundle of fibers and be the projection on . The cuts in this fiber bundle are of course isomorphic to the continuous functions ${\displaystyle (F\times B,B,\pi ,F)}$ ${\displaystyle \pi \colon F\times B\to B}$ ${\displaystyle B}$ ${\displaystyle s\colon B\to F\times B}$ ${\displaystyle B\to F.}$
2. A vector field on a manifold is a mapping which pairs each point of the manifold with a point of the corresponding tangent space. So the point is mapped to. ${\displaystyle v}$ ${\displaystyle M}$ ${\displaystyle v\colon M\to TM}$ ${\displaystyle p\in M}$ ${\displaystyle x\in T_{p}M}$ ${\displaystyle p}$ ${\displaystyle p\mapsto (p,x=g(p))}$
3. Another well-known example of cuts are the differential shapes . These are cuts in the external power of the cotangent bundle .
4. Let it be a vector bundle , the zero intersection is defined by for all . However, it is of interest when a vector bundle has intersections that are nowhere zero. This question is important, for example, to investigate the orientability of a manifold. An important result of this question is the proposition of the hedgehog . ${\displaystyle \xi }$ ${\displaystyle s(x)=0}$ ${\displaystyle x}$

Local cut

In contrast to the examples above, general fiber bundles do not always have global cuts. That is why it makes sense to define sections locally.

Be an open subset. A local cut in a fiber bundle is an image that also applies to all . ${\displaystyle U\subset B}$ ${\displaystyle (E,U,\pi ,F)}$ ${\displaystyle s\colon U\to E}$ ${\displaystyle \pi (s(x))=x}$ ${\displaystyle x\in U}$

literature

• Edwin H. Spanier: Algebraic Topology. 1. corrected Springer edition, reprint. Springer, Berlin et al. 1995, ISBN 3-540-90646-0 .