Klein bottle

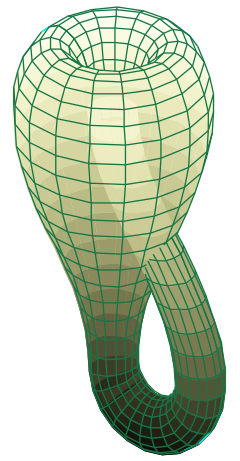
In mathematics, the Klein bottle is a certain non-orientable surface, i.e., a surface (a two-dimensional topological space) with no distinction between the "inside" and "outside" surfaces. The Klein bottle was first described in 1882 by the German mathematician Felix Klein. Other related non-orientable objects are the Möbius strip and the real projective plane. It was originally named the Kleinsche Fläche "Klein surface"; however, this was incorrectly interpreted as Kleinsche Flasche "Klein bottle", which ultimately, due to the dominance of the English language in science, led to the adoption of this term in the German language as well.^{[citation needed]}

Construction

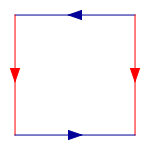
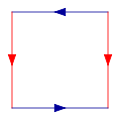
Start with a square, and then glue together corresponding colored edges, in the following diagram, so that the arrows match. More formally, the Klein bottle is the quotient space described as the square [0,1] × [0,1] with sides identified by the relations (0,y) ~ (1,y) for 0 ≤ y ≤ 1 and (x,0) ~ (1-x,1) for 0 ≤ x ≤ 1, :

This square is a fundamental polygon of the Klein bottle.

Note that this is an "abstract" gluing in the sense that trying to realize this in three dimensions results in a self-intersecting Klein bottle. The Klein bottle, proper, does not self-intersect. Nonetheless, there is a way to visualize the Klein bottle as being contained in four dimensions.

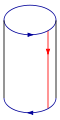
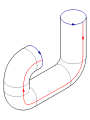
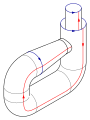
Glue the red arrows of the square together (left and right sides), resulting in a cylinder. To glue the ends together so that the arrows on the circles match, pass one end through the side of the cylinder. Note that this creates a circle of self-intersection. This is an immersion of the Klein bottle in three dimensions.

By adding a fourth dimension to the three dimensional space, the self-intersection can be eliminated. Gradually push a piece of the tube containing the intersection out of the original three dimensional space. A useful analogy is to consider a self-intersecting curve on the plane; self-intersections can be eliminated by lifting one strand off the plane.

This immersion is useful for visualizing many properties of the Klein bottle. For example, the Klein bottle has no boundary, where the surface stops abruptly, and it is non-orientable, as reflected in the one-sidedness of the immersion.

Properties

The Klein bottle can be seen as a fiber bundle as follows: one takes the square from above to be E, the total space, while the base space B is given by the unit interval in x, and the projection $\pi$ is given by $\pi (x,y)=x.$ Since the two endpoints of the unit interval in x are identified, the base space B is actually the circle $S^{1}$ , and so the Klein bottle is the twisted $S^{1}$ -bundle (circle bundle) over the circle.

Like the Möbius strip, the Klein bottle is a two-dimensional differentiable manifold which is not orientable. Unlike the Möbius strip, the Klein bottle is a closed manifold, meaning it is a compact manifold without boundary. While the Möbius strip can be embedded in three-dimensional Euclidean space R³, the Klein bottle cannot. It can be embedded in R⁴, however.

The Klein bottle can be constructed (in a mathematical sense, because it cannot be done without allowing the surface to intersect itself) by joining the edges of two Möbius strips together, as described in the following anonymous limerick:

A mathematician named Klein

Thought the Möbius band was divine.

Said he: "If you glue

The edges of two,

You'll get a weird bottle like mine."

It can also be constructed by folding a Möbius strip in half lengthwise and attaching the edge to itself.

Six colors suffice to color any map on the surface of a Klein bottle; this is the only exception to the Heawood conjecture, a generalization of the four color theorem, which would require seven.

A Klein bottle is equivalent to a sphere plus two cross caps.

Dissection

Dissecting the Klein bottle results in Möbius strips.

Dissecting a Klein bottle into halves along its plane of symmetry results in two mirror image Möbius strips, i.e. one with a left-handed half-twist and the other with a right-handed half-twist (one of these is pictured on the right). Remember that the intersection pictured isn't really there. In fact, it is also possible to cut the Klein bottle into a single Möbius strip.

Parametrization

The "figure 8" immersion of the Klein bottle has a particularly simple parametrization:

{\begin{array}{rcl}x&=&\left(r+\cos {\frac {u}{2}}\sin v-\sin {\frac {u}{2}}\sin 2v\right)\cos u\\y&=&\left(r+\cos {\frac {u}{2}}\sin v-\sin {\frac {u}{2}}\sin 2v\right)\sin u\\z&=&\sin {\frac {u}{2}}\sin v+\cos {\frac {u}{2}}\sin 2v\end{array}}

In this immersion, the self-intersection circle is a geometric circle in the XY plane. The positive constant $r$ is the radius of this circle. The parameter $u$ gives the angle in the XY plane, and $v$ specifies the position around the 8-shaped cross section.

The parametrization of the 3-dimensional immersion of the bottle itself is much more complex. Here is a simplified version:

{\begin{array}{rcl}x&=&{\frac {{\sqrt {2}}\left(20u^{3}-65\pi u^{2}+50\pi ^{2}u-16\pi ^{3}\right)\cos \left(v\right)\left(\cos \left(u\right)\left(3\cos ^{2}\left(u\right)-1\right)-2\cos \left(2u\right)\right)}{80\pi ^{3}{\sqrt {8\cos ^{2}\left(2u\right)-\cos \left(2u\right)\left(24\cos ^{3}\left(u\right)-8\cos \left(u\right)+15\right)+6\cos ^{4}\left(u\right)\left(1-3\sin ^{2}\left(u\right)\right)+17}}}}-{\frac {3\cos u}{4}}+{\frac {3}{4}}\\y&=&-{\frac {\left(20u^{3}-65\pi u^{2}+50\pi ^{2}u-16\pi ^{3}\right)\sin v}{60\pi ^{3}}}\\z&=&-{\frac {{\sqrt {2}}\left(20u^{3}-65\pi u^{2}+50\pi ^{2}u-16\pi ^{3}\right)\sin u\cos v}{15\pi ^{3}{\sqrt {8\cos ^{2}\left(2u\right)-\cos \left(2u\right)\left(24\cos ^{3}\left(u\right)-8\cos \left(u\right)+15\right)+6\cos ^{4}\left(u\right)\left(1-3\sin ^{2}u\right)+17}}}}+{\frac {\sin u\cos ^{2}u}{4}}-{\frac {\sin u\cos u}{2}}+{\frac {\sin u}{4}}\end{array}}

In this parametrization, $u$ follows the length of the bottle's body while $v$ goes around its circumference.

Generalizations

The generalization of the Klein bottle to higher genus is given in the article on the fundamental polygon.

Trivia

A mounted Klein bottle is the trophy for the BASIC WonderCup Challenge.
The TV series Futurama has a brand of beer, Klein's Beer, sold in a Klein bottle.
The British Science Museum has on display a beautiful collection of hand-blown glass Klein bottles, exhibiting many variations on the same topological theme. The bottles date from 1995 and were made for the museum by Alan Bennett. [1]
Russell Hoban's 2001 novel Amaryllis Night and Day makes extensive use of the Klein bottle as a metaphor. The display of bottles at London's Science Museum, and Alan Bennett himself, also feature in the book.
In the computer game Nethack, attempting to dip a potion into itself gives the message, "That is a potion bottle, not a Klein bottle!"
Joe Strummer and the Mescaleros have a song entitled "Mega Bottle Ride" which describes a journey "into the fourth dimension" via the "Banchoff-Klein Mega Bottle Ride".
In the Janine Melnitz, Ghostbuster episode of the TV show The Real Ghostbusters, Ray mentions adding another Klein bottle to the Containment Unit.
In the book Visitors From Oz, the characters construct a Klein bottle to travel from Oz to Earth.
In the Infocom game Trinity, a giant Klein bottle figures prominently, and is used to help solve one of the puzzles.
Clifford Stoll, author of The Cuckoo's Egg, manufactures Klein bottles and sells them via the Internet at Acme Klein Bottle.
The Magic: The Gathering card Elkin Bottle shows a 3D representation of a Klein bottle. The name "Elkin" is an obvious anagram of the word "Klein".
American computer scientist Ted Kaehler was visiting Germany and walked into a glass-blower's shop. He asked for "ein Klein Flasche." The glass-blower handed him a small bottle, as "klein" means "small". Kaehler eventually had to draw a Klein bottle on paper before the artisan understood what he was asking for; he was able to make one, although it was not a real Klein bottle.^{[citation needed]}
The unix fortune cookie program says, "Klein bottle for rent, enquire within."

References

Weisstein, Eric W. "Klein Bottle". MathWorld.

External links