Arccosecans and arccosecans

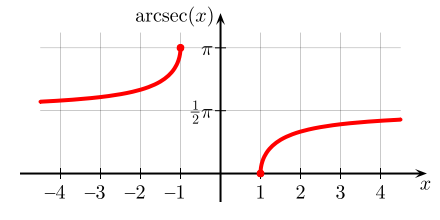
Arkussekans and Arkuskosekans are cyclometric functions. They are the inverse functions of the secant function or the coscan function and thus arc functions . Since the secant and cosecant functions are periodic, the reversal of the secant is limited to and the cosecant is limited to. The arccosecant is denoted by and the arccosecant by . The spellings and are used less often, but especially in English ; but they do not mean that or are the reciprocal values of and . ${\displaystyle \lbrack 0\,,\,\pi \rbrack }$ ${\displaystyle \lbrack -{\pi /2},\,\pi /2\rbrack }$ ${\displaystyle \operatorname {arcsec} \,(x)}$ ${\displaystyle \operatorname {arccsc} \,(x)}$ ${\displaystyle \sec ^{-1}(x)}$ ${\displaystyle \csc ^{-1}}$ ${\displaystyle \operatorname {arcsec} }$ ${\displaystyle \operatorname {arccsc} }$ ${\displaystyle \sec }$ ${\displaystyle \csc }$

properties

	Arc secans	Arccosecans
Function graph
Domain of definition	${\displaystyle -\infty <x\leq -1\,,\,1\leq x<+\infty }$	${\displaystyle -\infty <x\leq -1\,,\,1\leq x<+\infty }$
Range of values	${\displaystyle 0\leq f(x)\leq \pi }$	${\displaystyle -{\frac {\pi }{2}}\leq f(x)\leq {\frac {\pi }{2}}}$
monotony	Strictly increasing monotonously in both sections	Strictly decreasing monotonically in both sections
Symmetries	Point symmetry to the point ${\displaystyle x=0,y={\frac {\pi }{2}}}$	Odd function ${\displaystyle \operatorname {arccsc} \,(x)=-\operatorname {arccsc} \,(-x)}$
Asymptotes	${\displaystyle f(x)\to {\frac {\pi }{2}}}$ For ${\displaystyle x\to \pm \infty }$	${\displaystyle f(x)\to 0}$ For ${\displaystyle x\to \pm \infty }$
zeropoint	${\displaystyle x=1\!\,}$	no
Jump points	no	no
Poles	no	no
Extremes	Minimum at , maximum at ${\displaystyle \left(1|0\right)}$ ${\displaystyle \left(-1|\pi \right)}$	Minimum at , maximum at ${\displaystyle \left(-1|-{\frac {\pi }{2}}\right)}$ ${\displaystyle \left(1|{\frac {\pi }{2}}\right)}$
Turning points	no	no

Series developments

The series expansions of arcsecans and arcsecans are:

${\displaystyle \operatorname {arcsec}(x)={\frac {\pi }{2}}-\sum _{k=0}^{\infty }{\frac {(2k-1)!!x^{-(2k+1)}}{(2k)!!\cdot (2k+1)}}\approx {\frac {\pi }{2}}-x^{-1}-{\frac {1}{6}}x^{-3}-{\frac {3}{40}}x^{-5}}$

${\displaystyle \operatorname {arccsc}(x)=\sum _{k=0}^{\infty }{\frac {(2k-1)!!x^{-(2k+1)}}{(2k)!!\cdot (2k+1)}}={\frac {1}{x}}\;+\;{\frac {1}{2\cdot 3x^{3}}}\;+\;{\frac {3}{2\!\cdot \!4\cdot 5x^{5}}}\;+\;{\frac {3\!\cdot \!5}{2\!\cdot \!4\!\cdot \!6\cdot 7x^{7}}}\;+\;\ldots }$

Integral representations

The following integral representations exist for the arccosecans and arccosecans:

${\displaystyle \operatorname {arcsec}(x)=\int \limits _{1}^{x}{\frac {\mathrm {d} t}{t{\sqrt {t^{2}-1}}}}}$

${\displaystyle \operatorname {arccsc}(x)=\int \limits _{x}^{\infty }{\frac {\mathrm {d} t}{t{\sqrt {t^{2}-1}}}}}$

Derivatives

The derivatives are given by:

${\displaystyle {\frac {\mathrm {d} }{\mathrm {d} x}}\operatorname {arcsec} (x)={\frac {1}{|x|{\sqrt {x^{2}-1}}}}}$

${\displaystyle {\frac {\mathrm {d} }{\mathrm {d} x}}\operatorname {arccsc} (x)=-{\frac {1}{|x|{\sqrt {x^{2}-1}}}}}$

Integrals

${\displaystyle \int \operatorname {arcsec} (x)\,\mathrm {d} x=x\cdot \operatorname {arcsec} (x)-\operatorname {sgn}(x)\cdot \ln \left(\left|x+{\sqrt {x^{2}-1}}\right|\right)+C=x\cdot \operatorname {arcsec} (x)-\operatorname {arcosh} (|x|)+C}$

${\displaystyle \int \operatorname {arccsc} (x)\,\mathrm {d} x=x\cdot \operatorname {arccsc} (x)+\operatorname {sgn}(x)\cdot \ln \left(\left|x+{\sqrt {x^{2}-1}}\right|\right)+C=x\cdot \operatorname {arccsc} (x)+\operatorname {arcosh} (|x|)+C}$

Conversion and relationships with other cyclometric functions

${\displaystyle \operatorname {arcsec} \,(x)=\arccos \left({\frac {1}{x}}\right)}$

${\displaystyle \operatorname {arccsc} \,(x)=\arcsin \left({\frac {1}{x}}\right)}$

See also

• Trigonometry formula collection
• Trigonometric functions

Web links

• Eric W. Weisstein: Inverse Secant and Inverse Cosecant on MathWorld