Zariski tangent space

The Zariski tangent space is a concept from algebraic geometry , which translates the terms tangents , tangent planes and tangent spaces known from elementary geometry and differential geometry into the language of algebraic geometry.

In order to assign an affine subspace of the surrounding space to a point of a variety , the analytical methods of differential geometry are translated into an algebraic language. In the language of modern algebraic geometry, the tangent space of a schema is defined intrinsically, i.e. without reference to a surrounding space.

motivation

Analogy to differential geometry

Classically, the tangent space at a point is defined as a set of tangent vectors . These in turn clearly correspond to the directional derivatives on this point. Directional derivatives are exactly the derivatives (see the section below) of the smooth functions, which is why the tangent space can also be defined as the set of derivatives at a point.

Because derivations are linear and the derivation of a constant function results in zero, a derivation is uniquely determined by its application to the elements of the maximum ideal . Furthermore, every derivation disappears due to the Leibniz rule that applies to it . So you can understand derivatives as linear mappings . This motivates the definition below. ${\displaystyle {\mathfrak {m}}=\left\{f\colon f(x)=0\right\}}$ ${\displaystyle {\mathfrak {m}}^{2}}$ ${\displaystyle D:{\mathfrak {m}}/{\mathfrak {m}}^{2}\to k}$

(While this definition can also be transferred to the tangent space of manifolds , it has hardly any application there. Within algebraic geometry, the algebraic definition enables the use of ideal theory also in the investigation of tangent spaces, as well as the generalization of the term in the context of Schemes .)

Tangent space of an affine hypersurface

In the following, let be an algebraically closed field, the affine- dimensional space and an irreducible polynomial. be the hypersurface defined by ${\displaystyle k}$ ${\displaystyle \mathbb {A} _{k}^{n}}$ ${\displaystyle n}$ ${\displaystyle f\in k[X_{1},\dots ,X_{n}]}$ ${\displaystyle H}$ ${\displaystyle f}$

${\displaystyle H:=\{(x_{1},\dots ,x_{n})\in A_{k}^{n}|f(x_{1},\dots ,x_{n})=0\}}$

If a point is on the hypersurface, then a straight line is a tangent at the point if it has a multiple intersection with the point . Expressed algebraically, this means: ${\displaystyle P}$ ${\displaystyle H}$ ${\displaystyle P}$ ${\displaystyle H}$ ${\displaystyle P}$

Without loss of generality, let it be the zero point. (This can always be achieved after changing coordinates.) If there is any point, the straight line has ${\displaystyle P}$ ${\displaystyle (a_{1},\dots ,a_{n})\in \mathbb {A} _{k}^{n}}$

${\displaystyle g:=t\cdot (a_{1},\dots ,a_{n}),}$

which goes through the zero point and , exactly in the zeros of the polynomial : ${\displaystyle (a_{1},\dots ,a_{n})}$ ${\displaystyle p\in k[t]}$

${\displaystyle p:=f(t\cdot a_{1},\dots ,t\cdot a_{n})}$

Intersections with . ${\displaystyle H}$

The polynomial is of the form ${\displaystyle p}$

${\displaystyle p=\alpha _{0}+\alpha _{1}x^{1}+\cdots +\alpha _{k}x^{k}}$

Since zero is an intersection, is . If so, the straight line has a multiple point of intersection with at the zero point and is a tangent at . The union of all tangents is an affine subspace and is called the tangent space of . ${\displaystyle \alpha _{0}=0}$ ${\displaystyle \alpha _{1}=0}$ ${\displaystyle H}$ ${\displaystyle H}$ ${\displaystyle H}$

definition

Let be an algebraic variety (over a body ) with a coordinate ring , and let be a point with the associated maximum ideal ${\displaystyle V}$ ${\displaystyle k}$ ${\displaystyle k\left[V\right]}$ ${\displaystyle x\in V}$

${\displaystyle {\mathfrak {m}}_{x}=\left\{f\in k\left[V\right]\colon f(x)=0\right\}}$.

Then the cotangent space is defined as ${\displaystyle T_{x}^{*}V}$

${\displaystyle T_{x}^{*}V:={\mathfrak {m}}_{x}/{\mathfrak {m}}_{x}^{2}}$

and the Zariski tangent space as its dual space ${\displaystyle T_{x}V}$

${\displaystyle T_{x}V=\operatorname {Hom} ({\mathfrak {m}}_{x}/{\mathfrak {m}}_{x}^{2},k)}$.

More generally, for a local ring with a maximum ideal, the cotangent space can be defined as, and analogously the Zariski tangent space as its dual space . The Zariski tangent space of an algebraic variety in the point is then the Zariski tangent space of the local ring , i.e. the ring of the seeds of regular functions in . ${\displaystyle R}$ ${\displaystyle {\mathfrak {m}}}$ ${\displaystyle {\mathfrak {m}}/{\mathfrak {m}}^{2}}$ ${\displaystyle \operatorname {Hom} ({\mathfrak {m}}/{\mathfrak {m}}^{2},R/{\mathfrak {m}})}$ ${\displaystyle x}$ ${\displaystyle {\mathcal {O}}_{x}=k\left[V\right]_{{\mathfrak {m}}_{x}}}$ ${\displaystyle x}$

Explicit calculation

Let be an algebraic variety with a defining ideal and be . For be ${\displaystyle V\subset k^{n}}$ ${\displaystyle I(V)}$ ${\displaystyle x\in V}$ ${\displaystyle f\in I}$

${\displaystyle df_{x}:=\sum _{i=1}^{n}{\frac {\partial f}{\partial X_{i}}}(x)X_{i}}$.

Then the Zariski tangent space is isomorphic to , where that of the ${\displaystyle V(I_{x})}$ ${\displaystyle I_{x}\subset k\left[X_{1},\ldots ,X_{n}\right]}$

${\displaystyle df_{x},f\in I(V)}$

spanned ideal is. So

${\displaystyle T_{x}V=\left\{v\in k^{n}\colon df_{x}(v)=0\ \forall f\in I(V)\right\}}$.

Are producers of , then are producers of . ${\displaystyle f_{1},\ldots ,f_{r}}$ ${\displaystyle I(V)}$ ${\displaystyle df_{1},\ldots ,df_{r}}$ ${\displaystyle I_{x}}$

Examples

Graphs of the curves defined above y ² = x ³ - x and y ² = x ³ - x + 1 ${\displaystyle \mathbb {R} }$

• ${\displaystyle V(y^{2}-x^{3}+x)}$:

The tangent in an is the y-axis, so . The tangent space in is the same, i.e. H. the tangent space is not to be understood as an affine space, but as a vector space. In general, the tangent at the point is the core of the linear mapping , i.e. the 1-dimensional subspace of the spanned by the vector . ${\displaystyle (0,0)}$ ${\displaystyle V(y^{2}-x^{3}+x)}$ ${\displaystyle V(x)}$ ${\displaystyle (1,0)}$ ${\displaystyle (x,y)}$ ${\displaystyle (1-3x^{2},2y)}$ ${\displaystyle (2y,3x^{2}-1)}$ ${\displaystyle k^{2}}$

• ${\displaystyle V(y^{2}-x^{3}+x-1)}$:

Here, too, the tangent at the point is the core of the linear mapping , i.e. the 1-dimensional subspace of the spanned by the vector . ${\displaystyle (x,y)}$ ${\displaystyle (1-3x^{2},2y)}$ ${\displaystyle (2y,3x^{2}-1)}$ ${\displaystyle k^{2}}$

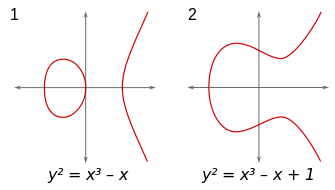
• ${\displaystyle V(y^{2}-x^{3}-x^{2})}$( Newtonian knot ):

Here you can create in two tangents, and . The tangential space is the one spanned by it . The dimension of the tangent space is larger than the dimension of the variety at this point, it is a singularity (see below). ${\displaystyle (0,0)}$ ${\displaystyle y=x}$ ${\displaystyle y=-x}$ ${\displaystyle k^{2}}$

• ${\displaystyle V=V(y^{2}-x^{3})}$( Neil's parable ):

As in the previous example, here is , so . ${\displaystyle df_{(0,0)}=0}$ ${\displaystyle T_{(0,0)}V=k^{2}}$

• ${\displaystyle V=V(x^{2}+y^{2}-z^{2})}$:

One calculates . In particular is a singularity. There are no further singularities on this surface. For example is . ${\displaystyle T_{(0,0,0)}V=k^{3}}$ ${\displaystyle (0,0,0)}$ ${\displaystyle T_{(1,0,1)}V=V(x-z)}$

Derivations

Equivalently, the tangent space can also be defined with the help of derivatives . (This corresponds to the interpretation of vector fields as direction derivatives .)

Let be an algebraic variety and the ring of its regular functions. A derivation of in one point is a -linear mapping with ${\displaystyle V}$ ${\displaystyle {\mathcal {O}}(V)}$ ${\displaystyle {\mathcal {O}}(V)}$ ${\displaystyle x\in V}$ ${\displaystyle k}$ ${\displaystyle \delta \colon {\mathcal {O}}(V)\to k}$

${\displaystyle \delta (fg)=\delta (f)g(x)+f(x)\delta (g)}$

for everyone . ${\displaystyle f,g\in {\mathcal {O}}(V)}$

The - vector space of the derivatives in is isomorphic to the Zariski tangent space . ${\displaystyle k}$ ${\displaystyle x}$ ${\displaystyle T_{x}V}$

Dimension and singularities

For a local Noetherian ring with a maximum ideal, the following always applies ${\displaystyle R}$ ${\displaystyle {\mathfrak {m}}}$

${\displaystyle \dim _{k}({\mathfrak {m}}/{\mathfrak {m}}^{2})\geq \dim(R)}$,

where denotes the Krull dimension of . ${\displaystyle \dim(R)}$ ${\displaystyle R}$

In particular, for all points of an algebraic variety : ${\displaystyle x}$ ${\displaystyle V}$

${\displaystyle \dim T_{x}V\geq \dim _{x}V}$.

Points in which is are called singularities . Points in which is are called regular points or smooth points . ${\displaystyle x\in V}$ ${\displaystyle \dim T_{x}V>\dim _{x}V}$ ${\displaystyle \dim T_{x}V=\dim _{x}V}$

The smooth spots form an open and dense subset of the variety . ${\displaystyle V}$

A smooth variety is an algebraic variety in which all points are smooth, so there are no singularities.

literature

• Oscar Zariski: The concept of a simple point of an abstract algebraic variety. Trans. Amer. Math. Soc. 62, 1-52 (1947)
• Pierre Samuel: Méthodes d'algèbre abstraite en géométrie algébrique. Results of mathematics and its border areas (NF), volume 4. Springer-Verlag, Berlin-Göttingen-Heidelberg, 1955.
• Igor Shafarevich: Basic algebraic geometry. Translated from the Russian by KA Hirsch. Revised printing of Grundlehren der Mathematischen Wissenschaften, Vol. 213, 1974. Springer Study Edition. Springer-Verlag, Berlin-New York, 1977 (Volume 1, Chapter II.1.2)
• Joe Harris: Algebraic geometry. A first course. Corrected reprint of the 1992 original. Graduate Texts in Mathematics, 133. Springer-Verlag, New York, 1995. ISBN 0-387-97716-3 (Chapter 14)

Web links

• Frank Herrlich: Local properties
• Basics of Algebraic Geometry
• VI Danilov: Zariski tangent space (Encyclopedia of Mathematics)