Parallelogram equation

The parallelogram equation (also parallelogram law or parallelogram identity ) is a mathematical theorem that has its origins in and its name from elementary geometry , but also applies in a very similar formulation to complex numbers and vectors in interior product spaces .

Application in geometry

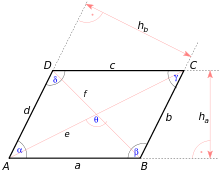
Designations on the parallelogram

sentence

In a parallelogram with side lengths a , b and diagonals e , f applies:

${\displaystyle 2\left(a^{2}+b^{2}\right)=e^{2}+f^{2}.}$

proofs

The theorem follows directly and in a particularly simple way from the Pythagorean theorem . To do this, we expand the adjacent drawing by the height on the left side at the diagonal f with the sections q . Applying the Pythagorean theorem twice gives the two equations ${\displaystyle h_{a}}$

${\displaystyle (a+q)^{2}+h_{a}^{2}=e^{2}}$

${\displaystyle (a-q)^{2}+h_{a}^{2}=f^{2}}$

The sum of these two equations gives . A third application delivers with which the theorem is proven. ${\displaystyle 2(a^{2}+q^{2}+h_{a}^{2})=e^{2}+f^{2}}$ ${\displaystyle q^{2}+h_{a}^{2}=b^{2}}$

The proof is very simple with the cosine law :

${\displaystyle {\begin{aligned}e^{2}+f^{2}&=(a^{2}+b^{2}-2ab\ \cos(\beta ))+(c^{2}+b^{2}-2cb\ \cos(\gamma ))\\&=2(a^{2}+b^{2})\end{aligned}}}$,

there and is. ${\displaystyle c=a}$ ${\displaystyle \cos(\gamma )=\cos(\pi -\beta )=-\cos(\beta )}$

Two vectors and create a parallelogram ${\displaystyle {\vec {a}}}$ ${\displaystyle {\vec {b}}}$

In linear algebra at school, the proof with vectors and scalar product is suitable :

With and applies ${\displaystyle \color {red}{\vec {e}}\color {black}={\vec {a}}+{\vec {b}}}$ ${\displaystyle \color {blue}{\vec {f}}\color {black}={\vec {a}}-{\vec {b}}}$

${\displaystyle \color {red}e^{2}\color {black}+\color {blue}f^{2}\color {black}=\color {red}a^{2}+2{\vec {a}}\cdot {\vec {b}}+b^{2}\color {black}+\color {blue}a^{2}-2{\vec {a}}\cdot {\vec {b}}+b^{2}\color {black}=2a^{2}+2b^{2}}$.

Generalization and inversion

The following applies to any plane square with the given designations:

${\displaystyle a^{2}+b^{2}+c^{2}+d^{2}=e^{2}+f^{2}+4x^{2},}$

where denotes the distance between the centers of the two diagonals. ${\displaystyle x}$

If the square is a parallelogram, the two diagonal centers coincide. Thus the parallelogram equation is a special case. ${\displaystyle x=0}$

Conversely, it follows: If the parallelogram equation holds, then . The two diagonals halve each other, the square is a parallelogram. ${\displaystyle x=0}$

Application for complex numbers

sentence

For two complex numbers z, w:

${\displaystyle 2\left(|z|^{2}+|w|^{2}\right)=|z+w|^{2}+|z-w|^{2}.}$

proof

The validity of the theorem is obvious if one interprets the numbers in the Gaussian plane of numbers , in which z and w then span a parallelogram with the diagonals z + w and zw. However, it can also be derived directly mathematically. Using for every complex number we have: ${\displaystyle \left|z\right|^{2}=z{\overline {z}}}$ ${\displaystyle z}$

${\displaystyle \left|z+w\right|^{2}+\left|z-w\right|^{2}=(z+w){\overline {(z+w)}}+(z-w){\overline {(z-w)}}}$

${\displaystyle =(z+w)({\overline {z}}+{\overline {w}})+(z-w)({\overline {z}}-{\overline {w}})}$

${\displaystyle =(z{\overline {z}}+w{\overline {z}}+z{\overline {w}}+w{\overline {w}})+(z{\overline {z}}-w{\overline {z}}-z{\overline {w}}+w{\overline {w}})}$

${\displaystyle =2z{\overline {z}}+2w{\overline {w}}}$

${\displaystyle =2\left|z\right|^{2}+2\left|w\right|^{2}}$

The equation in vector spaces

The consideration in Prähilberträumen represents the most abstract view course, the statements of the two previous sections with the now following theorem can be proved (the one with the means of. Analytic geometry , the other by the recycling of a two-dimensional - vector space under Definition a multiplication and a norm), but the respective proofs are certainly not superfluous with the means available. ${\displaystyle \mathbb {C} }$ ${\displaystyle \mathbb {R} }$

sentence

In prehilbert spaces , i.e. vector spaces in which a scalar product is defined (or in vector spaces with at least one positive semidefinite inner product) the following applies:

${\displaystyle \|x+y\|^{2}+\|x-y\|^{2}=2(\|x\|^{2}+\|y\|^{2})}$

where is the norm induced by the scalar product (positive semidefinite inner product) ( semi-norm ). ${\displaystyle \|x\|={\sqrt {\langle x,x\rangle }}}$

proof

For the proof one only needs the fact that an inner product of each inner product space is linear with respect to the addition for both arguments (see definition of inner product and sesquilinear form ). Then we get:

${\displaystyle \|x+y\|^{2}+\|x-y\|^{2}=\langle x+y,x+y\rangle +\langle x-y,x-y\rangle }$

${\displaystyle =\langle x,x+y\rangle +\langle y,x+y\rangle \ +\ \langle x,x-y\rangle -\langle y,x-y\rangle }$

${\displaystyle =\langle x,x\rangle +\langle x,y\rangle +\langle y,x\rangle +\langle y,y\rangle \ +\ \langle x,x\rangle -\langle x,y\rangle -\langle y,x\rangle +\langle y,y\rangle }$

${\displaystyle =2\langle x,x\rangle +2\langle y,y\rangle =2(\|x\|^{2}+\|y\|^{2})}$

reversal

The parallelogram equation does not apply in normalized vector spaces , the norm of which is not defined by a scalar product. It is namely the set of Jordan-von Neumann (after Pascual Jordan and John von Neumann ): Applies in a normalized vector space the parallelogram, there is a scalar product , which generates the norm, that is, for all applicable ${\displaystyle (V,\|{\cdot }\|)}$ ${\displaystyle \langle {\cdot },{\cdot }\rangle }$ ${\displaystyle x\in V}$

${\displaystyle \|x\|={\sqrt {\langle x,x\rangle }}.}$

This scalar product can be defined by a polarization formula, in the real case for example by

${\displaystyle \langle x,y\rangle ={\frac {1}{4}}\left({\|x+y\|^{2}-\|x-y\|^{2}}\right)}$

and in the complex case by

${\displaystyle \langle x,y\rangle ={\frac {1}{4}}\left(\|x+y\|^{2}-\|x-y\|^{2}\right)+{\frac {i}{4}}\left(\|x+iy\|^{2}-\|x-iy\|^{2}\right).}$

swell

• Dirk Werner : Functional Analysis . 6th, corrected edition, Springer-Verlag, Berlin 2007, ISBN 978-3-540-72533-6 , pages 203-204.

Web links

Wikibooks: Proof of the Parallelogram Equation  - Learning and Teaching Materials