The Stokes' current function (symbol , dimension L³ T −1 ) by George Gabriel Stokes is an analytical aid in fluid mechanics for solving the Euler equations in three-dimensional, axially symmetrical , stationary flows of incompressible, frictionless fluids . The Stokes current function is the application of the concept of the current function to axially symmetric flows, which then also have analogous properties. The velocity field, which is automatically divergence-free and the flow therefore maintains volume and is density-stable, results from the derivatives of Stokes' current function. As in the flat case, the contour lines of Stokes' current function represent streamlines that border flow tubes here because of the axial symmetry. As in the flat case, the volume flow between two streamlines - in the stream tube ring bordered by them - is the same everywhere.

definition
A density-stable and steady flow is considered with a location-dependent but not time-dependent because it is a stationary velocity field. In the case of axially symmetrical flow, the position vector can advantageously be parameterized with cylindrical or spherical coordinates .


Stokes' current function in cylindrical coordinates
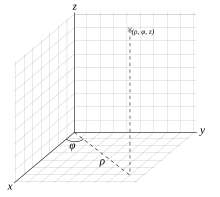
Parameterization of the room with cylindrical coordinates
The cylindrical coordinate system as shown in the picture is aligned so that the -direction is the direction about which the flow is axially symmetric. The distance of a point from the axis is given by the coordinate , which is denoted here with a large , in order to avoid confusion with the density . The angle counts in the circumferential direction perpendicular to the -axis. The speed must not depend on nor have any component in the tangential direction. The velocities in - and - direction result from the following derivatives of the current function :















The operator red forms the rotation , grad the gradient and the cross product .

Stokes' current function in spherical coordinates

Parameterization of the space with spherical coordinates
In spherical coordinates , the axis with is the direction about which the flow is axially symmetric. The distance of a point from the origin is given by the radius and the angle counts - as in cylindrical coordinates - in the circumferential direction perpendicular to the axis. Again, the speed must not depend on or have a component in the tangential direction. The velocities in - and - direction are then calculated using the following derivatives of the current function :












The relationship with the cylinder coordinates is given by and or and .




Properties of flows described by Stokes' current functions
Streamlines
The gradient of the current function in cylindrical coordinates is due to
![\ operatorname {grad} (\ psi) \ cdot \ vec {v} = \ operatorname {grad} (\ psi) \ cdot \ frac {1} {R} [\ operatorname {grad} (\ psi) \ times \ hat {e} _ \ varphi] = 0](https://wikimedia.org/api/rest_v1/media/math/render/svg/ace2e29dc914a0476952f0ea646922bcdfcdb66e)
perpendicular to the velocity and in spherical coordinates the same applies:
![\ operatorname {grad} (\ psi) \ cdot \ vec {v} = \ operatorname {grad} (\ psi) \ cdot \ frac {1} {r \ sin (\ theta)} [\ operatorname {grad} (\ psi) \ times \ hat {e} _ \ varphi] = 0](https://wikimedia.org/api/rest_v1/media/math/render/svg/b6fdd1b626476013aa2c26cd33677553d1e6595e)
By definition, the speed is everywhere tangential to the streamline, on which the value of the current function is constant. In the axial symmetry assumed here, the streamline represents a flow tube.
Density stability
If the velocity field of an axially symmetric flow is given by a Stoke's current function, then it applies in cylinder coordinates

as in spherical coordinates

because the divergence of fields of rotation is always zero. In a divergence-free flow, the substantial time derivative of the density disappears due to the mass balance , which is therefore constant over time.

A divergence-free flow contains neither sources nor sinks, so that under the given conditions streamlines in the interior of the liquid can neither begin nor end. The streamlines are either closed in a toroidal shape, are literally infinite, or end on the edge of the flow area.
Rotation of the flow
The rotation of the velocity field is the vortex strength, which is due to cylindrical coordinates
![\ operatorname {rot} \ vec {v} = \ omega \ hat {e} _ \ varphi = \ left (\ frac {\ partial v_R} {\ partial z} - \ frac {\ partial v_z} {\ partial R} \ right) \ hat {e} _ \ varphi = - \ left [\ frac {1} {R} \ frac {\ partial ^ 2 \ psi} {\ partial z ^ 2} + \ frac {\ partial} {\ partial R} \ left (\ frac {1} {R} \ frac {\ partial \ psi} {\ partial R} \ right) \ right] \ hat {e} _ \ varphi \ ,,](https://wikimedia.org/api/rest_v1/media/math/render/svg/22ad8e13895eaf7ecfb03e444b2aed2156a3d772)
has only one component ω in the tangential circumferential direction, which is why the vortex strength can be treated as a scalar field. In spherical coordinates it is also like this:
![\ operatorname {rot} \ vec {v} = \ omega \ hat {e} _ \ varphi = - \ frac {1} {r \ sin (\ theta)} \ left [\ frac {\ partial ^ 2 \ psi} {\ partial r ^ 2} + \ frac {\ sin (\ theta)} {r ^ 2} \ frac {\ partial} {\ partial \ theta} \ left (\ frac {1} {\ sin (\ theta) } \ frac {\ partial \ psi} {\ partial \ theta} \ right) \ right] \ hat {e} _ \ varphi \ ,.](https://wikimedia.org/api/rest_v1/media/math/render/svg/f565096269002f635bae790a07a16c1bd4f34284)
Unlike in plane flows, the Laplace operator is not on the right-hand side of the equals sign .
Volume flow between streamlines

The volume flow that occurs between two streamlines over the area A is independent of the location and the shape of the area
The volume flow between two streamlines is the same everywhere. This is shown using two streamlines, on which the current function assumes the values or , see picture. In order to calculate the volume flow that passes between these two streamlines, a line with the arc length and is defined, which therefore begins on one streamline and ends on the other streamline. The parameterization with the arc length has the effect that the length of the line and the tangent unit vector is equal to the derivative of the position vector. Due to the axial symmetry, this line defines an area on which the overflowing volume flow is to be determined. The volume flow which passes over this surface, is calculated with a line integral of the curve and the normal to



![s \ in [0, l] \ ,, \; \ psi (\ vec {x} (0)) = \ psi_0](https://wikimedia.org/api/rest_v1/media/math/render/svg/64eeaa0f869501c76e73290e05d9ad2c5bfce2dc)







Replacing gives the same result in spherical coordinates. Therefore, the same applies here as with the flow function in the plane: regardless of the special curve shape, the volume flow between two flow lines is the same everywhere. If the line starts and ends on the same streamline, the volume flow across it disappears. If the selected line is part of a streamline, then it will be seen that at no point in a streamline does fluid flow over it. Here too, a streamline acts like an impenetrable wall.

Determining equations for the current function
Not every flow function represents a physically realistic flow. In order for the current function to be in accordance with the laws of physics, it must obey Euler's equations, from which - as can be seen - the current function can be calculated independently of pressure. In a conservative gravitational field, the search for the current function is particularly easy. In contrast to the flat case, differential equations with variable coefficients result, which makes the solution more difficult.
Euler's equations
The Euler equations yield after formation of the rotation

Equations for the current function from the table:
| Coordinate system |
Determining equation
|
| Cylindrical coordinates
|

|
| Spherical coordinates
|

|
This is the component of the vortex strength, see above. These equations must satisfy the flow function so that they describe a physically realistic flow.


Conservative field of acceleration
In a conservative acceleration field - as is the force of gravity - the right-hand sides of the determining equations disappear because of the freedom of rotation of such fields. Then - as in the above case - it can be argued: the determining equation that occurred in the above proof as an intermediate result

will with

and any function f always satisfies:

In spherical coordinates, the same applies to the end result:
| Cylindrical coordinates
|

|
| Spherical coordinates
|

|
In particular is allowed.

boundary conditions
A flow field can only be stationary with solid walls. The boundary conditions are given along meridian curves, which are defined with and the arc length s. Then the tangent unit vector and the normal of the curve is in the radial direction . If fluid does not flow over the line anywhere, then it is part of a streamline and the line also represents a wall.



The Dirichlet boundary conditions specify the value of the current function along such a line and in cylindrical coordinates it follows:

which is why the radial velocity is defined perpendicular to lines with Dirichlet boundary conditions. If the value of the current function on the line is constant, then the line is part of a streamline and the normal component of the speed disappears along the line.
The Neumann boundary conditions specify the derivatives of the current function perpendicular to lines:

The speed component tangential to the line is specified by the Neumann boundary conditions. In spherical coordinates the same results with
example
In cylinder coordinates, the following applies in a plane flow and the velocity has only a radial component. With the above equation we then get:



So the second derivative of the Stokes current function vanishes according to the z-coordinate and the first derivative is therefore a constant . Then is the radial velocity


what is the velocity distribution of the plane source / sink.
A flow that only depends on the radius in spherical coordinates is the three-dimensional source / sink. With and results from the above determining equation



from which the velocity field of a three dimensional source / sink follows:

Here the speed decreases with the square of the distance to the source.
literature
























![\ operatorname {grad} (\ psi) \ cdot \ vec {v} = \ operatorname {grad} (\ psi) \ cdot \ frac {1} {R} [\ operatorname {grad} (\ psi) \ times \ hat {e} _ \ varphi] = 0](https://wikimedia.org/api/rest_v1/media/math/render/svg/ace2e29dc914a0476952f0ea646922bcdfcdb66e)
![\ operatorname {grad} (\ psi) \ cdot \ vec {v} = \ operatorname {grad} (\ psi) \ cdot \ frac {1} {r \ sin (\ theta)} [\ operatorname {grad} (\ psi) \ times \ hat {e} _ \ varphi] = 0](https://wikimedia.org/api/rest_v1/media/math/render/svg/b6fdd1b626476013aa2c26cd33677553d1e6595e)



![\ operatorname {rot} \ vec {v} = \ omega \ hat {e} _ \ varphi = \ left (\ frac {\ partial v_R} {\ partial z} - \ frac {\ partial v_z} {\ partial R} \ right) \ hat {e} _ \ varphi = - \ left [\ frac {1} {R} \ frac {\ partial ^ 2 \ psi} {\ partial z ^ 2} + \ frac {\ partial} {\ partial R} \ left (\ frac {1} {R} \ frac {\ partial \ psi} {\ partial R} \ right) \ right] \ hat {e} _ \ varphi \ ,,](https://wikimedia.org/api/rest_v1/media/math/render/svg/22ad8e13895eaf7ecfb03e444b2aed2156a3d772)
![\ operatorname {rot} \ vec {v} = \ omega \ hat {e} _ \ varphi = - \ frac {1} {r \ sin (\ theta)} \ left [\ frac {\ partial ^ 2 \ psi} {\ partial r ^ 2} + \ frac {\ sin (\ theta)} {r ^ 2} \ frac {\ partial} {\ partial \ theta} \ left (\ frac {1} {\ sin (\ theta) } \ frac {\ partial \ psi} {\ partial \ theta} \ right) \ right] \ hat {e} _ \ varphi \ ,.](https://wikimedia.org/api/rest_v1/media/math/render/svg/f565096269002f635bae790a07a16c1bd4f34284)




![s \ in [0, l] \ ,, \; \ psi (\ vec {x} (0)) = \ psi_0](https://wikimedia.org/api/rest_v1/media/math/render/svg/64eeaa0f869501c76e73290e05d9ad2c5bfce2dc)














![\ begin {array} {llclcl} \ text {cylinder coordinates:} & \ operatorname {rot} \ left (\ omega \ hat {e} _ \ varphi \ times \ frac {1} {R} [\ operatorname {grad} ( \ psi) \ times \ hat {e} _ \ varphi] \ right) & = & \ operatorname {rot} \ left (\ frac {\ omega} {R} \ operatorname {grad} (\ psi) \ right) & = & \ operatorname {red} \ vec k \\ \ text {spherical coordinates:} & \ operatorname {red} \ left (\ omega \ hat {e} _ \ varphi \ times \ frac {1} {r \ sin \ theta } [\ operatorname {grad} (\ psi) \ times \ hat {e} _ \ varphi] \ right) & = & \ operatorname {rot} \ left (\ frac {\ omega} {r \ sin \ theta} \ operatorname {grad} (\ psi) \ right) & = & \ operatorname {red} \ vec k \ ,, \ end {array}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3598b4bde97e518df26f697104320b03062ac160)

![\ begin {array} {llcl} \ text {cylindrical coordinates:} & \ operatorname {red} \ left (\ frac {\ omega} {R} \ operatorname {grad} (\ psi) \ right) & = & \ operatorname { grad} \ left (\ frac {\ omega} {R} \ right) \ times \ operatorname {grad} (\ psi) = \ left [\ frac {1} {R} \ frac {\ partial \ psi} {\ partial R} \ frac {\ partial \ omega} {\ partial z} - \ frac {\ partial \ psi} {\ partial z} \ frac {\ partial} {\ partial R} \ left (\ frac {\ omega} {R} \ right) \ right] \ hat {e} _ \ varphi = \ operatorname {red} \ vec k \\ \ text {spherical coordinates:} & \ operatorname {red} \ left (\ frac {\ omega} { r \ sin \ theta} \ operatorname {grad} (\ psi) \ right) & = & \ operatorname {grad} \ left (\ frac {\ omega} {r \ sin \ theta} \ right) \ times \ operatorname { grad} (\ psi) \\ && = & \ frac {1} {r} \ left [\ frac {\ partial \ psi} {\ partial \ theta} \ frac {\ partial} {\ partial r} \ left ( \ frac {\ omega} {r \ sin (\ theta)} \ right) - \ frac {\ partial \ psi} {\ partial r} \ frac {\ partial} {\ partial \ theta} \ left (\ frac { \ omega} {r \ sin (\ theta)} \ right) \ right] \ hat {e} _ \ varphi = \ operatorname {red} \ vec k \,. \ end {array}](https://wikimedia.org/api/rest_v1/media/math/render/svg/08a619e6df8d7efed22a7eb5cdef7085cfb3351e)

![\ operatorname {rot} (\ vec k) \ cdot \ hat {e} _ \ varphi = \ frac {1} {r} \ left [\ frac {\ partial} {\ partial r} (r k_ \ theta) - \ frac {\ partial k_r} {\ partial \ theta} \ right]](https://wikimedia.org/api/rest_v1/media/math/render/svg/8ae9a4f3b715dbbc60afb517a79de0fbcb363388)

















