Elliptical geometry

The real elliptical plane shown on the unit spherical surface in three-dimensional real space: The great circle is assigned to an elliptical point (pair of antipodes) as a polar , which is cut out of the sphere by the plane that is too perpendicular . The polar-polar assignment is fundamental in elliptical geometry. A representation on the sphere is possible for the elliptical planes, which are projective planes over a subfield of the real numbers and whose polarity as a square form is equivalent to the real, elliptical standard polarity. ${\displaystyle (A,A')}$ ${\displaystyle a}$ ${\displaystyle {\vec {AA'}}}$ ${\displaystyle M}$ ${\displaystyle S}$

An elliptical geometry is a non-Euclidean geometry in which, in the plane case , there is no straight line that is too parallel to a given straight line and a point that does not lie on the straight line . ${\displaystyle g}$ ${\displaystyle P}$ ${\displaystyle g}$ ${\displaystyle P}$

In elliptical geometry, certain axioms of absolute geometry apply , more on this later in this article . In addition, instead of the parallel postulate of Euclidean geometry, the axiom applies :

If there is a straight line and a point outside of this straight line, then there is no straight line in the plane through and that does not intersect. ${\displaystyle g}$ ${\displaystyle P}$ ${\displaystyle h}$ ${\displaystyle g}$ ${\displaystyle P}$ ${\displaystyle g}$

This means that there are no parallels in an elliptical geometry. Another alternative to the Euclidean axiom of parallels leads to hyperbolic geometry .

Properties of elliptical planes

Problem of the axioms of absolute geometry

There is no general consensus in the literature on how an absolute geometry should be characterized by axioms. The geometrical axioms mentioned in the introduction for an “absolute geometry”, which Felix Bachmann formulated, are quoted in full in Metric absolute geometry . They represent a certain minimum consensus if the absolute geometry is based on an orthogonality relation as being equal to the incidence relation. It is not trivial to compare these axioms with systems that do not include orthogonality as a basic relation.

Identification of the elliptical planes

A polar triangle is characteristic of the elliptical planes. All angles marked in red are right. The point is the pole of the straight line , this is the polar of . All straight lines cut through and are plumb bobs on . Every straight line that is too perpendicular goes through the point . ${\displaystyle C}$ ${\displaystyle c}$ ${\displaystyle C}$ ${\displaystyle C}$ ${\displaystyle c}$ ${\displaystyle c}$ ${\displaystyle c}$ ${\displaystyle C}$

On the basis mentioned, Bachmann characterizes elliptical geometry by the axiom

${\displaystyle \mathbf {P} }$There are three different straight lines that are orthogonal in pairs. ${\displaystyle a,b,c}$

In short: there is a polar triad . Under these axiomatic assumptions, he proves a statement that is stronger than the "elliptical parallel axiom" mentioned in the introduction:

“In the group level of an elliptical movement group, the projective incidence axioms apply. There is an elliptical polarity in it. "

The idiosyncratic formulation stems on the one hand from the fact that Bachmann uses a group-theoretical approach (straight lines are axis reflections and points are point reflections), in which "points" and "straight lines" have to be artificially differentiated for this statement, and on the other hand because he uses the term projective Level is more narrowly defined than it is nowadays: With Bachmann, a projective level is always a two-dimensional projective space above a body , the characteristic of which is not 2, i.e. a Pappus projective Fano level. In other words the sentence says:

1. As an incidence structure, every elliptical plane is isomorphic to a projective plane .
2. In addition to the incidence structure, there is an elliptical polarity (see correlation (projective geometry) for this term ). The orthogonal structure of the elliptical plane can be described as the elliptical polarity of the projective plane and vice versa.

An elliptical plane in the sense of the axiomatics mentioned at the beginning is always also a projective plane. Conversely, under certain necessary conditions, a projective plane can be turned into an elliptical plane:

1. The projective plane must be Pappus, because Pappus' theorem applies in the elliptical plane . In other words: The projective plane must be a two-dimensional projective space over a body.
2. The projective level has to fulfill the Fano axiom: Otherwise the whole group theoretic approach will not work that way. There are approaches to carry out similar investigations on geometries over bodies, the characteristic of which is 2. Bachmann (1973) has extensive literature references on this.
3. In addition, an elliptical projective polarity must be definable. This is not possible , for example

• in a finite pappus projective plane (see correlation (projective geometry) # polarities over finite spaces ),
• in planes over algebraically closed fields and
• somewhat more general in planes over bodies with only one square class .

A sufficient condition for the existence of (at least) an elliptical plane: Be a formal real body , then the symmetric bilinear form on the projective plane a projective polarity defined by this plane is an elliptical plane. The body doesn't have to be Archimedean . ${\displaystyle K}$ ${\displaystyle B(x,y)=x_{1}y_{1}+x_{2}y_{2}+x_{3}y_{3}}$ ${\displaystyle K^{3}}$ ${\displaystyle \mathbb {P} ^{2}(K)}$ ${\displaystyle K}$

Level models

The real elliptical plane and its representation on the sphere

In real elliptical geometry, the sum of the angles in the triangle is greater than 180 °, 2 rights or  . ${\displaystyle \pi }$

Above the body of the real numbers there is only one elliptical plane, apart from isomorphism: A well-known representation of this real model is provided by the spherical geometry , which can be understood as an illustration of the projective plane over the real numbers when one identifies opposite points. The additional, elliptical structure results from the elliptical polarity described here . ${\displaystyle \mathbb {R} }$

• The "plane" is a sphere,
• a "point" is a pair of two opposite points on the surface of the sphere, and
• a “straight line” is a circle on the surface of the sphere, the center of which is the center of the sphere (a great circle ).

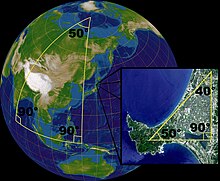
As a clear difference to Euclidean geometry, one can consider the sum of angles of triangles, which in this model is always greater than 180 ° - the fixed sum of angles of 180 ° in Euclidean geometry is equivalent to the parallel postulate. If you choose two straight lines through the North Pole, which form the angle with each other , they intersect the equator at an angle of 90 °. So the resulting triangle has an angle sum of 180 ° + . Compare with the picture on the right, there is . ${\displaystyle a}$ ${\displaystyle a}$ ${\displaystyle \mathbf {a} =50^{\circ }}$

First of all, the angles between great circles are “Euclidean” angles between the planes on which the great circles lie (or between associated normal vectors). In the real case, however, this does not cause any problems as long as only figures on the sphere are considered which are contained in the sphere without glued opposing poles in a "hemisphere without its edge".

The right faded "small" triangle on the map is to make clear that for small triangles on the sphere approximately or in the Euclidean plane (then distorted) map of a spherical section exactly the usual angle sum of 180 ° results, this second statement is true to.

The first statement that sufficiently small sections of a world map actually behave approximately Euclidean is also true. However, this can only be illustrated by a map if the triangle sides shown correspond to great circles on the sphere, which with the usual map projections can lead to a display as exactly straight lines at most for two of the three triangle sides (compare Mercator projection ) without this the angles at issue become distorted.

→ For area calculations and triangular congruence theorems for the real elliptical plane, see spherical triangle , whereby the limitation of lengths and angles explained in the next section must be observed.

Planes and straight lines in three-dimensional vector space

The projective plane over a subfield of the real numbers can be illustrated as a set of straight lines (as projective points) and planes (as straight lines) in the vector space . If the elliptical polarity is defined by (“real elliptical standard polarity ”), then a vector space line (ie a point of elliptical geometry) in the usual Euclidean sense is perpendicular to exactly one plane (a straight line of elliptical geometry). Every such pair (straight line, perpendicular plane) in vector space is a (pole, polar) pair in elliptical geometry. Two elliptical straight lines are perpendicular to each other if and only if their associated planes in vector space are perpendicular to each other in the Euclidean sense. As with the spherical representation described above, the Euclidean angle measure can also be transferred more generally for part bodies of . ${\displaystyle \mathbb {P} ^{2}(K)}$ ${\displaystyle K}$ ${\displaystyle K^{3}\subseteq \mathbb {R} ^{3}}$ ${\displaystyle B(x,y)=x_{1}y_{1}+x_{2}y_{2}+x_{3}y_{3}}$ ${\displaystyle \mathbb {R} }$

The angles and lengths of the spherical representation are angles of rotation between two-dimensional subspaces (elliptical angles) or between one-dimensional subspaces (elliptical path lengths) of the . This concept of length agrees with the length measurement of spherical geometry, if the unit sphere is used and only lengths and angles are considered that are less than or equal to 1 right angle (90 ° or  ), for larger angles between planes the secondary angle is to be used larger distances between points as well , since (angular) distances between straight lines through the origin are determined. It also applies, if you observe this restriction: ${\displaystyle K^{3}}$ ${\displaystyle {\frac {\pi }{2}}}$ ${\displaystyle \lambda }$ ${\displaystyle \lambda '=\pi -\lambda }$ ${\displaystyle \mathbb {R} ^{3}}$

The angle between two elliptical straight lines is equal to the distance between their poles, the distance between two elliptical points is equal to the angle between their polars.

This notion of angle and distance can also be transferred to elliptical planes over subfields of real numbers, provided that the symmetrical bilinear form , which defines the projective elliptical polarity , is equivalent to the “standard elliptical form” described in this section . Compare the following examples. ${\displaystyle (\mathbb {P} ^{2}(K),B)}$ ${\displaystyle B}$ ${\displaystyle \mathbb {P} ^{2}(K)}$ ${\displaystyle B(x,y)=x_{1}y_{1}+x_{2}y_{2}+x_{3}y_{3}}$

Examples of rational elliptical planes

The polar triangle described in the text in the "ordinary" rational elliptical plane. The “side” can be halved once, this gives the point . The light blue "sides" and in the triangle have no rational centers. ${\displaystyle AB}$ ${\displaystyle M}$ ${\displaystyle AM}$ ${\displaystyle MC}$ ${\displaystyle AMC}$

The "ordinary" polarity

If one specifically considers the field of the rational numbers with the real elliptic standard polarity , which is determined by the bilinear form , then this is an elliptic subplane of the real elliptic plane. Starting from the polar triangle , we form the midpoints of the route, compare the figure on the right. is a real and therefore also a rational center of the "route" . - There is a second center point on the elliptical straight line : It is the mirror point from the reflection on , but this does not have to be taken into account for the following. The “line” , or rather the ordered pair of points, has no rational center: the real center is not a rational straight line. So the route has no center. The example shows how in the case of a partial body (here ) a real proof of non-existence can be made, and that a line in an elliptical plane does not have to have a center. If one applies the perpendicular theorem of absolute geometry to the triangle , more precisely only the (Euclidean uninteresting) reasoning of existence: If two sides of a triangle have a perpendicular perpendicular to the center, then also the third, then it follows that at least one of the pairs or no center has either. Since, according to the analogous calculation as above , has a center, which has the same “length” as has no center. If you look at all of this on the Euclidean unit sphere, you can see that the starting points A, B, C (projective straight lines!) Intersect this sphere at two opposite rational points, but the projective point no longer. One must therefore argue carefully with the spherical model for subfields of the real number. ${\displaystyle K=\mathbb {Q} }$ ${\displaystyle B(x,y)=x_{1}y_{1}+x_{2}y_{2}+x_{3}y_{3}}$ ${\displaystyle A=<1,0,0>,B=<0,1,0>,C=<0,0,1>}$ ${\displaystyle M=M_{AC}=<1,1,0>}$ ${\displaystyle (A,B)}$ ${\displaystyle M'=<1,-1,0>\neq M}$ ${\displaystyle AB}$ ${\displaystyle M}$ ${\displaystyle B}$ ${\displaystyle (A,M)}$ ${\displaystyle N=\left(A+\left({\tfrac {1}{\sqrt {2}}},{\tfrac {1}{\sqrt {2}}}\right)\right)/2}$ ${\displaystyle (A,M)}$ ${\displaystyle \mathbb {Q} }$ ${\displaystyle AMC}$ ${\displaystyle (A,C)}$ ${\displaystyle (M,C)}$ ${\displaystyle (A,C)}$ ${\displaystyle (A,B)}$ ${\displaystyle (M,C)}$ ${\displaystyle {\frac {\pi }{2}}}$ ${\displaystyle (A,C)}$ ${\displaystyle M=<1,1,0>}$

An indefinite elliptical polarity

If one chooses a fixed positive whole number with , then the equation of form cannot be solved by a triplet of whole numbers without common divisors, because it can only be solved by three even numbers. Therefore, the bilinear shape determines an elliptical polarity. With this polarity, however, the rational elliptical plane cannot be embedded in the real elliptical plane, because the shape is hyperbolic ! ${\displaystyle k}$ ${\displaystyle k\equiv 3{\pmod {4}}}$ ${\displaystyle x_{1}^{2}-kx_{2}^{2}-kx_{3}^{3}=0}$ ${\displaystyle (x_{1},x_{2},x_{3})\in \mathbb {Z} ^{3}\setminus \{0\}}$ ${\displaystyle x_{1}^{2}-kx_{2}^{2}-kx_{3}^{2}\equiv 0{\pmod {4}}}$ ${\displaystyle B_{k}(x,y)=x_{1}y_{1}-kx_{2}y_{2}-kx_{3}y_{3}}$ ${\displaystyle \mathbb {R} }$ ${\displaystyle B_{k}}$

This means that you have at least two non-isomorphic elliptical planes over the same body . - In fact, infinitely many, because forms with only result in isomorphic rational elliptical geometries if it is true, and are therefore quadratically equivalent . ${\displaystyle K=\mathbb {Q} }$ ${\displaystyle B_{k},B_{l}}$ ${\displaystyle 0<k,l\equiv 3{\pmod {4}}}$ ${\displaystyle k=c^{2}l,\;c\in \mathbb {Q} ^{*}}$ ${\displaystyle k}$ ${\displaystyle l}$

Higher-dimensional elliptical spaces

Three- and higher-dimensional elliptical geometries are described axiomatically in the article Metric Absolute Geometry . They are always projective-elliptical spaces. That means: Above a body with a suitable elliptical polarity must be explainable by a zero-part, symmetrical bilinear form from rank to . A -dimensional projective-elliptical space can then be defined via . This construction is explained in the article Projective-Metric Geometry . ${\displaystyle K}$ ${\displaystyle \operatorname {char} (K)\neq 2}$ ${\displaystyle B}$ ${\displaystyle n+1}$ ${\displaystyle V=K^{n+1})}$ ${\displaystyle n}$ ${\displaystyle (\mathbb {P} ^{n}(K),B)}$ ${\displaystyle K}$

literature

• Friedrich Bachmann : Structure of geometry from the concept of reflection . 2nd supplemented edition. Springer, Berlin / Heidelberg / New York 1973, ISBN 3-540-06136-3 .
• Benno Klotzek: Euclidean and non-Euclidean elementary geometries . 1st edition. Harri Deutsch, Frankfurt am Main 2001, ISBN 3-8171-1583-0 .

Individual evidence

1. ↑ according to Klotzek (2001)
2. ↑ Bachmann (1973), p. 24
3. ↑ Bachmann (1973), p. 47. There the axiom is formulated according to group theory, this is an equivalent geometric translation.
4. ↑ Bachmann (1973), §16.1 sentence 7
5. ↑ Bachmann (1973), §16.2 The Pappus-Pascal Theorem
6. ↑ Bachmann (1973), Newer Literature pp. 358–365
7. ↑ In real elliptical geometry there is free mobility: Every figure made up of a straight line and a point on this straight line can be converted into any other figure of the same kind through reflections . Bachmann (1973), pages 124-125: Note on free mobility