Shear modulus
| material | Typical values for the shear modulus in G Pa (at room temperature) |
|---|---|
| steel | 79.3-81 |
| copper | 47 |
| titanium | 41.4 |
| Glass | 26.2 |
| aluminum | 25.5 |
| magnesium | 17th |
| Polyethylene | 0.117 |
| rubber | 0.0003 |
The shear modulus (also slip modulus , G-modulus , shear modulus or torsion modulus ) is a material constant that provides information about the linear-elastic deformation of a component as a result of a shear force or shear stress . The SI unit is Newton per square meter (1 N / m² = 1 Pa ), i.e. the unit of mechanical stress . In material databases , the shear modulus is usually given in N / mm² (= MPa) or kN / mm² (= GPa).
In the context of the theory of elasticity , the shear modulus corresponds to the second Lamé constant and bears the symbol there .
definition
The shear modulus describes the relationship between the shear stress and the tangent of the shear angle (slip):
A first approximation can be used for small angles ( small-angle approximation ).
This formula is analogous to Hooke's law for the 1-axis stress state :
When torsional stress of a component to its calculated torsional stiffness of the shear modulus and the torsional constant , which is related to the axis about which the body is twisted:
analogous to the determination of the tensile stiffness (from the product of the modulus of elasticity and cross-sectional area).
Relationship with other material constants
In an isotropic material the shear modulus is the elastic modulus E , the Poisson's ratio ν (Poisson's ratio) and the bulk modulus K in the following relationship:
For linear-elastic, non - auxetic material, the Poisson's number is greater than or equal to zero. The energy conservation results in the positive definiteness of the compression module and the modulus of elasticity . It follows that the Poisson's number is below 0.5. This results in the shear modulus of most materials in the linear-elastic range:
Auxetic materials are defined to have a negative Poisson's number, which only a few materials do. Since the shear modulus has a positive definite size due to the conservation of energy, the following applies to auxetic materials in the linear-elastic range:
Since the modulus of elasticity is also positive definite, the range of validity results for the Poisson's number
Conversion between the elastic constants
| The module ... | ... results from: | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Compression module | |||||||||||
| modulus of elasticity | |||||||||||
| 1. Lamé constant | |||||||||||
|
Shear modulus or (2nd Lamé constant)
|
|||||||||||
| Poisson's number | |||||||||||
| Longitudinal module | |||||||||||
See also
- Complex shear modulus
- Shear strength
- Thrust flow
- Continuum mechanics
- Strength theory
- Rheometer
- Moment magnitude scale
Web links
- Physics internship for beginners. (PDF; 146 kB) Uni Kiel.
Individual evidence
- ↑ Crandall, Dahl, Lardner: An Introduction to the Mechanics of Solids . McGraw-Hill, Boston 1959.
- ↑ Eurocode 3: Steel construction. Retrieved May 7, 2020 .
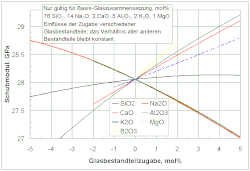
- ↑ Calculation of the shear modulus of glasses (English).
- ↑ G. Mavko, T. Mukerji, J. Dvorkin: The Rock Physics Handbook . Cambridge University Press, 2003, ISBN 0-521-54344-4 (paperback).