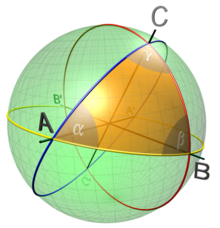
Spherical triangle

A spherical triangle or spherical triangle is in spherical geometry (spherical geometry) a part of a spherical surface that is delimited by three great arcs . The corners of the spherical triangle are the points at which two of these great circles intersect.

Similar to triangles in plane geometry, one speaks of the sides and angles of a triangle. However, the length of a side does not mean the length of the circular arc, but the associated central angle (central angle) . In radians , the value of this angle is exactly the length of the circular arc divided by the radius of the sphere:

{\ displaystyle {\ text {angle}} = {\ frac {\ text {circular arc}} {\ text {radius}}}}

To define lengths on a sphere, one first selects the scale so that the sphere is a unit sphere , and then first takes the length of the arc on this scale. One side, which corresponds to a quarter of the circumference of the sphere and great circle, has the length (i.e. 90 °). The interior angles (at the three corners) are defined by the tangents of the sides - i.e. the intersection angles between the planes in which the delimiting great circle arcs lie. ${\ displaystyle {\ tfrac {\ pi} {2}}}$

Euler's spherical triangles

Usually, the concept of the spherical triangle is restricted to Euler's spherical triangles (named after Leonhard Euler ), i.e. H. on spherical triangles in which all angles are smaller than or 180 ° and consequently all sides are smaller than (on the unit sphere:) . Without this restriction there would be several spherical triangles for any three points on the spherical surface, which are not all on a common great circle. This can be illustrated with the requirement for the shortest arc of the circle if one imagines that two points on a circle are farthest from each other when they are ( diametrically ) opposite one another , i.e. H. are 180 ° apart. If you go beyond 180 °, the bend is larger in one direction, but smaller than 180 ° in the other direction, which is why the latter can again be understood as the side of an Eulerian triangle. ${\ displaystyle \ pi}$ ${\ displaystyle r \ cdot \ pi}$ ${\ displaystyle \ pi}$

properties

Area

The area of a spherical triangle can be calculated from the angles and the triangle (in radians) and the spherical radius : ${\ displaystyle A_ {D}}$ ${\ displaystyle \ alpha, \ beta}$ ${\ displaystyle \ gamma}$ ${\ displaystyle r}$

{\ displaystyle A_ {D} = (\ alpha + \ beta + \ gamma - \ pi) \ cdot r ^ {2}.}

This relationship is derived as follows:

For area calculation on the spherical triangle

The three great circles determined by the corner points of a triangle ABC subdivide the spherical surface into eight triangles or four opposing triangle pairs. The triangle colored green in the figure forms a triangle with the opening angle with the yellow triangle ABC . The blue and red colored triangles form with the opposite triangle A'B'C 'two-triangles with the opening angles and . ${\ displaystyle \ beta}$ ${\ displaystyle \ alpha}$ ${\ displaystyle \ gamma}$

The following applies to the areas of the two-corners:

{\ displaystyle (I) \ quad A _ {\ alpha} = 2 \ alpha \ cdot r ^ {2}}

(Analogously for the two-corners with the opening angles and .) ${\ displaystyle \ beta}$ ${\ displaystyle \ gamma}$

The following applies to the areas of the blue, green and red triangles: ${\ displaystyle A_ {b}}$ ${\ displaystyle A_ {g}}$ ${\ displaystyle A_ {r}}$

{\ displaystyle A_ {b} = A _ {\ alpha} -A_ {D}}

{\ displaystyle A_ {g} = A _ {\ beta} -A_ {D}}

{\ displaystyle A_ {r} = A _ {\ gamma} -A_ {D}}

Together with the yellow counter-triangle A'B'C ', the blue, green and red triangles fill half of the surface of the sphere:

{\ displaystyle {\ frac {A_ {K}} {2}} = A_ {b} + A_ {g} + A_ {r} + A_ {D}}

If you put in, you get: ${\ displaystyle (I)}$

{\ displaystyle {\ frac {A_ {K}} {2}} = (A _ {\ alpha} -A_ {D}) + (A _ {\ beta} -A_ {D}) + (A _ {\ gamma} - A_ {D}) + A_ {D}}

{\ displaystyle = A _ {\ alpha} + A _ {\ beta} + A _ {\ gamma} -2A_ {D}}

With the equations for the calculation of the spherical surface and the spherical triangles one obtains:

{\ displaystyle {\ frac {4 \ pi r ^ {2}} {2}} = 2 \ alpha r ^ {2} +2 \ beta r ^ {2} +2 \ gamma r ^ {2} -2A_ { D}}

For thus results: ${\ displaystyle A_ {D}}$

{\ displaystyle A_ {D} = \ alpha r ^ {2} + \ beta r ^ {2} + \ gamma r ^ {2} - \ pi r ^ {2}}

{\ displaystyle = (\ alpha + \ beta + \ gamma - \ pi) \ cdot r ^ {2}}

Inside angle sum and spherical excess

On the unit sphere with radius 1, the following applies to the area:

{\ displaystyle A_ {D} = \ alpha + \ beta + \ gamma - \ pi}

The sum is called spherical excess (from the Latin excedere "to exceed") and indicates by how much the interior angle sum exceeds the value ( ). In contrast to the Euclidean triangle, the internal angle sum in the spherical triangle is not constant . The following applies to them (as a consequence of the formula for the area) in the general spherical triangle: ${\ displaystyle \ alpha + \ beta + \ gamma - \ pi}$ ${\ displaystyle \ pi}$ ${\ displaystyle = 180 ^ {\ circ}}$ ${\ displaystyle \ pi}$

{\ displaystyle \ pi <\ alpha + \ beta + \ gamma <5 \ pi}

in Euler's spherical triangle:

{\ displaystyle \ pi <\ alpha + \ beta + \ gamma <3 \ pi}

In a small spherical triangle ( "small" in comparison to the total surface of the sphere), the internal angle sum exceeds only slightly, since the triangle of the case of a plane of the inner angle sum rate approximates ( Verebnung ). The set of Legendre states, such as spherical triangles small size can be verebnet by reducing the angle. If, on the other hand, the triangle covers almost half of the spherical surface (3 angles too almost ), the sum of the angles is only slightly smaller than and the excess is therefore almost . ${\ displaystyle \ pi}$ ${\ displaystyle \ pi}$ ${\ displaystyle 3 \ pi}$ ${\ displaystyle 2 \ pi}$

Page total (on the unit ball)

In the general spherical triangle, the page total applies:

{\ displaystyle 0 <a + b + c <6 \ pi}

In Euler's triangle the following applies to the page total:

{\ displaystyle 0 <a + b + c <2 \ pi}

In general, a triangle is not uniquely determined by sww.

Congruence clauses

The sides a , b and c define two complementary triangles (colored blue and green).

There are two third sides to the given quantities a , b and γ .

On the sphere one has to distinguish between the congruence theorems of Euler's and non-Euler's triangles. For both it applies that similar triangles are already congruent (their area is already the same due to the proportionality to the spherical excess). The congruence theorem sww (side-angle-angle) valid in the Euclidean triangle, however, has no validity on the sphere (see figure). The congruence relationships in Euler's triangles are shown in the following table.

Overview of the congruence theorems in Euler's triangles
given triangle pieces	dual to it	Congruence class clearly determined?
sss	www	Yes
ssw	sww	No
sws	wsw	Yes

(for dualization, see the corresponding section in the article spherical geometry )
In non-Eulerian triangles, sss and sws do not yet determine a clear congruence class (see figures).

Sine law

The equations apply to spherical triangles

{\ displaystyle {\ frac {\ sin a} {\ sin \ alpha}} = {\ frac {\ sin b} {\ sin \ beta}} = {\ frac {\ sin c} {\ sin \ gamma}} }

Here are , and the sides ( arcs ) of the spherical triangle and , and the opposite angles on the spherical surface . ${\ displaystyle a}$ ${\ displaystyle b}$ ${\ displaystyle c}$ ${\ displaystyle \ alpha}$ ${\ displaystyle \ beta}$ ${\ displaystyle \ gamma}$

Cosine law

When spherical cosine law for spherical triangles the length of the sides of the triangle is in the angular indicate why instead of a trigonometric function whose six occur. The analogue to the plane sentence

{\ displaystyle c ^ {2} = a ^ {2} + b ^ {2} -2 \ cdot a \ cdot b \ cdot \ cos \ gamma}

is therefore

{\ displaystyle \ cos c = \ cos a \ cdot \ cos b + \ sin a \ cdot \ sin b \ cdot \ cos \ gamma}

,

Note that the sign is reversed. This side cosine law (here for c, analogously for sides a and b) is opposed to the angle cosine law :

{\ displaystyle \ cos \ gamma = - \ cos \ alpha \ cdot \ cos \ beta + \ sin \ alpha \ cdot \ sin \ beta \ cdot \ cos c}

,

where the first sign is negative.

literature

Isaac Todhunter: Spherical Trigonometry: For the Use of Colleges and Schools. Macmillan & Co., 1863, full text (Google Books)

Web links

Eric W. Weisstein : Spherical Triangle . In: MathWorld (English).
Surface of a spherical triangle on PlanetMath (English)

Individual evidence

↑ See definition of the spherical triangle in Guido Walz (Hrsg.): Lexikon der Mathematik . tape 4 . Springer-Verlag GmbH Germany, 2017, ISBN 978-3-662-53499-1 .

[1] See definition of the spherical triangle in Guido Walz (Hrsg.): Lexikon der Mathematik . tape 4 . Springer-Verlag GmbH Germany, 2017, ISBN 978-3-662-53499-1 .