Fokker-Planck equation

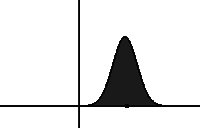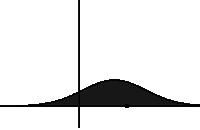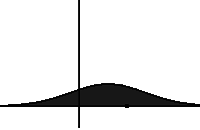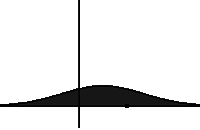
Solution of the 1D Fokker-Planck equation with drift and diffusion terms. The initial condition is a delta function at and the distribution drifts to the left. ${\displaystyle x=1}$

The Fokker-Planck equation (FPG, after Adriaan Daniël Fokker (1887–1972) and Max Planck (1858–1947)) is a partial differential equation . It describes the development of a probability density function over time under the effect of drift and diffusion . In its one-dimensional form, the equation is: ${\displaystyle P(x)}$ ${\displaystyle A(x,t)}$ ${\displaystyle B(x,t)}$

${\displaystyle {\frac {\partial }{\partial t}}P(x,t)=-{\frac {\partial }{\partial x}}{\Big [}A(x,t)\,P(x,t){\Big ]}+{\frac {1}{2}}{\frac {\partial ^{2}}{\partial x^{2}}}{\Big [}B(x,t)\,P(x,t){\Big ]}}$

In probability theory , this equation is also known as the Kolmogorov forward equation and, in this case, named after the mathematician Andrei Nikolayevich Kolmogorov . It is a linear parabolic partial differential equation that can only be solved analytically exactly for a few special cases (simple body geometry ; linearity of the boundary conditions , the drift and diffusion coefficients) .

For vanishing drift and constant diffusion , the FPG changes into the diffusion (or heat conduction) equation. ${\displaystyle A(x,t)=0}$ ${\displaystyle B(x,t)=B}$

In dimensions this is the Fokker-Planck equation ${\displaystyle D}$

${\displaystyle {\frac {\partial }{\partial t}}P(\mathbf {x} ,t)=-\sum _{i=1}^{D}{\frac {\partial }{\partial x_{i}}}{\Big [}A_{i}(x_{1},\ldots ,x_{D})P(\mathbf {x} ,t){\Big ]}+{\frac {1}{2}}\sum _{i=1}^{D}\sum _{j=1}^{D}{\frac {\partial ^{2}}{\partial x_{i}\,\partial x_{j}}}{\Big [}B_{ij}(x_{1},\ldots ,x_{D})P(\mathbf {x} ,t){\Big ]}}$

The Smoluchowski equation is used when describing the positions of the particles in the system. ${\displaystyle x}$

For Markovian processes , the FPG emerges from the Kramers-Moyal expansion , which is terminated after the second order.

Of great importance is the equivalent description of problems using Langevin equations , which, compared to the FPG, describe the microscopic dynamics of stochastic systems and - in contrast to the FPG - are generally non-linear .

Derivation

The FPG can be derived from the continuous Chapman-Kolmogorow equation , a more general equation for the time evolution of probabilities in Markov processes , if is a continuous variable and the jumps in are small. In this case a Taylor expansion (in this case it is also called a Kramers-Moyal expansion) is the Chapman-Kolmogorow equation ${\displaystyle x}$ ${\displaystyle x}$

${\displaystyle P(x,t)=\int {P\left(x-\Delta x,t-\Delta t\right)\Psi \left(x-\Delta x,\Delta x\right)d^{D}\!\left(\Delta x\right)}}$

possible and results in the FPG. Here is the probability that a state changes from to state . You can also start the development directly from the master equation , then the Taylor expansion according to time is no longer necessary. ${\displaystyle \Psi \left(x-\Delta x,\Delta x\right)}$ ${\displaystyle \left(x-\Delta x\right)}$ ${\displaystyle x}$

Assuming that the transition probability is small for large distances (only small jumps take place) one can use the following Taylor expansion (using the summation convention ): ${\displaystyle \Psi }$ ${\displaystyle \Delta x}$

${\displaystyle {\begin{aligned}P(x,t)\approx \int P(x,t)\Psi \left(x,\Delta x\right)-\Delta t\,\Psi \left(x,\Delta x\right){\frac {\partial P(x,t)}{\partial t}}&-\Delta x_{i}\,{\frac {\partial }{\partial x_{i}}}P(x,t)\Psi \left(x,\Delta x\right)\\&+{\frac {1}{2}}\Delta x_{i}\Delta x_{j}\,{\frac {\partial ^{2}}{\partial x_{i}\partial x_{j}}}P(x,t)\Psi \left(x,\Delta x\right)\;\,d^{D}\!\left(\Delta x\right)\end{aligned}}}$

By performing the integration (since it does not depend on it can be extracted from the integrals) one then obtains ${\displaystyle P}$ ${\displaystyle \Delta x}$

${\displaystyle {\frac {\partial P}{\partial t}}=-{\frac {\partial }{\partial x_{i}}}\left\langle \Delta x_{i}\right\rangle P+{\frac {1}{2}}{\frac {\partial ^{2}}{\partial x_{i}\partial x_{j}}}\left\langle \Delta x_{i}\Delta x_{j}\right\rangle P}$

With

${\displaystyle A_{i}=\left\langle \Delta x_{i}\right\rangle ={\frac {1}{\Delta t}}\int \Delta x_{i}\Psi \,d^{D}\Delta x}$

${\displaystyle B_{ij}=\left\langle \Delta x_{i}\Delta x_{j}\right\rangle ={\frac {1}{\Delta t}}\int {\Delta x_{i}\Delta x_{j}\Psi \,d^{D}\Delta x}}$

Stationary solution

The stationary solution of the one-dimensional FPG, i.e. H. for all , is given by ${\displaystyle P_{s}(x,t)}$ ${\displaystyle {\frac {\partial }{\partial t}}P_{s}(x,t)=0}$ ${\displaystyle t}$

${\displaystyle P_{s}(x,t)=P_{s}(x)={\frac {n}{B(x)}}\exp \left(2\int _{x_{0}}^{x}{\frac {A(x')}{B(x')}}dx'\right),}$

where the normalization constant can be determined using the condition . It should be noted that the integral for the lower edge disappears. ${\displaystyle n}$ ${\displaystyle \int _{-\infty }^{\infty }P_{s}(x)dx=1}$ ${\displaystyle x_{0}}$

In the case of higher dimensions, a stationary solution can generally no longer be found; here one is dependent on various approximation methods .

Relationship with stochastic differential equations

Be for the features and . Then the stochastic differential equation for the Ito process (in the Ito interpretation ) is given by ${\displaystyle \mathbf {U} \colon \mathbb {R} ^{n}\times \mathbb {R} _{+}\to \mathbb {R} ^{n}}$ ${\displaystyle \mathbb {V} \colon \mathbb {R} ^{n}\times \mathbb {R} _{+}\to \mathbb {R} ^{n\times m}}$ ${\displaystyle \{\mathbf {X} _{t}\}_{t\in \mathbb {R} _{+}}}$

${\displaystyle d\mathbf {X_{t}} =\mathbf {U} (\mathbf {X_{t}} ,t)dt+\mathbb {V} (\mathbf {X_{t}} ,t)d\mathbf {W_{t}} }$,

where denotes a -dimensional Wiener process ( Brownian motion ). Then the probability density function of the random variable fulfills an FPG in which drift or diffusion coefficients are given by and . ${\displaystyle (\mathbf {W_{t}} )}$ ${\displaystyle m}$ ${\displaystyle P(\mathbf {X_{t}} =\mathbf {x} ,t)=:P(\mathbf {x} ,t)}$ ${\displaystyle \mathbf {X} _{t}}$ ${\displaystyle \mathbf {A} =\mathbf {U} }$ ${\displaystyle \mathbb {B} =(B_{ij})=\mathbb {V} \mathbb {V} ^{T}}$

Fokker-Planck equation and path integral

Every Fokker-Planck equation is equivalent to a path integral . This follows e.g. B. from the fact that the general Fokker-Planck equation for variables ${\displaystyle D}$ ${\displaystyle \mathbf {q} =\{q_{i}\}}$

${\displaystyle {\begin{aligned}{\frac {\partial }{\partial t}}P(\mathbf {q} ,t)&=F\left({\frac {\partial }{\partial \mathbf {q} }},\mathbf {q} ,t\right)P(\mathbf {q} ,t),\\F\left({\frac {\partial }{\partial \mathbf {q} }},\mathbf {q} ,t\right)&=-\sum _{i=1}^{D}{\frac {\partial }{\partial q_{i}}}A_{i}(\mathbf {q} )+{\frac {1}{2}}\sum _{i=1}^{D}\sum _{j=1}^{D}{\frac {\partial ^{2}}{\partial q_{i}\,\partial q_{j}}}B_{ij}(\mathbf {q} ),\end{aligned}}}$

has the same structure as the Schrödinger equation . The Fokker-Planck operator corresponds to the Hamilton operator, the probability density function corresponds to the wave function . The path integral equivalent to the Fokker-Planck equation reads accordingly (see path integral ) ${\displaystyle F}$ ${\displaystyle P}$

${\displaystyle Z=N\int _{-\infty }^{\infty }{\mathcal {D}}\mathbf {q} \int _{-i\infty }^{i\infty }{\mathcal {D}}\mathbf {\tilde {q}} e^{\int Ldt},\;\;L=F\left(-\mathbf {\tilde {q}} ,\mathbf {q} ,t\right)-\mathbf {\tilde {q}} \cdot {\frac {\partial }{\partial t}}\mathbf {q} ,}$

where is a constant normalization factor. Path integrals of this type are the starting point for the perturbation calculation and renormalization group in the critical dynamics. The variables are z. B. for the Fourier components of the order parameter. The variables are called response variables . The Lagrange function only contains the response variables in square form. In contrast to quantum mechanics, however, it is not expedient here to carry out the integrations. ${\displaystyle N}$ ${\displaystyle \mathbf {q} }$ ${\displaystyle \mathbf {\tilde {q}} }$ ${\displaystyle L}$ ${\displaystyle \mathbf {\tilde {q}} }$

Fokker-Planck equation in plasma physics

The Fokker-Planck equation is particularly important in plasma physics because the impact term of the Boltzmann equation for plasmas can be written as a Fokker-Planck term. The reason for this is that the movement of the particles in the plasma is dominated by the many collisions with distant partners, which only cause small changes in speed (drift, diffusion); On the other hand, strong collisions with nearby particles are comparatively rare and therefore often negligible.

The equation is also known as the Landau equation , since it was first established by Lew Dawidowitsch Landau , but not in its Fokker-Planck form, which is described below.

In Landau equation gives single particle - distribution density in velocity space for particles of the type , the number of particles at a certain speed it is. In a plasma on which no external forces act, the change in distribution density due to collisions with particles of the type can be approximately described by the equation: ${\displaystyle \alpha }$ ${\displaystyle f_{\alpha }({\vec {v}},t)}$ ${\displaystyle v}$ ${\displaystyle \beta }$

${\displaystyle {\frac {\partial f_{\alpha }}{\partial t}}=-{\frac {\partial }{\partial v_{i}}}\left\langle \Delta v_{i}\right\rangle ^{\alpha \beta }f_{\alpha }+{\frac {1}{2}}{\frac {\partial ^{2}}{\partial v_{i}\partial v_{j}}}\left\langle \Delta v_{i}\Delta v_{j}\right\rangle ^{\alpha \beta }f_{\alpha }}$

With

${\displaystyle \left\langle \Delta v_{i}\right\rangle ^{\alpha \beta }=\left(1+{\frac {m_{\alpha }}{m_{\beta }}}\right)\Lambda _{c}\left({\frac {4\pi q_{\alpha }q_{\beta }}{m_{\alpha }}}\right)^{2}{\frac {\partial }{\partial v_{i}}}\left({\frac {1}{4\pi }}\int {\frac {f_{\beta }(v')}{\left|v-v'\right|}}dv'\right)}$

and

${\displaystyle \left\langle \Delta v_{i}\Delta v_{j}\right\rangle ^{\alpha \beta }=\Lambda _{c}\left({\frac {4\pi q_{\alpha }q_{\beta }}{m_{\alpha }}}\right)^{2}{\frac {\partial ^{2}}{\partial v_{i}\partial v_{j}}}\left({\frac {1}{4\pi }}\int f_{\beta }(v')\left|v-v'\right|dv'\right)}$

It is

• ${\displaystyle \Lambda _{c}}$the Coulomb logarithm : the larger its value, the stronger the dominance of many light collisions, and the better the validity of the Landau-Fokker-Planck equation
• ${\displaystyle q_{\alpha }}$and the electrical charges of the types of particles ${\displaystyle q_{\beta }}$
• ${\displaystyle m}$ their mass.

Since the particles in the plasma also collide with particles of the same species, the equation is usually non-linear.

This equation gets the particle number , momentum and energy . It also satisfies the H-theorem , i. H. Impacts lead to a Maxwell-Boltzmann velocity distribution .

See also

• Convection-diffusion equation

Web links

• Overview of stochastic processes
• Lecture notes: Stochastic description of physical systems Prof. Kree (pdf; 442 kB)

literature

• Crispin Gardiner: Stochastic Methods. A Handbook for the Natural and Social Sciences. 4th edition. Springer, Berlin et al. 2009, ISBN 978-3-540-70712-7 ( Springer series in synergetics = Springer complexity ).
• Hartmut Haug: Statistical Physics. Equilibrium Theory and Kinetics. 2nd revised and expanded edition. Springer, Berlin et al. 2006, ISBN 3-540-25629-6 ( Springer textbook ).
• Linda E. Reichl: A Modern Course in Statistical Physics. University of Texas Press. 1980, ISBN 0-7131-3517-4
• Hannes Risken: The Fokker-Planck Equation. Methods of Solutions and Applications. 2nd edition., 3rd printing, study edition. Springer, Berlin et al. 1996, ISBN 3-540-61530-X , ( Springer Series in Synergetics 18).
• Arthur G. Peeters, Dafni Strintzi: The Fokker-Planck equation, and its application in plasma physics. Ann. Phys. 17, No 2-3, 124 (2008). doi: 10.1002 / andp.200710279 .
• K.-H. Spatschek: Theoretical Plasma Physics. An introduction. Teubner, Stuttgart 1990, ISBN 3-519-03041-1 .

Individual evidence

1. ↑ ^{a b} H. K. Janssen: Lagrangean for Classical Field Dynamics and Renormalization Group Calculations of Dynamical Critical Properties . In: Z. Phys. B . 23, 1976, p. 377.