Johnson-Mehl-Avrami-Kolmogorow equation

The Johnson-Mehl-Avrami-Kolmogorow equation (short: JMAK equation , also Avrami equation ) describes the process of a phase or microstructure transformation at constant temperature ( isothermal change of state ). The equation gives an approximate rate of crystallization . The JMAK equation describes the entire process of the conversion with two quantities, the nucleation rate and the speed of growth of already formed areas of the new phase .

Historical

In 1937 the Russian mathematician Andrei N. Kolmogorov published a paper on the statistical theory of the crystallization of metals. Robert Franklin Mehl (1898–1976), head of the "Metallurgical Engineering" department at the Carnegie Institute of Technology since 1935 , and his doctoral student William Austin Johnson (* 1913, Bachelor 1933, MS 1935) showed their work on the kinetics of nucleation and growth in February 1939 at a conference. They published their results in the summer of 1939. The meeting was also attended by the metallurgist Melvin Avrami from the "School of Mines" at Columbia University in New York. Avrami then published a series of three seminal publications on the subject between 1939 and 1941.

Basics

Growth of a phase β in phase α: nuclei arise at nucleation centers N. The upper picture shows an earlier state, the lower a later one.

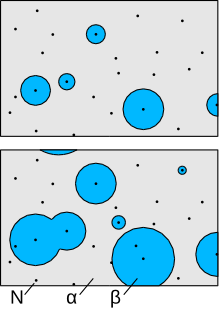
The transformation of one phase into another, for example the crystallization of an amorphous solid , does not happen everywhere at the same time, but begins at a few points ( nucleation ). The new phase (e.g. the crystallites ) grows from these points . At the same time, nucleation occurs again and again in other places; These areas of the new phase will then also continue to grow. This happens until all areas of the new phase are finally united and the old phase has completely disappeared. The JMAK equation indicates how large the proportion of the new phase in the overall system is as a function of time.

The prerequisite for the behavior described here is a system that first consists of one phase (here α), although another phase (β) is thermodynamically more stable. This occurs, for example, when the solubility of an element drops so much when an alloy cools that the alloy is oversaturated , i.e. when more of this element is in the solid than can remain in solution .

The JMAK equation is an important basis for the creation of time-temperature-conversion (TTT) diagrams .

Applications

The Johnson-Mehl-Avrami-Kolmogorow equation describes numerous processes in materials science , especially metallurgy , and in physical chemistry :

• Crystallization in an amorphous solid (e.g. polymer ).
• Phase changes with temperature, e.g. B. if above a limit temperature one, below another crystal structure is thermodynamically stable.
• In alloys during cooling: Formation of precipitates ( precipitates ) of poorly soluble elements or of crystallites with intermetallic phases that contain one or more poorly soluble elements (here, however, only the beginning of the process is described because the entire solid is not completely transformed comes).
• Chemical reactions with a reaction front when the reaction takes place close to thermodynamic equilibrium .

In many cases, the JMAK equation describes the beginning of the conversion well, while deviations from the JMAK behavior can occur towards the end of the conversion. In the case of the formation of crystals, this can be related, for example, to the fact that differently oriented crystals collide and that energetically unfavorable interfaces arise between them .

Mathematical treatment

The excretion of a phase β from the metastable phase α is considered.
Under the assumptions

• spherical germs
• a random distribution of the germs in the volume
• a constant nucleation rate  N , with which new nuclei are formed,
• a constant growth rate  v of the germs

the proportion f (t) of the transformed structure with time t results as:

${\displaystyle f(t)=1-e^{-{\frac {\pi }{3}}N\cdot v^{3}\cdot t^{4}}.}$

This equation applies to short and long transformation times  t as well as to small and large transformation components  f :

• For short times when the particles still grow independently of each other and where the following applies, the JMAK equation can be simplified to: ${\displaystyle f\ll 1}$

${\displaystyle f(t)={\frac {\pi }{3}}N\cdot v^{3}\cdot t^{4}.}$

In doing so, use is made of the rule that applies to: The equation for short times can be simplified as follows: the number of nuclei increases accordingly and the radius of each individual nucleus increases linearly with its volume, so the total volume of all nuclei increases at the beginning ${\displaystyle z\ll 1}$ ${\displaystyle 1-e^{-z}\approx z.}$
${\displaystyle N\cdot t}$ ${\displaystyle v\cdot t,}$ ${\displaystyle (v\cdot t)^{3}.}$ ${\displaystyle t^{4}.}$

• For long periods of time when the growing particles collide or their diffusion catchment areas overlap, the volume of the converted area increases more slowly than with and the proportion  f tends towards one: ${\displaystyle t\to \infty ,}$ ${\displaystyle t^{4},}$ ${\displaystyle f\to 1.}$

With the initial assumptions about seed forms and their growth, both equations are special cases of a more general law that also applies to many other models:

${\displaystyle f=1-e^{-k\cdot t^{n}}.}$

The Avrami exponent  n is between 1 and 4. For example, an exponent of n = 3 is obtained in two dimensions (crystallization in a very thin layer and disc-shaped nuclei) .

The constant  k depends on the nucleation rate  N and the growth rate  v . Since these depend on the temperature, k is also dependent on the temperature:

${\displaystyle k=k(N(T),v(T))=k(T).}$

Individual evidence

1. ↑ Michael C. Weinberg, Dunbar P. Birnie III, Vitaly A. Shneidman: Crystallization kinetics and the JMAK equation . In: Journal of Non-Crystalline Solids . tape 219 , October 1997, p. 89-99 , doi : 10.1016 / s0022-3093 (97) 00261-5 ( elsevier.com ).
2. ↑ M. Fanfoni, M. Tomellini: The Johnson-Mehl-Avrami-Kohnogorov model: A brief review . In: Nuovo Cimento D . tape 20 , no. 7-8 , July 1998, ISSN  0392-6737 , p. 1171-1182 , doi : 10.1007 / bf03185527 .
3. ↑ Original work: Andrei Nikolaevich Kolmogorov (КОЛМОГОРОВ): On the statistics of crystallization processes in metals / СТАТИСТИЧЕСКОЙ ТЕОРИИ КРИСТАЛЛИИЗАЦИИ МЕТАЛЛОВ . In: Izvestiya Akademii Nauk SSSR Seriya Mathemeticheskaya . [Bull. Acad. Sci. USSR Seria Mathematica, ИЗВЕСТИЯ АКАДЕМИИ НАУК СССР]. tape 1 , no. 3 , 1937, pp. 355–359 (Russian, online at Math-Net.Ru ): «The author gives a strict solution to the following schematic task: Crystallization centers occur randomly in the unlimited space […]»
4. ^ English translation: Andrei Nikolaevich Kolmogorov: Selected works of AN Kolmogorov Volume II, Probability theory and mathematical statistics . Translated from the Russian by G. Lindquist. Ed .: Albert Nikolaevich Shiryayev (= M. Hazewinkel [Ed.]: Mathematics and Its Applications (Soviet Series) . Volume 2 , no. 26 ). Kluwer Academic Publishers, Springer, Dordecht, Boston, London 1992, ISBN 94-010-5003-1 , On The Statistical Theory of Metal Crystallization, pp. 188–192 , doi : 10.1007 / 978-94-011-2260-3_22 (English, limited preview in Google Book Search - Russian: СТАТИСТИЧЕСКОЙ ТЕОРИИ КРИСТАЛЛИЗАЦИИ МЕТАЛЛОВ , online preview at Springer . 1937. Translated by G. Linder ).
5. ^ William A. Johnson. In: AIME Rossiter W. Raymond Memorial Award. American Institute of Mining, Metallurgical, and Petroleum Engineers, accessed November 15, 2017 .  
6. ↑ ^{a b c} Katayun Barmak: A Commentary on: “Reaction Kinetics in Processes of Nucleation and Growth” * . In: Metallurgical and Materials Transactions A . tape 41 , no. 11 , November 1, 2010, ISSN  1073-5623 , p. 2711-2775 , doi : 10.1007 / s11661-010-0421-1 (with a reprint of the original (1939) by Johnson and Mehl on pages 2713-2738).
7. ^ William A. Johnson, Robert F. Mehl: Reaction Kinetics in Processes of Nucleation and Growth . New York Meeting, February 1939. In: American Institute of Mining and Metallurgical Engineers AIME (Ed.): Transactions of the AIME . tape 135 , 1939, pp. 416–442 (English, discussion of the work on pages 442–458. Reprinted by see Katayun Barmak, A Commentary on: “Reaction Kinetics in Processes of Nucleation and Growth”, 2010): “the reaction proceeds by nucleation and growth […] The rate of nucleation […] and the rate of radial growth […] are both constant throughout the reaction ”
8. ^ Melvin Avrami: Kinetics of Phase Change. I general theory . In: The Journal of Chemical Physics . tape 7 , no. 12 , December 1, 1939, ISSN  0021-9606 , p. 1103-1112 , doi : 10.1063 / 1.1750380 ( scitation.org ).
9. ^ Melvin Avrami: Kinetics of Phase Change. II Transformation-Time Relations for Random Distribution of Nuclei . In: The Journal of Chemical Physics . tape 8 , no. 2 , February 1, 1940, ISSN  0021-9606 , p. 212-224 , doi : 10.1063 / 1.1750631 .
10. ↑ Melvin Avrami: Granulation, Phase Change, and Microstructure . Kinetics of Phase Change. III. In: The Journal of Chemical Physics . tape 9 , no. 2 , February 1, 1941, ISSN  0021-9606 , p. 177-184 , doi : 10.1063 / 1.1750872 .
11. ↑ Lecture 15: Kinetics of Phase Growth