Binomial formulas

The binomial formulas are common formulas in elementary algebra for transforming products from binomials . They are used as shopping formulas, first, the multiplying of parenthetical expressions facilitate, on the other hand they allow the factorization of terms , ie the conversion of certain sums and differences in products , resulting in the simplification of breaking terms , the root extraction of root terms and Logarithmenausdrücken very often represents the only solution strategy. Basically they are special cases of the distributive law for algebraic sums (each term of one is multiplied by each of the other sums)

${\displaystyle (a+b)(c+d)=ac+ad+bc+bd}$

with , and the corresponding sign variants. ${\displaystyle c=a}$ ${\displaystyle d=b}$

The adjective binomial is derived from the noun binomial , i.e. from bi (two) and nouns (names). The binomial formulas apply to all commutative rings .

Formulas

The following three transformations are usually referred to as binomial formulas :

${\displaystyle (a+b)^{2}=a^{2}+2ab+b^{2}}$		first binomial formula (plus formula)
${\displaystyle (a-b)^{2}=a^{2}-2ab+b^{2}}$		second binomial formula (minus formula)
${\displaystyle (a+b)\cdot (a-b)=a^{2}-b^{2}}$		third binomial formula (plus-minus formula)

The validity of the formulas can be seen by multiplying :

${\displaystyle (a+b)^{2}=(a+b)\cdot (a+b)=a\cdot a+a\cdot b+b\cdot a+b\cdot b=a^{2}+2\cdot a\cdot b+b^{2}}$

${\displaystyle (a-b)^{2}=(a-b)\cdot (a-b)=a\cdot a-a\cdot b-b\cdot a+b\cdot b=a^{2}-2\cdot a\cdot b+b^{2}}$

${\displaystyle (a+b)\cdot (a-b)=a\cdot a-a\cdot b+b\cdot a-b\cdot b=a^{2}-b^{2}}$

Geometric illustration

	The adjacent multicolored square has the length of the side . As you can see at once, the two squares and fit in, leaving two rectangles each with the same area . ${\displaystyle (a+b)}$ ${\displaystyle a^{2}}$ ${\displaystyle b^{2}}$ ${\displaystyle a\cdot b}$ This results in . ${\displaystyle (a+b)^{2}=a^{2}+2\cdot a\cdot b+b^{2}}$
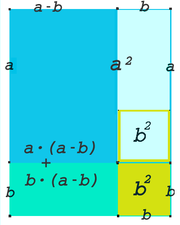
	In the second picture is the square framed in blue (large square, so, despite the same name as in the first binomial formula, a different square!). If a square of the length of the side is to be generated from this, the area framed in red (the rectangle) is first subtracted (again, despite the same name as in the first binomial formula, another rectangle). Then the equally large, lying area is deducted. But now you have deducted the small square twice, you have to add it again (for correction). ${\displaystyle a^{2}}$ ${\displaystyle (a-b)}$ ${\displaystyle a\cdot b}$ ${\displaystyle b^{2}}$ So the formula shown here is . ${\displaystyle (a-b)^{2}=a^{2}-2\cdot a\cdot b+b^{2}}$
	In the third picture is the light and dark blue square. If the small square (yellow frame) is subtracted from it and the remaining light blue rectangle is attached rotated below (shown in turquoise), a rectangle of width and height is created (from the dark blue and turquoise area) . ${\displaystyle a^{2}}$ ${\displaystyle b^{2}}$ ${\displaystyle (a-b)}$ ${\displaystyle (a+b)}$ So the formula results . ${\displaystyle a^{2}-b^{2}=(a+b)\cdot (a-b)}$

Another illustration of the third binomial formula can be obtained from the following decomposition:

Importance and uses

Tricks for mental arithmetic

These formulas, which are often used in mathematics , also help with mental arithmetic. The square of any number between 10 and 100 can often be easily determined using the binomial formula by reducing the calculation to squares of simpler numbers (multiples of 10 or single-digit numbers). For example is

${\displaystyle 37^{2}=(30+7)^{2}=30^{2}+2\cdot 30\cdot 7+7^{2}=900+420+49=1369}$

or

${\displaystyle 37^{2}=(40-3)^{2}=40^{2}-2\cdot 40\cdot 3+3^{2}=1600-240+9=1369}$.

If the square numbers up to 20 are known, many multiplications can be traced back to the third binomial formula. For example is

${\displaystyle 17\cdot 13=(15+2)\cdot (15-2)=15^{2}-2^{2}=225-4=221}$.

With the help of the binomial formulas, multiplication and division can be reduced to the simpler arithmetic methods of squaring, adding, subtracting, halving and doubling:

The first and second binomial formulas yield for the product of two numbers and : ${\displaystyle a}$ ${\displaystyle b}$

${\displaystyle a\cdot b=\left((a+b)^{2}-a^{2}-b^{2}\right)/2=\left(b^{2}+a^{2}-(a-b)^{2}\right)/2}$

If you know the first hundred square numbers instead of the multiplication table, you can easily calculate the general product of two numbers. In the absence of a numerical system with zero, the Babylonians can be shown to have calculated this way and throughout ancient times and in the Middle Ages this will have been the case. The alleged inconvenience of the ancient number systems is put into perspective, since one could add and subtract very well with these number systems.

Addition and subtraction of roots

The first and second binomial formulas also provide a calculation method for adding and subtracting roots, respectively. Since or are not directly calculable, the sum or difference is squared and then the square root is extracted. However, the procedure leads to box roots that are not necessarily simpler than the original expressions. ${\displaystyle {\sqrt {a}}+{\sqrt {b}}}$ ${\displaystyle {\sqrt {a}}-{\sqrt {b}}}$

${\displaystyle {\sqrt {a}}+{\sqrt {b}}={\sqrt {\left({\sqrt {a}}+{\sqrt {b}}\right)^{2}}}={\sqrt {{a}+{b}+2{\sqrt {ab}}}}}$

${\displaystyle {\sqrt {10}}+{\sqrt {11}}={\sqrt {{10}+{11}+2{\sqrt {110}}}}={\sqrt {{21}+2{\sqrt {110}}}}}$

Since roots are defined as nonnegative and squares are never negative by themselves, a case distinction is necessary for differences in roots:

${\displaystyle {\sqrt {a}}-{\sqrt {b}}=+{\sqrt {{a}+{b}-2{\sqrt {ab}}}}}$ For ${\displaystyle a>b}$

${\displaystyle {\sqrt {a}}-{\sqrt {b}}=-{\sqrt {{a}+{b}-2{\sqrt {ab}}}}}$ For ${\displaystyle a<b}$

Powers of complex numbers (in arithmetic representation)

The binomial formulas are also used to calculate powers of complex numbers , with the real part and the imaginary part being: ${\displaystyle a}$ ${\displaystyle b}$

${\displaystyle (a+ib)^{2}=a^{2}-b^{2}+2iab}$

${\displaystyle (a+ib)^{3}=a^{3}-3ab^{2}+i\left(3a^{2}b-b^{3}\right)}$

${\displaystyle (a+ib)^{4}=a^{4}-6a^{2}b^{2}+b^{4}+i\left(4a^{3}b-4ab^{3}\right)}$

A factorization of can also be derived from the third binomial formula by writing the sum of squares as the difference: ${\displaystyle a^{2}+b^{2}}$

${\displaystyle a^{2}+b^{2}=a^{2}-\left(-b^{2}\right)=a^{2}-(ib)^{2}=(a+ib)(a-ib)}$.

The third binomial formula is not only a mental arithmetic trick, but also provides a method to reduce division to multiplication and a simpler division. For example, expanding a fraction with a denominator with the so-called conjugate makes the denominator rational. Similarly, division by complex (and hyper- complex ) numbers can be transformed into division by real numbers (see rationalization (fraction calculation) ). ${\displaystyle {\sqrt {a}}+{\sqrt {b}}}$ ${\displaystyle {\sqrt {a}}-{\sqrt {b}}}$

2nd degree extended formulas

Some special formulas are derived from the binomial formulas, which also have a certain meaning for number theory :

• Babylonian multiplication formula: (see above) ${\displaystyle a\cdot b=\left((a+b)^{2}-(a-b)^{2}\right)/4}$
• Formula for Pythagorean triples : Example: returns . ${\displaystyle \left(a^{2}+b^{2}\right)^{2}=\left(a^{2}-b^{2}\right)^{2}+(2ab)^{2}}$ ${\displaystyle a=4,b=1}$ ${\displaystyle 17^{2}=15^{2}+8^{2}}$
• Identity of Diophantos : Example: delivers . ${\displaystyle (ac+bd)^{2}+(ad-bc)^{2}=(ac-bd)^{2}+(ad+bc)^{2}}$ ${\displaystyle a=1,b=2,c=5,d=7}$ ${\displaystyle 19^{2}+3^{2}=17^{2}+9^{2}}$
• Brahmagupta identity : ${\displaystyle \left(a^{2}+b^{2}\right)\left(c^{2}+d^{2}\right)=(ac+bd)^{2}+(ad-bc)^{2}}$

Higher powers and factorizations of power sums

Binomial formulas can also be given for higher powers , this generalization is the binomial theorem :

${\displaystyle (a+b)^{n}=\sum _{k=0}^{n}{\binom {n}{k}}a^{n-k}\cdot b^{k},n\in \mathbb {N} }$

The binomial coefficients denote that can be easily determined, for example, using the Pascal triangle . The first and second binomial formulas are special cases of the binomial theorem for : ${\displaystyle {\tbinom {n}{k}}={\tfrac {n!}{k!(n-k)!}}}$ ${\displaystyle n=2}$

${\displaystyle (a+b)^{2}={\binom {2}{0}}a^{2}b^{0}+{\binom {2}{1}}a^{1}b^{1}+{\binom {2}{2}}a^{0}b^{2}=a^{2}+2ab+b^{2},}$

${\displaystyle (a-b)^{2}={\binom {2}{0}}a^{2}(-b)^{0}+{\binom {2}{1}}a^{1}(-b)^{1}+{\binom {2}{2}}a^{0}(-b)^{2}=a^{2}-2ab+b^{2}.}$

For z. B. ${\displaystyle n=3}$

${\displaystyle (a\pm b)^{3}=a^{3}\pm 3a^{2}b+3ab^{2}\pm b^{3}}$.

A generalization to unnecessarily natural exponents leads to a power series expansion which is given by the binomial series .

There is also a generalization of the third binomial formula that enables the factorization of : ${\displaystyle a^{n}{-}b^{n}}$

${\displaystyle a^{3}-b^{3}=(a-b)\cdot \left(a^{2}+ab+b^{2}\right)}$

${\displaystyle a^{4}-b^{4}=(a-b)\cdot \left(a^{3}+a^{2}b+ab^{2}+b^{3}\right)}$

or generally for higher natural potencies

${\displaystyle {\begin{aligned}a^{n}-b^{n}&=(a-b)\cdot (a^{n-1}+a^{n-2}b+a^{n-3}b^{2}+\dotsb +a^{2}b^{n-3}+ab^{n-2}+b^{n-1})\\&=(a-b)\cdot \sum _{k=0}^{n-1}a^{k}b^{n-1-k}\\&=(a-b)\cdot a^{n-1}\cdot \sum _{k=0}^{n-1}\left({\frac {b}{a}}\right)^{k}\end{aligned}}}$

An expression can always be split off; a sum is obtained as the residual polynomial. If a prime number, this residual polynomial is irreducible; further decompositions are only possible using the complex numbers. Otherwise, the sum can be broken down further and is a product of 3 or more different odd prime numbers, polynomials with coefficients other than 0, −1, +1 also arise. The decomposition of a polynomial, starting with ${\displaystyle a^{n}-b^{n}}$ ${\displaystyle a-b}$ ${\displaystyle n}$ ${\displaystyle n}$ ${\displaystyle a^{105}-b^{105}}$

${\displaystyle a^{48}+a^{47}b+a^{46}b^{2}-a^{43}b^{5}-a^{42}b^{6}-2a^{41}b^{7}-a^{40}b^{8}\dotso }$

With one receives the so-called circle division polynomials as residual polynomials . ${\displaystyle b=1}$

In the case of a straight line one can even always and therefore split off; the division produces an alternating sum as the residual polynomial: ${\displaystyle n}$ ${\displaystyle a^{2}-b^{2}}$ ${\displaystyle a+b}$

${\displaystyle {\tfrac {a^{n}-b^{n}}{a+b}}=a^{n-1}-a^{n-2}b+\dotsb +ab^{n-2}-b^{n-1}}$ For ${\displaystyle n=2,4,6,\dotsc }$

A factorization of is also possible if is odd. Here, too, there is an alternating sum, this time with an even exponent as the highest and a positive term at the end, e.g. B .: ${\displaystyle a^{n}+b^{n}}$ ${\displaystyle n}$

${\displaystyle a^{3}+b^{3}=(a+b)\cdot \left(a^{2}-ab+b^{2}\right)}$

${\displaystyle a^{5}+b^{5}=(a+b)\cdot \left(a^{4}-a^{3}b+a^{2}b^{2}-ab^{3}+b^{4}\right)}$

For even , a factorization of over the complex numbers is possible, but only essential for: ${\displaystyle n}$ ${\displaystyle a^{n}+b^{n}}$ ${\displaystyle n=2}$

${\displaystyle a^{2}+b^{2}=(a+ib)\cdot (a-ib)}$ (see above)

With the help of the Sophie-Germain identity, it can already be split into two quadratic factors with real coefficients: ${\displaystyle a^{4}+b^{4}}$

${\displaystyle a^{4}+b^{4}=\left(a^{2}+{\sqrt {2}}ab+b^{2}\right)\left(a^{2}-{\sqrt {2}}ab+b^{2}\right)}$. As well

${\displaystyle a^{4}+1=\left(a^{2}+{\sqrt {2}}a+1\right)\left(a^{2}-{\sqrt {2}}a+1\right)}$

Thus, for all higher straight lines, a factorization into higher order factors is possible, e.g. B .: ${\displaystyle n}$

${\displaystyle a^{6}+b^{6}=\left(a^{2}+b^{2}\right)\cdot \left(a^{4}-a^{2}b^{2}+b^{4}\right)}$

Only if both irreducible factors are broken down further, for example into linear factors , do complex coefficients arise.

The factoring of

${\displaystyle a^{6}-b^{6}=\left(a^{2}-b^{2}\right)\left(a^{4}+a^{2}b^{2}+b^{4}\right)=(a+b)(a-b)\left(a^{2}-ab+b^{2}\right)\left(a^{2}+ab+b^{2}\right)}$

or.

${\displaystyle a^{6}-1=\left(a^{2}-1\right)\left(a^{4}+a^{2}+1\right)=(a-1)(a+1)\left(a^{2}-a+1\right)\left(a^{2}+a+1\right)}$

The non-trivial decomposition of the remainder of the 4th degree polynomial into two quadratic polynomials is used for solving equations of the 4th degree . The other remainder polynomials (see above) or are, however, irreducible. ${\displaystyle \left(a^{4}-a^{2}b^{2}+b^{4}\right)}$ ${\displaystyle \left(a^{4}-a^{2}+1\right)}$

A division of by is generally not possible without a remainder. ${\displaystyle a^{n}+b^{n}}$ ${\displaystyle a-b}$

Extensions to multi-part expressions

A generalization of the binomial formulas to powers of polynomials , i.e. sums with more than two terms, leads to the multinomial theorem . For example, the square of a trinomial applies

${\displaystyle (a+b+c)^{2}=a^{2}+b^{2}+c^{2}+2ab+2ac+2bc}$.

The coefficients are contained in the Pascal pyramid . So is

${\displaystyle (a+b+c)^{3}=a^{3}+b^{3}+c^{3}+3a^{2}b+3a^{2}c+3b^{2}c+3ab^{2}+3ac^{2}+3bc^{2}+6abc}$

Sample application

${\displaystyle {\begin{aligned}(5+3x)^{3}&=1\cdot 5^{3}\cdot (3x)^{0}+3\cdot 5^{2}\cdot (3x)^{1}+3\cdot 5^{1}\cdot (3x)^{2}+1\cdot 5^{0}\cdot (3x)^{3}\\&=125+75\cdot 3x+15\cdot 9x^{2}+1\cdot 27x^{3}\\&=125+225x+135x^{2}+27x^{3}\end{aligned}}}$

Trivia

In contrast to adjectives like abelsch , binomial is not derived from the name of a mathematician. In the sense of the scientific joke , the term binomial is jokingly traced back to a fictional mathematician named Alessandro (or Francesco) Binomi , who optionally appears as the author of some school and textbooks.

literature

• Harald Ludwig, Christian Fischer, Reinhard Fischer (eds.): Understanding learning in Montessori pedagogy. Upbringing and education in view of the challenges of the Pisa study (= impulses of reform pedagogy. Vol. 8). Lit-Verlag, Münster 2003, ISBN 3-8258-7063-4 , pp. 100-101 ( excerpt (Google) ).
• Albrecht Beutelspacher : Albrecht Beutelspacher's Small Mathematical Course. The 101 most important questions and answers about mathematics. CH Beck, sl 2011, ISBN 978-3-406-61658-7 , Chapter 50: What are the binomial formulas good for?

Individual evidence

1. ↑ Hans-Jochen Bartsch: Pocket book of mathematical formulas. 19th edition Fachbuchverlag Leipzig by Carl Hanser Verlag, Munich / Vienna 2001, p. 64.
2. ↑ Hans-Jochen Bartsch: Pocket book of mathematical formulas. 19th edition Fachbuchverlag Leipzig by Carl Hanser Verlag, Munich / Vienna 2001, p. 60.
3. ^ Heinrich Zankl: Insane things from science . Wiley 2012, ISBN 978-3-527-64142-0 , chapter Sonorous names ( excerpt (Google) )

Web links

• Binomial formulas calculator with calculation method and variables
• Binomial formulas - multiple choice test
• Binomial formulas - exercises with a solution
• Binomial formulas calculator with calculation method and helpful video explanations
• Binomial formulas . In: Serlo .