Spring pendulum

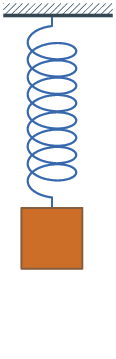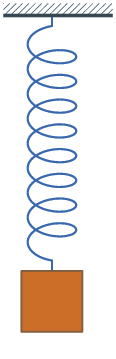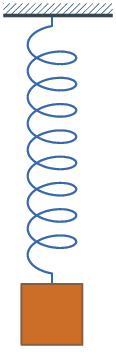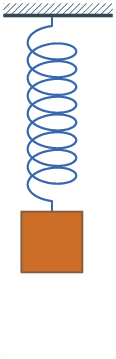
Movement of an undamped spring oscillator

A spring pendulum or spring oscillator is a harmonic oscillator consisting of a helical spring and a mass attached to it, which can move in a straight line along the direction in which the spring is elongated or contracted. If the mass moves in the vertical direction , gravity influences the rest position .

When the spring oscillator , which has been deflected from its rest position, is released, a harmonic oscillation begins which, if there is no damping, no longer decays.

This article does not deal with the pendulum movement to the side, which is also possible and can lead to chaotic behavior.

functionality

An ideal spring exerts a force on the mass that is composed of the force in the rest position and a proportion proportional to the distance from the rest position . The force in the rest position compensates for the weight and has no effect on the vibration behavior. The portion proportional to the deflection always has a restoring effect. A deflected spring oscillator therefore always strives to return to the rest position. Its mass is accelerated in the direction of the rest position and swings beyond it again due to the principle of inertia .

The potential energy stored in the spring is converted into kinetic energy of the mass. If there is no damping, no energy is withdrawn from the system, so this process is repeated periodically with a constant amplitude .

If the spring oscillator is periodically excited by an external force, the amplitude can become very large and lead to a resonance catastrophe .

Derivation of the oscillation equation

Force on a spring oscillator. The spring force F acts towards the rest position.

According to Hooke's law, the spring force acting on the mass is proportional to the deflection  y .

${\displaystyle F=D\cdot y}$

The proportionality factor D is the spring constant or direction constant .

According to the principle of action, the spring force causes the mass to accelerate against the deflection. The acceleration can also be expressed as the second derivative of the displacement with respect to time.

${\displaystyle m\cdot {\ddot {y}}=-F=-D\cdot y}$

After transforming the equation, one finally obtains

${\displaystyle m\cdot {\ddot {y}}+D\cdot y=0\Rightarrow {\ddot {y}}+{\frac {D}{m}}\cdot y=0}$

${\displaystyle {\ddot {y}}+\omega _{0}^{2}\cdot y=0}$

a linear homogeneous differential equation that can be solved using an exponential approach.

${\displaystyle \omega _{0}}$is called the undamped natural angular frequency .

${\displaystyle \omega _{0}={\sqrt {\frac {D}{m}}}}$

The natural angular frequency is general , the changeover after the period T results ${\displaystyle \omega _{0}={\tfrac {2\cdot \pi }{T}}}$

${\displaystyle T=2\cdot \pi \cdot {\sqrt {\frac {m}{D}}}}$

The period indicates the time required for an entire oscillation.

Solve the oscillation equation

The deflection is an exponential function of the form . The second derivative of the function is according to the chain rule ${\displaystyle y(t)=c\cdot e^{\lambda \cdot t}}$

${\displaystyle {\ddot {y}}(t)=c\cdot \lambda ^{2}\cdot e^{\lambda \cdot t}}$

Inserting y into the oscillation equation yields

${\displaystyle c\cdot \lambda ^{2}\cdot e^{\lambda \cdot t}+\omega _{0}^{2}\cdot c\cdot e^{\lambda \cdot t}=c\cdot e^{\lambda \cdot t}\cdot (\lambda ^{2}+\omega _{0}^{2})=0}$

According to the theorem of zero product, or must be zero. e-functions are for never zero. Therefore the so-called characteristic equation must be fulfilled. ${\displaystyle c\cdot e^{\lambda \cdot t}}$ ${\displaystyle \lambda ^{2}+\omega _{0}^{2}}$ ${\displaystyle c\neq 0}$ ${\displaystyle \lambda ^{2}+\omega _{0}^{2}=0}$

${\displaystyle \lambda ^{2}+\omega _{0}^{2}=0}$

${\displaystyle \lambda _{1/2}=\pm {\sqrt {-\omega _{0}^{2}}}=\pm \omega _{0}\cdot \mathrm {i} }$

For there are two complex solutions: ${\displaystyle \lambda }$

${\displaystyle y_{1}=c_{1}\cdot e^{\omega _{0}\cdot \mathrm {i} \cdot t}}$

and

${\displaystyle y_{2}=c_{2}\cdot e^{-\omega _{0}\cdot \mathrm {i} \cdot t}}$

The two solutions for and can be added. For the deflection y of the spring oscillator we get: ${\displaystyle y_{1}}$ ${\displaystyle y_{2}}$

${\displaystyle {\underline {y(t)=c_{1}\cdot e^{\omega _{0}\cdot \mathrm {i} \cdot t}+c_{2}\cdot e^{-\omega _{0}\cdot \mathrm {i} \cdot t}}}}$

The constants and must be determined. At the beginning of the oscillation are and . After a quarter of a period T , the oscillator has reached its maximum deflection . ${\displaystyle c_{1}}$ ${\displaystyle c_{2}}$ ${\displaystyle t=0}$ ${\displaystyle y=0}$ ${\displaystyle {\hat {y}}}$

${\displaystyle y(0)=c_{1}+c_{2}=0\quad \Rightarrow \quad c_{1}=-c_{2}}$

${\displaystyle y\left({\frac {T}{4}}\right)=y\left({\frac {2\pi }{4\cdot \omega _{0}}}\right)=c_{1}\cdot e^{{\frac {\pi }{2}}\mathrm {i} }+c_{2}\cdot e^{-{\frac {\pi }{2}}\mathrm {i} }={\hat {y}}}$

The complex exponential function can be converted into sine and cosine using Euler's formula .

${\displaystyle {\hat {y}}=c_{1}\cdot \left[\cos \left({\frac {\pi }{2}}\right)+\mathrm {i} \cdot \sin \left({\frac {\pi }{2}}\right)\right]+c_{2}\cdot \left[\cos \left(-{\frac {\pi }{2}}\right)+\mathrm {i} \cdot \sin \left(-{\frac {\pi }{2}}\right)\right]}$

${\displaystyle {\hat {y}}=c_{1}\cdot \mathrm {i} -c_{2}\cdot \mathrm {i} }$

Inserting supplies ${\displaystyle c_{1}=-c_{2}}$

${\displaystyle {\hat {y}}=2c_{1}\cdot i}$ and ${\displaystyle {\hat {y}}=-2\cdot c_{2}\cdot \mathrm {i} }$

We therefore get and . The constants can now be used in the trigonometric representation of the deflection function, which is then transformed taking into account the quadrant relationships and . ${\displaystyle c_{1}={\frac {\hat {y}}{2\mathrm {i} }}}$ ${\displaystyle c_{2}=-{\frac {\hat {y}}{2\mathrm {i} }}}$ ${\displaystyle \sin(-h)=-\sin(h)}$ ${\displaystyle \cos(k)=\cos(-k)}$

${\displaystyle y(t)={\frac {\hat {y}}{2\mathrm {i} }}\cdot [{\cancel {\cos(\omega _{0}\cdot t)}}+\mathrm {i} \cdot \sin(\omega _{0}\cdot t)]-{\frac {\hat {y}}{2\mathrm {i} }}\cdot [{\cancel {\cos(-\omega _{0}\cdot t)}}+\mathrm {i} \cdot \sin(-\omega _{0}\cdot t)]={\frac {\hat {y}}{2\mathrm {i} }}\cdot 2\cdot \mathrm {i} \cdot \mathrm {\sin(} \omega _{0}\cdot t)}$

The oscillation equation for the ideal spring oscillator without deflection at the beginning of the oscillation is ( ) ${\displaystyle \varphi _{0}=0}$

${\displaystyle {\underline {\underline {y(t)={\hat {y}}\cdot \sin(\omega _{0}\cdot t)}}}}$

Energy of a spring oscillator

The kinetic energy of a spring oscillator with the mass m can be calculated with . ${\displaystyle E_{\mathrm {kin} }={\frac {1}{2}}\cdot m\cdot v^{2}}$

After the onset of the velocity v one obtains

${\displaystyle E_{\mathrm {kin} }={\frac {1}{2}}m\cdot {\hat {y}}^{2}\cdot \omega _{0}^{2}\cdot \cos ^{2}(\omega _{0}\cdot t+\varphi _{0})}$.

The following applies to the natural angular frequency . Therefore the kinetic energy can also be expressed with: ${\displaystyle \omega _{0}={\sqrt {\frac {D}{m}}}\Rightarrow D=m\cdot \omega _{0}^{2}}$

${\displaystyle E_{\mathrm {kin} }={\frac {D}{2}}{\hat {y}}^{2}\cdot \cos ^{2}(\omega _{0}\cdot t+\varphi _{0})}$

The potential energy is general

${\displaystyle E_{\mathrm {pot} }=\int F_{\mathrm {F} }\,\mathrm {d} s}$ For ${\displaystyle F\parallel s}$

Since the spring force is, applies ${\displaystyle F_{\mathrm {F} }=D\cdot y}$

${\displaystyle E_{\mathrm {pot} }=D\cdot \int y\,\mathrm {d} y}$

${\displaystyle E_{\mathrm {pot} }={\frac {D}{2}}y^{2}}$

The total spring energy E _F is made up of the potential and the kinetic energy.

${\displaystyle E_{\mathrm {F} }=E_{\mathrm {pot} }+E_{\mathrm {kin} }}$

${\displaystyle E_{\mathrm {F} }={\frac {D}{2}}{\hat {y}}^{2}\cdot \sin ^{2}(\omega _{0}\cdot t+\varphi _{0})+{\frac {D}{2}}{\hat {y}}^{2}\cdot \cos ^{2}(\omega _{0}\cdot t+\varphi _{0})}$

Due to the " trigonometric Pythagoras " , the total energy is simplified to: ${\displaystyle \sin ^{2}x+\cos ^{2}x=1}$

${\displaystyle {\underline {\underline {E_{\mathrm {F} }={D \over 2}\cdot {\hat {y}}^{2}}}}}$

Massed spring

The equations of motion for ideal spring oscillators only apply to massless springs. If the elastic spring is assumed to have mass and the mass is homogeneously distributed, the period of the oscillation results in

${\displaystyle T=2\pi {\sqrt {\frac {m+{\frac {1}{3}}m_{F}}{D}}}}$

The parameters m and m _F correspond to the mass of the oscillator and the mass of the spring.

The total length of the spring is l , s is the distance between the suspension of the spring oscillator and any point on the spring. A section of the spring with the length d s then has the mass . The speed of the spring section is because it increases linearly with increasing distance from the suspension. It follows from this for the kinetic energy of a spring section ${\displaystyle \mathrm {d} m_{F}=m_{F}\cdot {\frac {\mathrm {d} s}{l}}}$ ${\displaystyle v_{F}={\dot {y}}{\frac {s}{l}}}$

${\displaystyle \mathrm {d} E_{\mathrm {kin,F} }={\frac {1}{2}}\cdot \mathrm {d} m_{F}\cdot v_{F}^{2}}$

${\displaystyle \mathrm {d} E_{\mathrm {kin,F} }={\frac {1}{2}}\cdot m_{F}\cdot {\frac {\mathrm {d} s}{l}}\cdot {\dot {y}}^{2}\cdot {\frac {\mathrm {s} ^{2}}{l^{2}}}={\frac {1}{2}}\cdot m_{F}\cdot {\dot {y}}^{2}\cdot {\frac {1}{l^{3}}}\cdot s^{2}\mathrm {d} s}$

The total kinetic energy of the spring is obtained by integrating:

${\displaystyle E_{\mathrm {kin,F} }=\int \mathrm {d} E_{\mathrm {kin} }={\frac {1}{2}}\cdot m_{F}\cdot {\dot {y}}^{2}\cdot {\frac {1}{l^{3}}}\cdot \int _{0}^{l}s^{2}\mathrm {d} s}$

${\displaystyle E_{\mathrm {kin,F} }={\frac {1}{2}}\cdot m_{F}\cdot {\dot {y}}^{2}\cdot {\frac {1}{3}}}$

The kinetic energy of a spring oscillator taking into account the massed spring is

${\displaystyle E_{\mathrm {kin} }={\frac {1}{2}}\cdot {\dot {y}}^{2}\cdot \left(m+{\frac {1}{3}}m_{F}\right)}$

You can see that a third of the spring's mass behaves as if it were part of the mass of the body. From this follows the period duration described above for a mass spring.

literature

• Dieter Meschede: Gerthsen Physics . Edition 23, Springer, Berlin Heidelberg New York 2006, ISBN 3-540-02622-3 .
• Istvan Szabo : Introduction to technical mechanics . Edition 8, Springer, 2002, ISBN 3-540-44248-0 .