Higgs mechanism

Five of the six winners of the APS Sakurai Prize for 2010: Kibble, Guralnik, Hagen, Englert and Brout; Higgs wasn't there.

The sixth: Peter Higgs 2009

The Higgs mechanism describes how the fundamental property “ mass ” comes about at the level of elementary particles . As a central component of the Standard Model of elementary particle physics, the mechanism explains why certain exchange particles (the “ gauge bosons ” of the weak interaction ) do not have zero mass. Accordingly, they gain their mass through interaction with the so-called Higgs field , which is omnipresent in the entire universe. The masses of all other (massy) elementary particles such as electrons and quarks are also explained here as a result of the interaction with the Higgs field. With this approach, it became possible to interpret the weak and electromagnetic interactions as two differently strong aspects of a single fundamental electroweak interaction , which is one of the most important steps in establishing the Standard Model.

While the Higgs field cannot be measured directly, another elementary particle must appear when it exists, the “ Higgs boson ”. For a long time, this was the only particle in the Standard Model that could not be definitively proven; meanwhile, the existence of a Higgs-like boson is considered certain.

The mechanism was found in 1964 not only by Peter Higgs , but also independently and almost simultaneously by two research groups: by François Englert and Robert Brout at the Université Libre de Bruxelles (submitted a little earlier) and by TWB Kibble , Carl R. Hagen and Gerald Guralnik at Imperial College . The mechanism is therefore also called the Brout-Englert-Higgs mechanism or Englert-Brout-Higgs-Guralnik-Hagen-Kibble mechanism . However, Peter Higgs was the first to also predict the existence of a new particle, which is why it was named after him. On October 8, 2013, François Englert and Peter Higgs were awarded the Nobel Prize in Physics for developing the Higgs mechanism ; Robert Brout had died a year earlier.

history

Role models in solid state theory

The elaboration of Higgs' theory in 1964 was based on a proposal by Philip Warren Anderson from 1962 from solid state physics , i.e. from a non-relativistic environment. A similar mechanism was developed by Ernst Stückelberg as early as 1957 .

Such a mechanism for the mathematically simpler Abelian gauge symmetries , such as electromagnetic interaction , was originally proposed in solid-state physics. The Ginsburg-Landau theory published in 1950 describes in full how magnetic fields are forced out of superconducting metals by the Meissner-Ochsenfeld effect . As a phenomenological theory with far-reaching nontrivial consequences, it is particularly suitable for translation into high-energy physics .

The effect mentioned is the finite - and very small - penetration depth of the magnetic field into the superconductor. This phenomenon can be interpreted as if the magnetic field - seen mathematically: a calibration field - had acquired a finite effective mass instead of zero mass due to superconductivity , according to the relationship ${\displaystyle \lambda }$ ${\displaystyle M_{\lambda }}$

${\displaystyle \lambda ={\frac {h}{M_{\lambda }c}}}$

where h is Planck's quantum and c is the speed of light . With normal line, however, is or . ${\displaystyle \lambda =\infty }$ ${\displaystyle M_{\lambda }=0}$

In contrast to the microscopic BCS theory of 1957, the Ginsburg-Landau theory did not yet predict the existence of Cooper pairs . Similarly, the experimental evidence of the existence of the Higgs mechanism is unlikely to provide a microscopic explanation for the nature of the Higgs boson.

Development towards the standard model

The Higgs mechanism was originally formulated only for Abelian gauge theories . After it was transferred to non- Abelian gauge theories ( Yang-Mills theories ) by TWB Kibble in 1967 , the mechanism could be applied to the weak interaction. This led to the prediction of the - experimentally confirmed in 1983 - large mass of the Z ⁰ , W ⁺ and W ^- responsible for the weak interaction .

In 1968, Abdus Salam applied the Higgs mechanism to the electroweak theory of Sheldon Lee Glashow and Steven Weinberg , creating the Standard Model of particle physics, for which all three received the 1979 Nobel Prize in Physics .

In predicting the Higgs boson, the phenomenon of spontaneous symmetry breaking of the Higgs field also plays a role. In addition to the physicists already mentioned, Yōichirō Nambu in 1960 (Nobel Prize 2008) and Jeffrey Goldstone in 1961 made important contributions.

Description in field theory

According to elementary particle physics, all forces are described by the exchange of so-called gauge bosons . These include B. the photons of quantum electrodynamics and the gluons of quantum chromodynamics . The photon and the gluons are massless. The exchange particles of the weak interaction , the W and Z bosons, on the other hand, have large masses of about 80 GeV / c² and 91 GeV / c² , respectively, compared to electrons, protons and neutrons . Among other things, these ensure that particles that decay according to the weak interaction have a comparatively long lifespan, so that radioactivity is a widespread, but relatively "weak phenomenon". Therefore one has to insert mass terms into the equations of motion for the named particles . Since the calibration fields with which the calibration bosons are described would then change during the so-called calibration transformations (these are local symmetries ), this is not possible. Because the properties of the basic forces are based precisely on the fact that the equations of motion do not change with gauge transformations ; this is called the "gauge invariance" of the equation of motion.

The standard model of elementary particles includes a. the electroweak interaction . In this theory there are four gauge bosons , the photon , the Z boson and the two W bosons . The last three of these four calibration bosons get their masses of 91 or 80 GeV / c ² and a longitudinal component due to the vacuum expectation value of the Higgs field, which is different from zero . In contrast, the photon that does not couple to the Higgs field remains massless and purely transversal.

Overall, the Higgs field that generates the masses contains an apparently “redundant” variable that corresponds to the Higgs boson. In the theory of superconductivity, the mass of the Higgs boson corresponds to the energy gap between the ground state and the excited states of the superconducting "condensate".

Higgs potential and spontaneous symmetry breaking

Definition of the Higgs potential

Higgs potential . For fixed values ​​of and , this quantity is plotted over the real and imaginary parts from above. Note the champagne bottle profile at the bottom of the potential. ${\displaystyle {\mathcal {V}}}$ ${\displaystyle \mu }$ ${\displaystyle \lambda }$ ${\displaystyle \phi }$

The Lagrange density of the Higgs field in the absence of other fields (particles) is in natural units : ${\displaystyle \phi }$

${\displaystyle {\mathcal {L'}}_{\text{Higgs}}(\phi )=(\partial _{\sigma }\phi )^{\dagger }(\partial ^{\sigma }\phi )+\mu ^{2}\phi ^{\dagger }\phi -\lambda (\phi ^{\dagger }\phi )^{2}}$.

Where and are positive, real parameters. The parameter has the physical dimension of a mass , the parameter is dimensionless. The symbol stands for the partial derivative . In this expression, Einstein's summation convention is used, so that multiple occurring indices are added: The sum above the Greek letters runs over the space-time indices from 0 to 3. ${\displaystyle \mu ^{2}}$ ${\displaystyle \lambda }$ ${\displaystyle \mu \approx 88{,}45\,{\text{GeV}}/c^{2}}$ ${\displaystyle \lambda \approx 0{,}18277}$ ${\displaystyle \partial }$ ${\displaystyle \sigma }$

In general, a term that contains twice the derivative operator and the field is called a kinetic term, a term that contains the second order field is called a mass term, and all other terms are called interaction terms.

The first two terms of this equation are almost identical to the free Klein-Gordon equation , but in comparison the sign before "false" is. So the idea of ​​the Higgs mechanism is to give the field an imaginary mass in contrast to a normal scalar boson , so that the square of the mass becomes negative. ${\displaystyle \mu ^{2}\phi ^{\dagger }\phi }$ ${\displaystyle \phi }$

The term describes an interaction between two - and two - fields with the coupling constant . ${\displaystyle \lambda (\phi ^{\dagger }\phi )^{2}}$ ${\displaystyle \phi }$ ${\displaystyle \phi ^{\dagger }}$ ${\displaystyle \lambda }$

Analogous to classical mechanics, the Higgs potential is defined as the negative of all terms that do not contain any derivative operators, i.e. ${\displaystyle {\mathcal {V}}}$

${\displaystyle {\mathcal {V}}=-\mu ^{2}\phi ^{\dagger }\phi +\lambda (\phi ^{\dagger }\phi )^{2}}$.

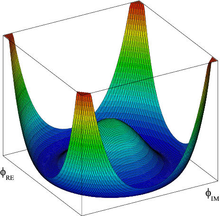
If and were a real number and not a complex field, and (that is, the mass was real), then the graph of this function would be a fourth-degree parabola open upwards with a minimum at the origin. Due to the imaginary mass, however, the graph clearly has the shape of a “W” with a maximum at the origin. If a complex number, the graph is the rotational figure of this "W", which is shown in the graphic opposite. Based on the bottom of a champagne bottle or sombrero, one speaks of the champagne bottle or sombrero potential. ${\displaystyle \phi }$ ${\displaystyle -\mu ^{2}\geq 0}$ ${\displaystyle \phi }$

Since in reality (the non-Abelian case) it is not only complex, but also has several components (similar to a vector ), a simple visualization and representation in reality is no longer possible. ${\displaystyle \phi }$

Spontaneous breaking of symmetry

In nature, every microscopic system strives for the smallest possible energy. In the case of the Higgs field, this means that, analogous to a marble in a ball track, it changes from the local maximum of the potential at the origin to a state on the “bottom” of the “champagne bottle”. This state of lowest energy is called the ground state . In the case of the Higgs potential, this ground state is degenerate , since all configurations on a circle around the origin correspond to the same energy. The random selection of exactly one of these states as the basic state reflects the concept of spontaneous symmetry breaking, since, to put it clearly, the “champagne bottle” no longer looks the same in all directions from this point.

It does not matter whether one is in the Abelian or non-Abelian case, since only the combination occurs in the potential , the minimum is always in a spherical shell with a distance ${\displaystyle \phi ^{\dagger }\phi }$

${\displaystyle \langle \phi \rangle ={\frac {v}{\sqrt {2}}}={\sqrt {\frac {\mu ^{2}}{2\lambda }}}}$

away from the origin. This value is called the vacuum expectation value (the square root of two in the denominator is convention). The name follows the fact that the field is expected to be in the vacuum state at such a value. The expected vacuum value has the dimension of an energy and can be calculated in the standard model from other known measured variables (see below). One finds for the value ${\displaystyle \phi }$ ${\displaystyle v}$

${\displaystyle v\approx 246\,{\text{GeV}}}$.

You can parameterize the (Abelian) Higgs field with two real parameters and the vacuum expectation value as follows: ${\displaystyle h}$ ${\displaystyle \chi }$

${\displaystyle \phi ={\frac {v+h}{\sqrt {2}}}e^{\mathrm {i} {\frac {\chi }{v}}}}$

This corresponds to the parameterization of complex numbers in polar form with a shifted origin. The field does not lose any free parameters because two real fields and have the same number of degrees of freedom as a complex field . ${\displaystyle h}$ ${\displaystyle \chi }$ ${\displaystyle \phi }$

If one now replaces the Higgs field in the original Lagrange density, this reads ${\displaystyle \phi }$

${\displaystyle {\mathcal {L}}'_{\text{Higgs}}(h,\chi )={\frac {1}{2}}\partial _{\sigma }h\partial ^{\sigma }h-\mu ^{2}h^{2}+{\frac {1}{2}}\partial _{\sigma }\chi \partial ^{\sigma }\chi +{\text{Wechselwirkung}}}$

Here, from a renewed comparison with the Klein-Gordon equation, the -field is a field with mass and the -field is massless. This situation corresponds to Goldstone's theorem that massless particles always occur in the event of spontaneous symmetry breaking; the particle is therefore called a Goldstone boson. The field , however, corresponds to a massive scalar boson Higgs boson . The different masses of the two fields clearly result from the direction of the deflection of the field in the potential: The field describes the polar component in which the "marble" can roll on the bottom of the "champagne bottle" without using energy, while the - Field describes the radial component in which energy has to be expended to transport the “marble” up the bottle wall. ${\displaystyle h}$ ${\displaystyle m_{h}={\sqrt {2}}\mu }$ ${\displaystyle \chi }$ ${\displaystyle \chi }$ ${\displaystyle h}$ ${\displaystyle \chi }$ ${\displaystyle h}$

Higgs potential at finite temperatures

Strictly speaking, the properties for the Higgs potential presented up to this point only apply at absolute temperature zero . At finite temperatures, effects from thermal field theory must also be taken into account. As early as 1972 Dawid Kirschniz and Andrei Linde showed that at sufficiently high temperatures the spontaneous symmetry breaking is canceled and the gauge bosons of the weak interaction become massless. Since the temperature at the beginning of the universe was extremely high, there must have been a phase transition of the Higgs field from the symmetrical phase to the broken phase since then. The temperature at which this happened was in the order of magnitude of over 110  GeV / k _B , i.e. 1.3 · 10 ¹⁵  K , just a few picoseconds after the Big Bang, the universe cooled down below this temperature.

Effect of spontaneous symmetry breaking on the gauge bosons

Conceptual example: Abelian model

For the generation of the mass of gauge bosons by the Higgs field, they must interact with the Higgs field. Therefore, additional interaction terms between a gauge boson field and the Higgs field must be included in the Lagrangian . The vacuum expectation value of the Higgs field, which differs from zero, also leads in these coupling terms to the coupling of the calibration bosons to the physical Higgs boson to a mass term for the calibration bosons. ${\displaystyle A}$ ${\displaystyle \phi }$ ${\displaystyle h}$

The coupling between the gauge bosons and other particles is achieved by replacing the partial derivative with the covariant derivative

${\displaystyle \partial ^{\sigma }\to D^{\sigma }=\partial ^{\sigma }-\mathrm {i} gA^{\sigma }}$

where is the coupling constant and the vector valued calibration field. With the explicit replacement of the covariant derivative, the Lagrangian is thus ${\displaystyle g}$ ${\displaystyle A^{\sigma }}$

${\displaystyle {\mathcal {L}}_{\text{Higgs}}(\phi ,A)=(\partial _{\sigma }\phi )^{\dagger }(\partial ^{\sigma }\phi )+\mu ^{2}\phi ^{\dagger }\phi +\mathrm {i} g\phi ^{\dagger }A_{\sigma }\partial ^{\sigma }\phi -\mathrm {i} g\partial _{\sigma }\phi ^{\dagger }A^{\sigma }\phi +g^{2}\phi ^{\dagger }A_{\sigma }A^{\sigma }\phi -\lambda (\phi ^{\dagger }\phi )^{2}}$.

If the -field is also repaired in the interaction terms , terms of the form arise there ${\displaystyle \phi }$

${\displaystyle {\mathcal {L}}(A,h,\chi )=-gvA_{\sigma }\partial ^{\sigma }\chi +{\frac {g^{2}v^{2}}{2}}A_{\sigma }A^{\sigma }+\dots }$.

The term that is quadratic in can again be interpreted as a mass term, so that the calibration field has a mass directly proportional to the vacuum expectation value. If the mass of the calibration boson and the coupling constant are known through measurements, the vacuum expectation value can be calculated using this relationship. ${\displaystyle A}$ ${\displaystyle m_{A}=gv}$

In addition, it happens that the interaction term can be interpreted as the conversion of a gauge boson into a Goldstone boson. This strange behavior can be eliminated by using the calibration fields ${\displaystyle A_{\mu }\partial ^{\mu }\chi }$

${\displaystyle A_{\mu }\to A_{\mu }^{'}=A_{\mu }-{\frac {1}{gv}}\partial _{\mu }\chi }$

be recalibrated. Correspondingly, the Higgs field must also go through

${\displaystyle \phi \to \phi '=\phi e^{-\mathrm {i} {\frac {\chi }{v}}}={\frac {v+h}{\sqrt {2}}}}$

be calibrated. As a result, the field no longer appears; In technical jargon one speaks of the fact that in the case of local gauge theories the gauge boson "eats up" the Goldstone boson. ${\displaystyle \chi }$

If one now counts the degrees of freedom of the theory, one began with a complex scalar field (2 degrees of freedom) and a massless vector field (2 degrees of freedom) and ends with a real scalar field (1 degree of freedom) and a massive vector field (3 degrees of freedom), so that the sum total is again consistent.

Higgs mechanism in the standard model

See also : standard model

The symmetry group broken by the Higgs mechanism in the Standard Model is , where is the circle group and the complex rotation group . The index symbolizes that this symmetry group is valid for leptons of left-handed chirality , which transform in the weak isospin doublet (the right-handed particles transform in a singlet), the index the weak hypercharge . ${\displaystyle SU(2)_{L}\otimes U(1)_{Y}}$ ${\displaystyle U(1)}$ ${\displaystyle SU(2)}$ ${\displaystyle L}$ ${\displaystyle Y}$

In contrast to the Abelian case, the covariant derivative, which operates on a left-handed particle doublet with a weak hypercharge , is in this case: ${\displaystyle Y}$

${\displaystyle D^{\sigma }=\partial ^{\sigma }-\mathrm {i} gT^{a}W_{a}^{\sigma }-{\frac {\mathrm {i} g'}{2}}YI_{2}B^{\sigma }}$.

Here, Einstein's sum convention is used again over the group index , which runs from 1 to 3 in the case of . Those are the three generators of the group; their representation can be found in the Pauli matrices . The three gauge bosons belonging to this symmetry group and the coupling constant are corresponding . The other term contains the individual calibration boson belonging to the circle group , for dimensional reasons the two-dimensional unit matrix as the generator of the group and another coupling constant . ${\displaystyle a}$ ${\displaystyle SU(2)}$ ${\displaystyle T^{a}}$ ${\displaystyle W_{a}}$ ${\displaystyle g}$ ${\displaystyle B}$ ${\displaystyle I_{2}}$ ${\displaystyle g'}$

The three massless bosons and the boson result from the Higgs mechanism, the two physical massive charged bosons, the uncharged massive boson and the uncharged massless photon. ${\displaystyle W_{a}}$ ${\displaystyle B}$ ${\displaystyle W^{\pm }}$ ${\displaystyle Z^{0}}$

The Higgs field must also be a left-handed doublet and have two components. To ensure a posteriori that a massless photon couples to the electrical charge, its weak hypercharge must be. The Higgs doublet can accordingly be described as ${\displaystyle Y_{\phi }=1}$

${\displaystyle \phi ={\begin{pmatrix}\phi ^{+}\\\phi ^{0}\end{pmatrix}}}$

write, where the superscripts denote the electrical charge that follows from the weak hypercharge of the Higgs and the weak isospin according to . Since, due to the electrical neutrality of the universe, only the vacuum expectation value of an electrically neutral field can differ from zero, it follows that the Higgs field as ${\displaystyle Q=Y_{\phi }/2+T^{3}}$

${\displaystyle \phi ={\frac {1}{\sqrt {2}}}{\begin{pmatrix}0\\v+h\end{pmatrix}}}$

must be written. Using a suitable local transformation, every Higgs doublet can be converted into this form with real and ( unitary calibration ). ${\displaystyle SU(2)\otimes U(1)}$ ${\displaystyle v}$ ${\displaystyle h}$

After the explicit insertion of the Pauli matrices and the replacement of the Higgs field in terms of the vacuum expectation value and , results for the mass terms ${\displaystyle \phi }$ ${\displaystyle h}$

${\displaystyle {\mathcal {L}}={\frac {v^{2}}{8}}\left[g^{2}(W_{1}^{2}+W_{2}^{2})+(gW_{3}-g'B)^{2}\right]+\dots }$.

In order to guarantee the correct electrical charge of the W bosons, one defines

${\displaystyle W^{\pm }={\frac {W_{1}\mp \mathrm {i} W_{2}}{\sqrt {2}}}}$.

Furthermore, since the observable particles can only be masses of their own , the second term in square brackets has to be reformulated as such. One finds these eigenstates as

${\displaystyle A={\frac {g'W_{3}+gB}{\sqrt {g^{2}+g'^{2}}}}\qquad Z={\frac {gW_{3}-g'B}{\sqrt {g^{2}+g'^{2}}}}}$.

Overall, the Lagrangian can be used

${\displaystyle {\mathcal {L}}={\frac {v^{2}}{8}}\left[g^{2}(W^{+})^{2}+g^{2}(W^{-})^{2}+(g^{2}+g'^{2})Z^{2}+0A^{2}\right]+\dots }$

sum up. So there are two equally heavy charged bosons with a mass , an uncharged massless boson and an uncharged boson with a mass . The Higgs mechanism not only explains why certain gauge bosons have a mass, but also provides an explanation why the Z boson is heavier than the W bosons. ${\displaystyle m_{W}={\frac {1}{2}}vg}$ ${\displaystyle m_{Z}={\frac {1}{2}}v{\sqrt {g^{2}+g'^{2}}}}$

Higgs mechanism and fermions

A generation of fermions

The mass term for Dirac fermions (fermions that are not their own antiparticles) has the form . There is a fermionic field and an overline denotes the Dirac adjoint with the zeroth Dirac matrix . In principle, such a term does not contradict the gauge invariance for fermionic fields. In the Standard Model, however, fields with left-handed chirality transform differently than those with right-handed (the symmetry group is explicit ). If you write the Lagrange density of a free fermion in terms of left- and right-handed fields, you get with ${\displaystyle m{\bar {\psi }}\psi }$ ${\displaystyle \psi }$ ${\displaystyle {\bar {\psi }}=\psi ^{\dagger }\gamma ^{0}}$ ${\displaystyle SU(2)_{L}\otimes U(1)_{Y}}$

${\displaystyle {\mathcal {L}}_{\text{Fermion}}=\mathrm {i} {\bar {\psi }}_{L}\gamma ^{\sigma }D_{\sigma }\psi _{L}+\mathrm {i} {\bar {\psi }}_{R}\gamma ^{\sigma }D_{\sigma }\psi _{R}-m\left({\bar {\psi }}_{L}\psi _{R}+{\bar {\psi }}_{R}\psi _{L}\right)}$

a term in which an independent transformation of left- and right-handed components violates the gauge invariance.

In order to grant the gauge invariance for fermions as well, instead of the explicit mass term, a Yukawa coupling is introduced between the fermion field and the Higgs field, so that a mass is also generated by the non-vanishing vacuum expectation value. It follows from the behavior of the various fields under the operations of the symmetry group that the terms

${\displaystyle {\mathcal {L}}_{\text{Yukawa}}=-\Lambda ^{d}({\bar {\psi }}_{L}\phi \psi _{R}+{\bar {\psi }}_{R}\phi ^{\dagger }\psi _{L})-\Lambda ^{u}({\bar {\psi }}_{L}{\tilde {\phi }}^{c}\psi _{R}+{\bar {\psi }}_{R}({\tilde {\phi }}^{c})^{\dagger }\psi _{L})}$

are gauge invariant. Where and are two coupling constants and with the second Pauli matrix . Is shown more clearly ${\displaystyle \Lambda ^{d}}$ ${\displaystyle \Lambda ^{u}}$ ${\displaystyle {\tilde {\phi }}^{c}=-\mathrm {i} \sigma _{2}\phi ^{*}}$ ${\displaystyle \sigma _{2}}$

${\displaystyle {\tilde {\phi }}^{c}={\frac {1}{\sqrt {2}}}{\begin{pmatrix}v+h\\0\end{pmatrix}}}$

in unitary calibration, so that the entries of the doublet are swapped.

If you insert - for quarks - the doublet as well as the singlets or , then one of the two terms in the Yukawa Lagrangian always disappears and you get ${\displaystyle \psi _{L}={\begin{pmatrix}u_{L}\\d_{L}\end{pmatrix}}}$ ${\displaystyle \psi _{R}=u_{R}}$ ${\displaystyle \psi _{R}=d_{R}}$

${\displaystyle {\mathcal {L}}_{\text{Yukawa}}=-{\frac {\Lambda ^{d}v}{\sqrt {2}}}({\bar {d}}_{R}d_{L}+{\bar {d}}_{L}d_{R})-{\frac {\Lambda ^{u}v}{\sqrt {2}}}({\bar {u}}_{R}u_{L}+{\bar {u}}_{L}u_{R})+{\text{Wechselwirkung}}}$

with the crowds and . ${\displaystyle m_{d}={\frac {\Lambda ^{d}v}{\sqrt {2}}}}$ ${\displaystyle m_{u}={\frac {\Lambda ^{u}v}{\sqrt {2}}}}$

For leptons , the left-handed doublet and the corresponding right -handed singlets are used analogously . It should be noted that in the standard model the right-handed neutrino singlet can not interact with any other particle, not even with itself ( sterile neutrino ) and its existence is therefore questionable. ${\displaystyle \psi _{L}={\begin{pmatrix}\nu _{L}\\e_{L}\end{pmatrix}}}$ ${\displaystyle \nu _{R}}$

Several generations

See also : CKM matrix

In the standard model, there are three generations of fermions that have identical quantum numbers with regard to transformations . In general, therefore, the coupling constants between the Higgs boson and the fermions are matrices that mix the different generations; each of these resulting terms is gauge invariant and therefore valid in the Lagrangian. These matrices give rise to mixed terms of the second order, analogous to that between the photon and the Z boson. Therefore the mass eigenstates of the fermions are not the eigenstates of the electroweak interaction. The transformation matrix between the different quark states is called the CKM matrix , that between leptons is called the MNS matrix . ${\displaystyle SU(2)_{L}\otimes U(1)_{Y}}$

The interaction states of the strong interaction , the additional unbroken symmetry of the Standard Model, are the mass eigenstates and not the eigenstates of the weak interaction. ${\displaystyle SU(3)}$

Relationship to astrophysics

Since the Higgs field does not couple to the massless light quanta (" photons ") and itself generates " mass ", a connection with the astrophysically interesting dark matter is obvious, because this matter is only "visible" due to its gravitational effect. In fact, at the end of 2009 , Marco Taoso and colleagues from CERN calculated that the Higgs field could become indirectly visible as a result of the annihilation of very heavy particles in connection with elementary particle reactions involving dark matter.

Popular scientific interpretation ("Alice, Bob and the Party")

As a popular scientific illustration of the Higgs mechanism taken from everyday life as a collective effect of the Higgs field, one often finds the appearance of a star, usually called "Alice", at a party: Before "Alice" enters the hall, the party guests are evenly distributed in the room. As soon as she enters, however, numerous guests run up to her, wanting autographs or small talk. As a result, “Alice” progresses much more slowly in this crowd of party guests than she actually could, so the interactions of the party guests with the star have the same effect in terms of progress as the star's additional body mass. The effect of the party guests on "Alice" is the same as one would get from a single male colleague ("Bob") who fascinates the female star herself.

In this illustration, the party guests generate the Higgs potential, "Alice" represents the calibration particle that gets mass. The Higgs field itself, including the “breaking of symmetry”, is represented by the guests, who move closer together to whisper about “Alice” and therefore hardly move around the room as a group. "Bob", who has the same effect as all of the party guests on "Alice", represents the Higgs boson. On “Bob” himself, the gathering of party guests is attractive; The fact that "Alice" is in it is noted by him, but is basically secondary ("it occurs in the Lagrange density"). He himself feels like “surplus” and is accordingly distant, difficult to stimulate and even more difficult to find.

Another way of displaying the Higgs boson compares this with a rumor, which also draws party guests together locally. The German physicist Harald Lesch gives various other popular scientific interpretations in an online interview.

literature

• Peter Higgs: Broken symmetries, massless particles and gauge fields. In: Physics Letters. Volume 12, 1964, pp. 132-133
• Peter Higgs: Broken symmetries and the masses of gauge bosons. In: Physical Review Letters. Volume 13, 1964, pp. 508-509
• Guralnik, Hagen, Kibble: Global conservation laws and massless particles. In: Physical Review Letters. Volume 13, 1964, pp. 585-587
• Englert, Brout: Broken symmetry and the mass of gauge vector mesons. In: Physical Review Letters. Volume 13, 1964, pp. 321-323
• Walter Greiner , Berndt Müller : Calibration theory of the weak interaction . 2nd edition, Harri Deutsch, 1995, pp. 133 ff, ISBN 3-8171-1427-3

Web links

• What is a Higgs particle? from the alpha-Centauri television series(approx. 15 minutes). First broadcast on May 25, 2005.
• David J. Miller: A quasi-political Explanation of the Higgs Boson
• Animation of the Higgs mechanism (Uni-Wuppertal)
• Guralnik: The History of the Guralnik, Hagen and Kibble development of the Theory of Spontaneous Symmetry Breaking and Gauge Particles. In: International Journal of Modern Physics A. Volume 24, 2009, pp. 2601-2627
• The three works by Brout and Englert or Higgs or Guralnik, Hagen and Kibble (Physical Review Letters, "Milestones" from 1964)

Individual evidence

1. ↑ ^{a b} Peter Higgs: Broken symmetries, massless particles and gauge fields. In: Physics Letters. Volume 12, 1964, pp. 132-133
2. ↑ Peter Higgs: Broken symmetries and the masses of gauge bosons. In: Physical Review Letters. Volume 13, 1964, pp. 508-509
3. ^ Englert, Brout: Broken Symmetry and the Mass of Gauge Vector Mesons . In: Physical Review Letters. Volume 13, 1964, pp. 321-323
4. ^ Guralnik, Hagen and Kibble: Global Conservation Laws and Massless Particles . In: Physical Review Letters. Volume 13, 1964, pp. 585-587
5. ↑ CERN publication rule. CERN, accessed April 16, 2018 . 
6. ↑ Englert-Brout-Higgs-Guralnik-Hagen-Kibble Mechanism on Scholarpedia
7. ^ Nobelprize.org: The Nobel Prize in Physics 2013 , accessed October 8, 2013.
8. ↑ Ph. Anderson: Plasmons, gauge invariance and mass. In: Physical Review. Volume 130, 1963, pp. 439-442
9. ^ TWB Kibble: Symmetry breaking in non-Abelian gauge theories . In: Phys. Rev. . 155, 1967, p. 1554. doi : 10.1103 / PhysRev.155.1554 .
10. ^ A. Salam: Weak and electromagnetic interactions . In: Proc. Nobel Symp . 8, 1968, pp. 367-377.
11. ^ SL Glashow: Partial symmetries of weak interactions . In: Nucl. Phys. . 22, 1961, p. 579. doi : 10.1016 / 0029-5582 (61) 90469-2 .
12. ^ S. Weinberg: A model of leptons . In: Phys. Rev. Lett. . 19, 1967, pp. 1264-1266. doi : 10.1103 / PhysRevLett.19.1264 .
13. ↑ Details can be found below.
14. ↑ DA Kirzhnits and AD Linde: Macroscopic Consequences of the Weinberg model . In: Physics Letters B . tape 42 , no. 4 , 1972, p. 471-474 (English).
15. ↑ Mikko Laine: Electroweak phase transition beyond the Standard Model . In: Strong and Electroweak Matter 2000 . 2001, p. 58-69 (English).
16. ↑ Taoso's proposal is fully visible in "Higgs in Space!"
17. ↑ According to this proposal, the interaction between the hypothetical so-called "WIMPs" (the "Weakly Interacting Massive Particles", which are supposed to form the dark matter) and the Higgs field mainly concerns the most massive gauge boson, the Z boson, 90 GeV / c ² ) and the most massive fermionic elementary particle, the “top” quark, 171 GeV / c ² ) of the standard model , which could implicitly make the Higgs boson, especially its mass, visible. See also the comment in physicsworld.com, Higgs could reveal itself in dark-matter collisions . ${\displaystyle M_{Z}\sim }$ ${\displaystyle M_{\,{\rm {T}}}\sim }$
18. ^ Comparison of the Higgs mechanism by David Miller: "Politics, Solid State and the Higg" s
19. ↑ Popular scientific presentation of the Higgs boson by DESY
20. ^ Süddeutsche.de: Harald Lesch on Higgs boson - "Nobody understands that" , July 6, 2012.