Mathematical structure of quantum mechanics

This article presents the mathematical structure of quantum mechanics .

Formulated by von Neumann

The essential foundations for the mathematically strict formulation of quantum mechanics were formulated in 1932 by John von Neumann . Accordingly, a physical system can generally be described by three essential components: its states , its observables and its dynamics (that is, its development over time). Von Neumann's postulates are presented here in a slightly updated form (Spin, Pauli principle, see below):

Postulates of Quantum Mechanics (Copenhagen Interpretation)

In the context of the Copenhagen interpretation , the quantum mechanical description of a system is based on the following postulates:

1. State: The state of a physical system at a point in time is defined by specifying a complex state vector belonging to the state space. Vectors which differ only by a factor different from 0 describe the same state. The state space of the system is a Hilbert space . ${\displaystyle t_{0}}$ ${\displaystyle {\mathcal {H}}}$ ${\displaystyle |\psi (t_{0})\rangle }$ ${\displaystyle c\in \mathbb {C} }$
2. Observable: Every quantity that can be physically “measured” is described by a Hermitian operator operating in the state space . This operator is called an observable and has a real spectrum with a complete so-called spectral family, consisting of a “discrete” part with eigenvectors and eigenvalues ​​(point spectrum) and a continuum. ${\displaystyle A}$ ${\displaystyle {\hat {A}}}$
3. Measurement result: The result of the measurement of a physical quantity can only be one of the eigenvalues ​​of the corresponding observable or, in the case of a continuous spectrum of the operator, a measurable set from the continuum. ${\displaystyle A}$ ${\displaystyle {\hat {A}}}$
4. Measurement probability in the case of a discrete nondegenerate spectrum: If the physical quantity is measured on a system in the state , the probability is to obtain the nondegenerate eigenvalue of the corresponding observable (with the associated eigenvector ) . Let and be standardized. ${\displaystyle A}$ ${\displaystyle |\psi \rangle }$ ${\displaystyle P(a_{n})}$ ${\displaystyle a_{n}}$ ${\displaystyle {\hat {A}}}$ ${\displaystyle |u_{n}\rangle }$ ${\displaystyle P(a_{n})=|\langle u_{n}|\psi \rangle |^{2}}$ ${\displaystyle \psi }$ ${\displaystyle u_{n}}$
5. The time development of the state vector is given by the Schrödinger equation : ${\displaystyle |\psi (t)\rangle }$

   ${\displaystyle \mathrm {i} \hbar {\frac {\partial }{\partial t}}|\psi (t)\rangle ={\hat {H}}(t)|\psi (t)\rangle }$,

where is the observable associated with the total energy of the system. ${\displaystyle {\hat {H}}(t)}$

In addition, there are statements about the spin and Pauli principle , which can only be justified in a relativistic expansion of quantum mechanics, but are already essential for non-relativistic quantum mechanics and, for example, decisively determine the periodic table of the elements .

Quantum mechanical states

In classical mechanics, the state of a physical system with degrees of freedom and its development over time are completely determined by specifying pairs of canonically conjugated variables . Because in quantum mechanics two corresponding conjugate observables cannot be determined at the same time as precisely as desired, the fundamental question arises to what extent a corresponding definition of the state of a quantum physical system makes sense. The fundamental approach within the framework of quantum mechanics, that a physical system can only be defined using simultaneously measurable observables, is one of its main differences from classical mechanics. A multitude of quantum physical phenomena can only be theoretically described through the consistent implementation of such a definition of the state. ${\displaystyle f}$ ${\displaystyle f}$ ${\displaystyle (q_{i},\,p_{i})}$

In the context of quantum mechanics, a physical state is defined via a maximum set of simultaneously measurable observables; in this context one speaks of a complete set of commuting observables (VSKO). Observables can take on very specific values ​​during a measurement, the respective spectrum of which generally depends on the system under consideration and on the respective observables. The respective possible measured values form the spectrum of the observables. They can be distributed both discretely and continuously. In the discrete case, they are called the eigenvalues ​​of the observables. For the sake of simplicity, it is usually assumed that the spectrum is purely discrete, although there are important observables whose spectrum is purely continuous (for example position and momentum operators). ${\displaystyle |\psi \rangle }$ ${\displaystyle \{O_{1},O_{2},\dotsc ,O_{f}\}}$ ${\displaystyle n}$

The states associated with the eigenvalues are called the eigenstates of the observables. In the case of a continuous spectrum, one speaks of generalized eigenfunctions. These are distributions such as the Dirac function or monochromatic, plane waves, which actually do not belong to the state space because they cannot be square-integrated, but from which permitted states can be superposed through integration (wave packet formation, cf. generalized Fourier series ). Unless otherwise stated, only the discrete case is considered below. ${\displaystyle |n\rangle }$ ${\displaystyle \psi _{\mathbf {r} '}(\mathbf {r} ):=\delta (\mathbf {r} -\mathbf {r} ')}$ ${\displaystyle \psi _{\mathbf {p} }(\mathbf {r} ):={\frac {1}{(2\pi \hbar )^{3/2}}}\,e^{{\rm {i}}\mathbf {p} \cdot \mathbf {r} /\hbar }}$

Since measurements regarding the observables of a VSKO do not influence each other, a given quantum physical system can be prepared to a state that is eigenstate for each of the observables of the VSKO by using suitable filters :

${\displaystyle |\psi \rangle =\left|n^{(O_{1})},\ldots ,n^{(O_{f})}\right\rangle .}$

Fig. 1: Schematic representation of a 3-dimensional subspace of the i. A. infinite-dimensional Hilbert space. The state is built up from a linear combination of the eigenstates of a VSKO. The coordinates are the probability amplitudes.

Such a state is often called a pure quantum state . It is defined and maximally determined via its associated eigenvalues.

It should be emphasized that a quantum state prepared in this way - in contrast to the state of a classical system - does not determine all the measurable properties of the physical system! For observables that are incompatible with the VSKO, only a certain probability can be given for each of their eigenvalues, with which this results from a measurement; the measurement result is in any case an eigenvalue of the observable. This indeterminacy in principle depends on the above. Uncertainty relation together. It is one of the most important statements of quantum mechanics and is at the same time the cause of many rejection of it.

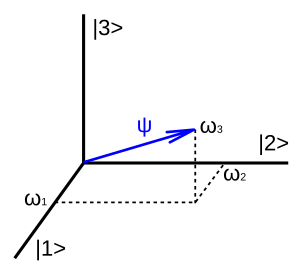
For a given quantum physical system, the eigenstates belonging to the eigenvalues ​​of an observable form a linear state space  - mathematically a so-called Hilbert space . This represents the totality of all possible states of the system and thus generally has an infinite number of dimensions even in simple systems such as the quantum mechanical harmonic oscillator ; however, one can get by with countable-infinite-dimensional spaces (separable Hilbert spaces). This does not contradict the fact that there are also measured quantities with a continuous spectrum. In any case, it is essential that a linear superposition of several eigenstates is again part of the state space, even if the superimposing state is not an eigenstate of the observables. In this context, one speaks of a superposition of several states. This property is comparable to that of vectors in a plane, the superposition of which is also a vector in the plane. ${\displaystyle {\mathcal {H}}}$

A simple example of a quantum system is the two-state system , see the article Qubit .

Statistical statements of quantum mechanics

Fig. 2: Probabilities of discrete measured values ​​of the observables , expected value and standard deviation. ${\displaystyle O}$

From the decomposition of the state into a linear combination of the orthonormal eigenstates of the observables, the square of the magnitude of the corresponding pre-factor results in a measure of the probability of measuring the eigenvalue in such a superimposed state, i.e. encountering the system in its eigenstate . The coefficients are therefore referred to as “probability amplitudes” for the measured values . They can be calculated as a projection (= scalar product) of the respective eigenstate (see Fig. 2): ${\displaystyle |n_{i}\rangle }$ ${\displaystyle |\omega _{i}|^{2}}$ ${\displaystyle n_{i}}$ ${\displaystyle |n_{i}\rangle }$ ${\displaystyle \omega _{i}}$ ${\displaystyle n_{i}}$ ${\displaystyle |\psi \rangle }$ ${\displaystyle |n_{i}\rangle }$

${\displaystyle \omega _{i}=\langle n_{i}|\psi \rangle }$

Accordingly, if a measurement of an observable is carried out repeatedly, i. A. different measurement results, even if the system was always in the same state before the measurement. Exception: If the system was prepared in an eigenstate of an observable , further measurements of these observables each result in the same measured value. The statistical distributions of the measured values ​​can be determined experimentally by repeatedly performing measurements on identically prepared systems. This connection between the measurement protocol and the mathematical calculation of quantum mechanics is confirmed in all experiments. ${\displaystyle O}$ ${\displaystyle n_{i}}$

For the sake of simplicity, it is assumed here that one is dealing with a purely discrete spectrum.

Development over time

The dynamics of quantum states are described by different representations, the so-called "images", which can be converted into one another by redefining the operators and states and are therefore equivalent. The expectation values ​​of operators are the same for all images.

Schrodinger picture

In the Schrödinger picture , the dynamics result from the following consideration: The state is defined by a differentiable mapping of the parameterized time to the Hilbert space of states. When describing the state of the system at any given time , the so-called Schrödinger equation applies ${\displaystyle t}$ ${\displaystyle \left|\psi \left(t\right)\right\rangle }$ ${\displaystyle t}$

${\displaystyle \mathrm {i} \hbar {\frac {\partial }{\partial t}}\left|\psi _{S}(t)\right\rangle ={\hat {H}}_{S}(t)\left|\psi _{S}(t)\right\rangle ,}$

with as a tightly-defined self-adjoint operator, the Hamiltonian , the imaginary unit and the reduced Planck's constant . As an observable corresponds to the total energy of the system. In general, the Hamilton operator can be time dependent, e.g. B. when the system interacts with an electromagnetic field. ${\displaystyle {\hat {H}}_{S}}$ ${\displaystyle {\rm {i}}}$ ${\displaystyle \hbar }$ ${\displaystyle {\hat {H}}_{S}}$

The time evolution of a state is given by the unitary time evolution operator ( is the time order operator , see below): ${\displaystyle {\hat {U}}(t,t_{0})}$ ${\displaystyle {\hat {\mathcal {T}}}}$

${\displaystyle |\psi _{S}(t)\rangle ={\hat {U}}(t,t_{0})|\psi _{S}(t_{0})\rangle ,}$

in which

${\displaystyle {\hat {U}}(t,t_{0})={\hat {\mathcal {T}}}\left[\exp \left(-{\frac {\mathrm {i} }{\hbar }}\int _{t_{0}}^{t}{\hat {H}}_{\text{S}}(t^{\prime })dt^{\prime }\right)\right]\quad \left(\,{\overset {{\frac {\partial {\hat {H}}_{\text{S}}}{\partial t}}=0}{=}}\exp \left(-{\frac {\mathrm {i} }{\hbar }}{\hat {H}}_{\text{S}}\cdot (t-t_{0})\right)\,\right).}$

The time ordering operator ensures that operator products of the form are rearranged in the case of non-interchangeability in such a way that applies. That results in a causal chain; right cause, left effect. ${\displaystyle {\hat {\mathcal {T}}}}$ ${\displaystyle {\hat {A}}_{1}(t_{1})\cdot {\hat {A}}_{2}(t_{2})\cdot \dots \cdot {\hat {A}}_{n}(t_{n})}$ ${\displaystyle \to {\hat {A}}_{i_{1}}(t_{i_{1}})\cdot {\hat {A}}_{i_{2}}(t_{i_{2}})\cdot \dots \cdot {\hat {A}}_{i_{n}}(t_{i_{n}}),}$ ${\displaystyle t_{i_{1}}\geq t_{i_{2}}\geq \dots \geq t_{i_{n}}}$

Heisenberg picture

In the Heisenberg picture of quantum mechanics, instead of changes in the states over time, which remain constant in this picture, the time dependency is described by time-dependent operators for the observables. For the time-dependent Heisenberg operators, the differential equation ( Heisenberg's equation of motion ) results in ${\displaystyle t\geq t_{0}}$

${\displaystyle {\frac {\mathrm {d} {\hat {A}}_{\rm {H}}(t)}{\mathrm {d} t}}={\frac {\mathrm {i} }{\hbar }}\left[{\hat {H}}_{H}(t),{\hat {A}}_{H}(t)\right]+{\hat {U}}^{\dagger }(t,t_{0}){\frac {\partial {\hat {A}}_{S}(t)}{\partial t}}{\hat {U}}(t,t_{0}),}$

where denotes the operator in the Schrödinger picture. With the (already known) time evolution operator , the states and operators in the Heisenberg picture are linked with those in the Schrödinger picture via the following relationships: ${\displaystyle {\hat {A}}_{S}(t)}$ ${\displaystyle {\hat {U}}(t,t_{0})}$

${\displaystyle |\psi _{\text{H}}\rangle ={\hat {U}}^{\dagger }(t,t_{0})|\psi _{\text{S}}(t)\rangle =|\psi _{\text{S}}(t_{0})\rangle ={\text{const}}_{\,t}\quad \,(\,\Rightarrow \,{\frac {\mathrm {d} }{\mathrm {d} t}}|\psi _{\text{H}}\rangle \equiv 0\,)}$

${\displaystyle {\hat {A}}_{\text{H}}(t)={\hat {U}}^{\dagger }(t,t_{0})\,{\hat {A}}_{\rm {\text{S}}}(t)\,{\hat {U}}(t,t_{0})\quad \,(\,\Rightarrow \,{\hat {A}}_{H}(t_{0})\equiv {\hat {A}}_{\text{S}}(t_{0})\,)}$

Dirac image

In the Dirac or interaction picture, states and operators are generally time-dependent. In the Schrödinger picture, let the Hamilton operator be composed of a time-independent and a time-dependent Hermitian operator:

${\displaystyle {\hat {H}}_{S}(t)={\hat {H}}_{S}^{(0)}+{\hat {V}}_{S}(t)}$

In the interaction picture, one uses only the similarity transformation with the observables and the correspondingly formed expression for the states ${\displaystyle e^{\pm {\frac {\rm {i}}{\hbar }}(t-t_{0}){\hat {H}}_{S}^{(0)}}}$

${\displaystyle {\hat {V}}_{\text{I}}(t)\,:=e^{{\rm {i}}(t-t_{0}){\hat {H}}_{S}^{(0)}/\hbar }{\hat {V}}_{S}(t)\,e^{-{\rm {i}}(t-t_{0}){\hat {H}}_{S}^{(0)}/\hbar }}$.

From this it follows in particular . ${\displaystyle {\hat {H}}_{I}^{(0)}={\hat {H}}_{S}^{(0)}}$

The interaction picture is most useful when the temporal development of the observables can be solved exactly (that is, when "trivial" is), so that all mathematical complications remain limited to the temporal development of the states. For this reason, the Hamilton operator for the operators is called the "free Hamiltonian" and the Hamilton operator for the states is called the "interaction Hamiltonian". The dynamic development is now described by the following two equations: ${\displaystyle {\hat {H}}_{S}^{(0)}}$

• firstly the equation of state (see Schrödinger equation)

${\displaystyle \mathrm {i} \hbar {\frac {\partial }{\partial t}}\left|\psi _{\text{I}}(t)\right\rangle ={\hat {V}}_{\text{I}}(t)\left|\psi _{\text{I}}(t)\right\rangle }$

• and secondly the operator equation (see Heisenberg's equation of motion)

${\displaystyle {\frac {\mathrm {d} A_{\text{I}}(t)}{\mathrm {d} t}}={\frac {\mathrm {i} }{\hbar }}\left[H_{\text{I}}^{(0)},A_{\text{I}}(t)\right]}$

With the time evolution operator of the time-independent sub-problem

${\displaystyle {\hat {U}}^{(0)}=\exp \left(-{\frac {\mathrm {i} }{\hbar }}{\hat {H}}_{S}^{(0)}\cdot (t-t_{0})\right)}$

states and operators in the Dirac picture are linked with those in the Schrödinger picture via the following relationships:

${\displaystyle |\psi _{\text{I}}(t)\rangle ={\hat {U}}^{(0)\dagger }(t,t_{0})|\psi _{\text{S}}(t)\rangle \quad \,(\,\Rightarrow \,|\psi _{\text{I}}(t_{0})\rangle \equiv |\psi _{\text{S}}(t_{0})\rangle \,)}$

${\displaystyle {\hat {A}}_{\text{I}}(t)={\hat {U}}^{(0)\dagger }(t,t_{0})\,{\hat {A}}_{\rm {\text{S}}}(t)\,{\hat {U}}^{(0)}(t,t_{0})\quad (\,\Rightarrow \,{\hat {A}}_{\text{I}}(t_{0})\equiv {\hat {A}}_{\text{S}}(t_{0})\,)}$

Remarks

At the point in time, the operators and states of all images match: ${\displaystyle t_{0}}$

${\displaystyle {\hat {A}}_{\text{I}}(t_{0})={\hat {A}}_{\text{H}}(t_{0})={\hat {A}}_{\text{S}}(t_{0})}$

${\displaystyle |\psi _{\text{I}}(t_{0})\rangle =|\psi _{\text{H}}(t_{0})\rangle =|\psi _{\text{S}}(t_{0})\rangle }$

The Heisenberg picture corresponds to classical Hamilton mechanics (for example, the commutators of quantum mechanics correspond to the classical Poisson brackets ). Physically, however, the Schrödinger picture is more intuitive. The Dirac picture is often used in perturbation theory - especially in quantum field theory and many-particle physics . ${\displaystyle {\frac {\mathrm {i} }{\hbar }}[{\hat {A}},{\hat {B}}]}$

Some wave functions form probability distributions that do not change over time. Many systems, which in classical mechanics have to be described with a dynamic time behavior, show such “static” wave functions in the quantum mechanical description. For example, a single electron in an atom in the ground state is described by a circular trajectory around the atomic nucleus , while in quantum mechanics it is described by a static, spherically symmetric wave function that surrounds the atomic nucleus. (Note that only the smallest angular momentum states, the “s” waves, are spherically symmetric).

The Schrödinger equation, like the closely related Heisenberg equation and the equations of the interaction image, is a partial differential equation that can only be solved analytically for a few model systems (the most important examples include the quantum mechanical harmonic oscillator and the electron in the Coulomb potential). Even the electronic structure of the helium atom, which has only one more electron than hydrogen, can no longer be calculated analytically. However, there are a number of different techniques for computing approximate solutions. One example is the perturbation theory already mentioned , in which existing analytical solutions of simplified model systems are used as a starting point for calculating more complex models. This method is particularly successful when the interactions of the complex model can be formulated as "small" disturbances of the simple model system. Another method is the so-called “semiclassical approximation”, which can be applied to systems that have only small quantum effects. The effects caused by quantum mechanics can then be calculated assuming classical movement trajectories. This approach is used, for example, in research into quantum chaos .

Spin

In addition to their other properties, many particles have a kind of intrinsic angular momentum, the spin , for which there is no equivalent in classical physics. The spin is in units of quantized integer or half-integer, in the coordinate representation, therefore, does not apply , but where, which often is referred to, one of the following discrete values: . ${\displaystyle \hbar }$ ${\displaystyle \psi =\psi (\mathbf {r} )}$ ${\displaystyle \psi =\psi (\mathbf {r} ,m_{s})}$ ${\displaystyle m_{s}}$ ${\displaystyle \sigma }$ ${\displaystyle m_{s}\in \{-S,-(S-1),\dotsc ,+(S-1),+S\}}$

A distinction is made between bosons ( ) and fermions ( ). ${\displaystyle S\in \{0,1,2,\dotsc \}}$ ${\displaystyle S\in \{{\frac {1}{2}},{\frac {3}{2}},{\frac {5}{2}},\dotsc \}}$

Pauli principle

Linked to this is the so-called Pauli principle for systems made up of identical particles , which states, for example in the position representation, that when two of the particles ,, are interchanged , the following permutation behavior must apply to the -particle wave function: ${\displaystyle N}$ ${\displaystyle N}$ ${\displaystyle i\leftrightarrow j}$ ${\displaystyle N}$

${\displaystyle \psi (\dotsc ;\,\mathbf {r} _{i},\sigma _{i};\,\dotsc ;\,\mathbf {r} _{j},\sigma _{j};\,\dotsc ){\stackrel {!}{=}}(-1)^{2S}\cdot \psi (\dotsc ;\,\mathbf {r} _{j},\sigma _{j};\,\dotsc ;\,\mathbf {r} _{i},\sigma _{i};\,\dotsc ),}$

that is, the prefactor +1 must result for bosons and −1 for fermions. In two spatial dimensions, one can be replaced by any complex number with the amount one (see Anyon ). In so-called supersymmetric theories, as discussed in high energy physics, the state would be a linear combination of a bosonic and a fermionic component. ${\displaystyle (-1)^{2S}}$

Electrons are fermions with ; Photons are bosons with . ${\displaystyle S=1/2}$ ${\displaystyle S=1}$

Newer formalisms

An alternative approach to calculating quantum mechanical systems is the path integral formalism by Richard Feynman , in which a quantum mechanical amplitude is represented as the sum of the probability amplitudes for all theoretically possible paths of a particle in its movement from an initial state to a target state. This formulation is the quantum mechanical analogue of the classical principle of action .

It is only recently that a more general mathematical description of observables using positive operator valued probability measures has emerged, which is hardly dealt with in traditional textbook literature. Operations on quantum systems are described very comprehensively and mathematically in the modern, but still little-known version of quantum mechanics using completely positive images . This theory generalizes both the unitary time evolution and the traditional von Neumann description of the change in a quantum system during a measurement described above. Concepts that are difficult to describe in the traditional picture, such as continuously running fuzzy measurements, fit easily into this newer description. The method of so-called C * algebras should also be mentioned here .

literature

• John von Neumann : Mathematical foundations of quantum mechanics. Springer, Berlin-Heidelberg 1996, second edition, ISBN 978-3-5405-9207-5 .
• Claude Cohen-Tannoudji : Quantum Mechanics. de Gruyter, 1999, ISBN 3-11-016458-2 .
• Wolfgang Nolting: Basic course Theoretical Physics 5/1 (Quantum Mechanics - Basics). Springer Spectrum, Berlin-Heidelberg 2013, eighth edition, ISBN 978-3-6422-5402-4 .
• Wolfgang Scherer: Mathematics of Quantum Informatics. Springer-Verlag, Berlin-Heidelberg 2016, ISBN 978-3-6624-9079-2 .

References and footnotes

1. ↑ The occurrence of the factor −1 in fermions can be related to the unusual rotational behavior of these particles by imagining, for example, that the interchanging of two identical fermions takes place in such a way that one particle runs on the lower semicircle from to and at the same time the other on the upper semicircle from to . Overall, this creates a 360 ° orbit, which implies a factor of −1 for fermions. ${\displaystyle P_{i}}$ ${\displaystyle P_{j}}$ ${\displaystyle P_{j}}$ ${\displaystyle P_{i}}$