Faraday's law of induction

Faraday's law of induction (or the law of electromagnetic induction) is a term which is (ambiguously) applied to two related, but different, laws in classical electrodynamics.

The primary version, which (for the purposes of this article) we will call Faraday's law of induction, states that the induced electromotive force in a closed loop of wire is directly proportional to the time rate of change of magnetic flux through the loop.

In the other formulation, which (for the purposes of this article) we will call the Maxwell-Faraday equation is best known as one of the four modern Maxwell's equations.

(Note: this terminology is non-standard, and not used universally.)

The Maxwell-Faraday equation refers to only one aspect of Faraday's law of induction — namely, that a changing B-field induces an E-field with a nonzero curl, that is, an E-field that is not conservative – it has a nonzero path integral around a closed loop.

The Maxwell-Faraday equation makes no reference to EMF, and is better thought of as an equation than a law because it is one of the set of Maxwell's equations, and because its prediction of an EMF is possible only with the supplementary aid of the Lorentz force law, and even then is more restricted in application than Faraday's law of induction.

Faraday's law of induction

Moving a permanent magnet near a conductor (such as a metal wire) produces a voltage in that conductor. The resulting voltage is proportional to the speed of movement: moving the magnet twice as fast produces twice the voltage.

For a tightly-wound coil of wire, composed of N loops with the same area, Faraday's law of induction states that

${\mathcal {E}}=-N{{d\Phi _{B}} \over dt}$

where

${\mathcal {E}}$ is the electromotive force (emf) in volts

N is the number of turns of wire

Φ_B is the magnetic flux in webers through a single loop. The direction of the electromotive force (the negative sign in the above formula) was first given by Lenz's law.

Example

Figure 1: Rectangular wire loop in magnetic field B moving along x-axis at velocity v.

Consider the case in Figure 1 of a rectangular loop of wire in the xy-plane translated in the x-direction at velocity v. Thus, the center of the loop at x_C satisfies v = dx_C / dt. The loop has length ℓ in the y-direction and width w in the x-direction. A time-independent but spatially varying magnetic field B(x) points in the z-direction. The magnetic field on the left side is B( x_C − w / 2), and on the right side is B( x_C + w / 2). The electromotive force is to be found directly and by using Faraday's law above.

Lorentz force law method

A charge q in the wire on the left side of the loop experiences a force q v B(x_C − w / 2) leading to an EMF (work per unit charge) of v ℓ B(x_C − w / 2) along the length of the left side of the loop. On the right side of the loop the same argument shows the EMF to be v ℓ B(x_C + w / 2). The two EMF's oppose each other, both pushing positive charge toward the bottom of the loop. In the case where the B-field increases with position x, the force on the right side is largest, and the current will be clockwise: using the right-hand rule, the B-field generated by the current opposes the impressed field. The EMF driving the current must increase as we move counterclockwise (opposite to the current). Adding the EMF's in a counterclockwise tour of the loop we find

${\mathcal {E}}=v\ell [B(x_{C}+w/2)-B(x_{C}-w/2)]\ .$

Faraday's law method

At any position of the loop the magnetic flux through the loop is

$\Phi _{B}=\pm \int _{0}^{\ell }dy\int _{x_{C}-w/2}^{x_{C}+w/2}B(x)dx$

$=\pm \ell \int _{x_{C}-w/2}^{x_{C}+w/2}B(x)dx\ .$

The sign choice is decided by whether the normal to the surface points in the same direction as B, or in the opposite direction. If we take the normal to the surface as pointing in the same direction as the B-field of the induced current, this sign is negative. The time derivative of the flux is then (using the chain rule of differentiation):

${\frac {d\Phi _{B}}{dt}}=(-){\frac {d}{dx_{C}}}\left[\int _{0}^{\ell }dy\ \int _{x_{C}-w/2}^{x_{C}+w/2}dxB(x)\right]{\frac {dx_{C}}{dt}}\ ,$

$=(-)v\ell [B(x_{C}+w/2)-B(x_{C}-w/2)]\ ,$

(where v = dx_C / dt is the rate of motion of the loop in the x-direction ) leading to:

${\mathcal {E}}=-{\frac {d\Phi _{B}}{dt}}=v\ell [B(x_{C}+w/2)-B(x_{C}-w/2)]\ ,$

as before.

The equivalence of these two approaches is general and, depending on the example, one or the other method may prove more practical.

The Maxwell-Faraday equation

Figure 2: An illustration of Kelvin-Stokes theorem with surface Σ its boundary ∂Σ and orientation n set by the right-hand rule.

A changing magnetic field can create an electric field; this phenomenon is described by the Maxwell-Faraday equation:

$\nabla \times \mathbf {E} =-{\frac {\partial \mathbf {B} }{\partial t}}$

where:

$\nabla \times$ denotes curl

E is the electric field

B is the magnetic field

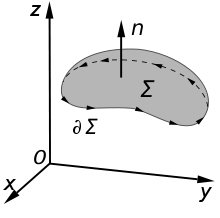
This is called the differential form of the Maxwell-Faraday equation; by Kelvin-Stokes theorem it can also be written in an integral form:

$\oint _{\partial \Sigma }\mathbf {E} \cdot d\mathbf {\ell } =-\ {\partial \over {\partial t}}\iint _{\Sigma }\mathbf {B} \cdot d\mathbf {A}$

where, as indicated in Figure 2:

Σ is a surface bounded by the closed contour ∂Σ; both Σ and ∂Σ are fixed, independent of time

E is the electric field,

dℓ is an infinitesimal vector element of the contour ∂Σ,

B is the magnetic field.

dA is an infinitesimal vector element of surface Σ , whose magnitude is the area of an infinitesimal patch of surface, and whose direction is orthogonal to that surface patch.

Both dℓ and dA have a sign ambiguity; to get the correct sign, the right-hand rule is used, as explained in the article Kelvin-Stokes theorem.

The integral around ∂Σ is called a path integral or line integral. The surface integral at the right-hand side of this equation is the explicit expression for the magnetic flux Φ_B through Σ.

Notice that a nonzero path integral for E is different from the behavior of the electric field generated by charges. A charge-generated E-field can be expressed as the gradient of a scalar field that is a solution to Poisson's equation, and has a zero path integral. See gradient theorem.

The integral equation is true for any path ∂Σ through space, and any surface Σ for which that path is a boundary. Note, however, that ∂Σ and Σ are understood not to vary in time in this formula; in other words, the equation also can be written:

$\oint _{\partial \Sigma }\mathbf {E} \cdot d\mathbf {\ell } =-\iint _{\Sigma }{\frac {\partial \mathbf {B} }{\partial t}}\cdot d\mathbf {A} .$

Notice that this equation makes no connection to EMF ${\overset {\mathcal {E}}{}}$,  and indeed cannot do so without introduction of the Lorentz force law to enable a calculation of work. See full discussion below.

Relation between two versions

In this section, Faraday's law of induction is derived from the Maxwell-Faraday equation plus the Lorentz force law.

First, the time derivative of the magnetic flux is found using Maxwell's equations. Take the example in Figure 2 of a surface Σ bounded by a curve ∂Σ. The magnetic flux through Σ is

$\Phi _{B}=\iint _{\Sigma }\mathbf {B} \cdot d\mathbf {A} \ .$

The total time derivative of the flux (that is, including all the time dependencies, a "d/dt" derivative) has two terms, one the time-differentiated integrand (that is, related to how the magnetic field changes in time) and the other related to the time-derivative of the boundary motion (for example, how a wire loop itself might move in time). Schematically, we might write

$-{\frac {d\Phi _{B}}{dt}}=-{\frac {d}{dt}}\iint _{\Sigma }\mathbf {B} \cdot d\mathbf {A} =$$-\iint _{\Sigma }{\frac {\partial \mathbf {B} }{\partial t}}\cdot d\mathbf {A} -{\frac {\partial }{\partial \Sigma }}\left[\iint _{\Sigma }\mathbf {B} \cdot d\mathbf {A} \right]{\frac {d\Sigma }{dt}}$

$=\oint _{\partial \Sigma }\mathbf {E} \cdot d\mathbf {\ell } -{\frac {\partial }{\partial \Sigma }}\left[\iint _{\Sigma }\mathbf {B} \cdot d\mathbf {A} \right]{\frac {d\Sigma }{dt}}$

where the last term is an informal way to write the contribution to the time derivative from the boundary motion (using the chain rule). A specific case of the second term is seen in the example above.

At this point, the right-hand side of the EMF version of Faraday's law has been found using the Maxwell-Faraday equation. Finding the left side, namely the EMF ${\stackrel {\mathcal {E}}{}}\$   (that is, the work required to bring one unit of charge around the loop) in Faraday's law, requires addition of the Lorentz force law to the Maxwell-Faraday equation, inasmuch as work is force × distance.

Like the above equation for −dΦ_B / dt, ${\stackrel {\mathcal {E}}{}}\$   also has two terms: one from the "electric force" q E, and one from the "magnetic force" q v × B in the Lorentz force law. The EMF due to the electric force around a closed path ∂Σ is

$\oint _{\partial \Sigma }\mathbf {E} \cdot d\mathbf {\ell } \ ,$

which matches precisely the first term in the −dΦ_B/dt expression above. The EMF around a closed path due to the magnetic force is more difficult to write down, but turns out to correspond exactly to the second term in the −dΦ_B/dt expression above.

This argument outlines how the Faraday's law can be derived from the Maxwell-Faraday equation by adding the Lorentz force law and including the "moving boundary" effects. However, this "extended" integral form is not what the term "Maxwell-Faraday equation" normally refers to.

Note that when B is constant in time, the EMF is purely from the magnetic force (∇ × E is zero). When ∂Σ is stationary, the EMF is purely from the electric force, (the v is zero in v × B) and Faraday's law reduces to the less general Maxwell-Faraday equation (in integral form) supplemented with the following connection to EMF valid for the stationary boundary case:

${\mathcal {E}}=\oint _{\partial \Sigma }\mathbf {E} \cdot d\mathbf {\ell } \$  [stationary boundary].

The converse derivation also can be done: it is also true that the Maxwell-Faraday equation can be derived starting with both Faraday's law and the Lorentz force law.

History

Faraday's law, along with the other laws of electromagnetism, was later incorporated into Maxwell's equations, unifying all of electromagnetism. However, in today's terminology, the term Maxwell's equations refers only to the four equations governing E ( r, t) and B ( r, t) with their relation to current J ( r, t) and charge ρ ( r, t). These laws are referred to individually as Gauss' law, Ampère's law (with Maxwell's addition of displacement current), the law of absence of magnetic monopoles (also called Gauss' law of magnetism), and Faraday's law of induction. The Lorentz force law is considered an additional law.

Faraday's law of induction is based on Michael Faraday's experiments in 1831. The effect was also discovered by Joseph Henry at about the same time, but Faraday published first.

See Maxwell's original discussion of electromotive force, or one of today's textbook treatments.

Lenz's law, formulated by German physicist Heinrich Lenz in 1834, gives the direction of the induced electromotive force and current resulting from electromagnetic induction.

Electrical generators

Figure 3: Faraday's disc electric generator. The disc rotates with angular rate ω, sweeping the conducting radius circularly in the static magnetic field B. The magnetic Lorentz force v × B drives the current along the conducting radius to the conducting rim, and from there the circuit completes through the lower brush and the axle supporting the disc. Thus, current is generated from mechanical motion.

Main article: electrical generator

The EMF generated by Faraday's law of induction is called an induced electromotive force (or induced EMF), and is the phenomenon underlying electrical generators. When a permanent magnet is rotated around a conductor, or vice versa, an electromotive force is created. If the wire is connected through an electrical load, current will flow, and thus electrical energy is generated. For example, see the Faraday's disc in Figure 3.

When the current is flowing through the wire loop, a magnetic field will be generated through Ampere's law. The electromagnet thus created will resist the rotation of the disc (Le Chatelier's principle). The energy required to keep the disc moving, despite this resistive force, is exactly equal to the electrical energy generated (plus energy wasted due to friction and other inefficiencies). This is why electrical generators can convert mechanical energy to electrical energy.

Although Faraday's law (EMF version) always describes the working of electrical generators, the detailed mechanism can differ in different cases. When the magnet is rotated around a stationary conductor, the changing magnetic field creates an electric field, as described by the the Maxwell-Faraday equation, and that electric field pushes the charges through the wire. On the other hand, when the magnet is stationary and the conductor is rotated, the moving charges experience a magnetic force (as described by the Lorentz force law), and this magnetic force pushes the charges through the wire. (This case is also called motional EMF.)

Electrical transformers

Main article: transformer

The "induced EMF" caused by Faraday's law is also responsible for electrical transformers. When the electric current in a loop of wire changes, the changing current creates a changing magnetic field. A second wire in reach of this magnetic field will experience this change in magnetic field as a change in its coupled magnetic flux. Therefore, an electromotive force is set up in the second loop. If the two ends of this loop are connected through an electrical load, current will flow.

Magnetic flow meter

Faraday's law is used for measuring the flow of electrically conductive liquids and slurries. Such instruments are called magnetic flow meters. The induced voltage U generated in the magnetic field B due to a conductive liquid moving at velocity v is thus given by:

$\mathbf {U} =BLv$,

where L is the distance between electrodes in the magnetic flow meter.

See also

• Michael Faraday
• Magnetic field
• Magnetic flux
• Ampère's law
• Lenz's law
• Lorentz force
• Stokes' theorem
• Vector calculus
• Moving magnet and conductor problem
• Crosstalk, an electrical interference caused by this law.
• Homopolar generator