Bending line

A bending line (also bending line , deflection line , elastic line ) is a mathematically easy to describe curve for the deformation of a straight beam under mechanical load .

Figure 4:
Course of the bending moment
on a beam
with a central force  F , shown
here as a point load  P ,
with the maximum bending moment  M at l / 2,
including the transverse force course  Q
and the bending line w

The equation of the elastic line is a part of the beam theory . It is used to determine the deflection of beams in the area of ​​the linear-elastic material behavior. The theory of the first order is used as a basis, i.e. H. the deformations caused by bending are assumed to be so small that they can be neglected when setting up the equation.

Modifications are required for the area of ​​non-linear elastic material behavior (see non-linear bar statics ).

Relation to the curvature of the beam

The relationship between the curvature of the beam and the bending line can be represented with a differential equation .

The curvature in an elastic straight beam is proportional to the bending moment ( cutting moment ) at the point . Taking into account Hooke's law of substances , one obtains ${\displaystyle \kappa (x)}$ ${\displaystyle M_{y}}$ ${\displaystyle x}$

${\displaystyle \kappa ={\frac {1}{r}}={\frac {M_{y}}{EI_{y}}}}$.

Are in it

• ${\displaystyle r}$the radius of curvature at the point ${\displaystyle x}$
• ${\displaystyle E}$the modulus of elasticity of the beam material
• ${\displaystyle I_{y}}$the axial geometrical moment of inertia of the beam cross-section.

With the purely geometric definition of a curve curvature, the differential equation for beam deflection follows : ${\displaystyle w(x)}$

${\displaystyle {\frac {w''(x)}{({1+(w'(x))^{2}})^{3/2}}}=-{\frac {M_{y}(x)}{EI_{y}}}}$.

The lines denote the derivation according to the longitudinal coordinate of the bar . ${\displaystyle x}$

In most practical cases the deflection is so small that it remains. Then the approximate differential equation is sufficient to determine the bending line : ${\displaystyle w}$ ${\displaystyle (w')^{2}\ll 1}$ ${\displaystyle w(x)}$

${\displaystyle w''(x)\approx -{\frac {M_{y}(x)}{EI_{y}}}}$

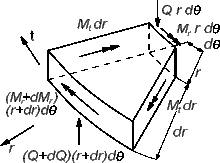
Differential relationships

In beam theory there are the following differential equations for the transverse components under the Bernoulli assumptions :

• ${\displaystyle {\frac {\mathrm {d} R(x)}{\mathrm {d} x}}=-q(x)}$
• ${\displaystyle {\frac {\mathrm {d} M(x)}{\mathrm {d} x}}=R(x)-N^{II}(x)\cdot \left[{\frac {\mathrm {d} w_{v}}{\mathrm {d} x}}+{\frac {\mathrm {d} w}{\mathrm {d} x}}\right]+m(x)}$
• ${\displaystyle {\frac {\mathrm {d} \varphi (x)}{\mathrm {d} x}}=-\left[{\frac {M(x)}{E\cdot I(x)}}+\kappa ^{e}(x)\right]}$
• ${\displaystyle {\frac {\mathrm {d} w(x)}{\mathrm {d} x}}=\varphi (x)+{\frac {V(x)}{G{\tilde {A}}(x)}}}$

With

• the running coordinate along the beam axis ${\displaystyle x}$
• the modulus of elasticity ${\displaystyle E}$
• the shear modulus (term does not appear in the differential equations in rigid theory) ${\displaystyle G}$
• the geometrical moment of inertia  I (x)
• ${\displaystyle R(x)}$the transverse force ( applies in the first order theory ) ${\displaystyle R(x)=V(x)}$
• ${\displaystyle V(x)}$the shear force
• ${\displaystyle N^{II}(x)}$the normal force according to the theory of the second order (in the theory of the first order this term does not appear in the differential equation)
• ${\displaystyle q(x)}$ the uniform load (transverse load per unit length)
• ${\displaystyle M(x)}$the bending moment
• ${\displaystyle m(x)}$ the insertion torque (bending load per unit length)
• ${\displaystyle \varphi (x)}$ the twist
• ${\displaystyle \kappa ^{e}(x)}$ the impressed curvature
• ${\displaystyle w(x)}$ load due to deflection
• ${\displaystyle w_{v}(x)}$ pre-deformation due to deflection
• ${\displaystyle {\tilde {A}}(x)}$ the shear area (term does not appear in the rigid theory).

These differential equations thus provide a relationship between the deflection and the bending moment in the beam. This leads to three equations for which there is a relationship between the deflection and the sectional loads in the beam (bending moment and shear force) as well as the external surface load (the coordinate is counted along the beam axis, the bending occurs around the coordinate axis , the coordinate runs in the direction of the transverse force.): ${\displaystyle w}$ ${\displaystyle M_{y}(x)}$ ${\displaystyle q_{z}(x)}$ ${\displaystyle x}$ ${\displaystyle y}$ ${\displaystyle z}$

${\displaystyle {\begin{alignedat}{2}&EI_{y}\,w''(x)\approx EI_{y}\,\kappa (x)&&=-M_{y}(x)\\(&EI_{y}\,w''(x))'&&=-Q_{z}(x)\\(&EI_{y}\,w''(x))''&&=q_{z}(x)\end{alignedat}}}$

The last equation of the fourth order is also called the Euler-Bernoulli equation.

So that the deflection can be calculated, the elastic modulus of the material must be known. Furthermore, the geometrical moment of inertia of the cross-section of the beam must be determined in advance and the course of the external line load or the course of the bending moment or shear force determined. The equation can then be integrated several times until the deflection is on one side. This results in several integration constants that can be determined by a corresponding number of boundary conditions . ${\displaystyle q_{z}(x)}$

The following summary shows the procedure when the curve of the bending moment has been determined in advance and the modulus of elasticity and the geometrical moment of inertia are constant over the length of the beam:

${\displaystyle EI_{y}\,w''(x)=-M_{y}(x)}$ ,

${\displaystyle EI_{y}\,w'(x)=-\int M_{y}(x)\mathrm {\,} dx+C_{1}}$ ,

${\displaystyle EI_{y}\,w(x)=-\int \int M_{y}(x)\mathrm {\,} dx^{2}+xC_{1}+C_{2}}$.

The two unknown constants and result . These can now be determined by two boundary conditions. For example, applies to a support at the location of which can hold a lateral force: . For a support on the site , which can hold a moment, then: . ${\displaystyle C_{1}}$ ${\displaystyle C_{2}}$ ${\displaystyle x=a}$ ${\displaystyle w(a)=0}$ ${\displaystyle x=b}$ ${\displaystyle w'(b)=0}$

If the beam is subjected to a line load, the bending moment curve can be found as follows: ${\displaystyle q(x)}$

${\displaystyle EI_{y}\,w''''(x)=q(x)}$ ,

${\displaystyle EI_{y}\,w'''(x)=\int q(x)\mathrm {\,} dx+C_{3}}$ ,

${\displaystyle EI_{y}\,w''(x)=\int \int q(x)\mathrm {\,} dx^{2}+xC_{3}+C_{4}=-M_{y}(x)}$ ,

determines the constants of integration and follows the previous list.

Circular membrane

Half circular membrane

Infinitesimal membrane element

In the case of a circular membrane, the formulas from bar theory are often used in simplified form. Assuming a homogeneous membrane, a simple bending line is then calculated for rotationally symmetrical forces. So just a cross section of the membrane.

With the tangential and radial bending moment and neglecting higher order differentials the moment equation results ${\displaystyle M_{t}}$ ${\displaystyle M_{r}}$

${\displaystyle M_{r}+{\frac {\mathrm {d} M_{r}}{\mathrm {d} r}}\cdot r-M_{t}+Q\cdot r=0.}$

The bending moments can be specified using the Poisson's ratio: ${\displaystyle \mu }$

${\displaystyle M_{r}=-D\left({\frac {\mathrm {d} ^{2}w}{\mathrm {d} r^{2}}}+{\frac {\mu }{r}}{\frac {\mathrm {d} w}{\mathrm {d} r}}\right)}$

${\displaystyle M_{t}=-D\left(\mu {\frac {\mathrm {d} ^{2}w}{\mathrm {d} r^{2}}}+{\frac {1}{r}}{\frac {\mathrm {d} w}{\mathrm {d} r}}\right)}$

${\displaystyle D}$here is the moment of resistance extending over the elastic modulus of the membrane thickness can be described as follows: ${\displaystyle E_{M}}$ ${\displaystyle d}$

${\displaystyle D={\frac {E_{M}\cdot d^{3}}{12\cdot (1-\mu ^{2})}}}$

The bending line of a circular membrane then reads in differential form, neglecting small terms of higher order as well as tensile stresses (only permissible for small elongations):

${\displaystyle {\frac {\mathrm {d} ^{3}w}{\mathrm {d} r^{3}}}+{\frac {1}{r}}{\frac {\mathrm {d} ^{2}w}{\mathrm {d} r^{2}}}-{\frac {1}{r^{2}}}{\frac {\mathrm {d} w}{\mathrm {d} r}}={\frac {Q}{D}}}$

Web links

Wikibooks: The Physics of Punching / Bending the Cane  - Learning and Teaching Materials

• The bending line (web archive) . Retrieved June 5, 2019.
• To the bending line (PDF; 116 kB)

Individual evidence

1. ^ Otto Lueger : Lexicon of the entire technology . 1904
2. ↑ Heinz Parkus : Mechanics of the solid body . Springer-Verlag, Vienna 1966, ISBN 3-211-80777-2
3. ↑ ^{a b} Bernhard Pichler, Josef Eberhardsteiner: Structural Analysis VO - LVA-Nr . 202.065 . Ed .: E202 Institute for Mechanics of Materials and Structures - Faculty of Civil Engineering, TU Vienna. SS 2017 edition. TU Verlag, Vienna 2017, ISBN 978-3-903024-41-0 , 10. Solving the linear differential equations in linear rod theory (516 pp., Tuverlag.at - first edition: 2012). Structural Analysis VO - LVA-Nr. 202.065 ( Memento of the original from July 17, 2017 in the Internet Archive ) Info: The archive link was inserted automatically and has not yet been checked. Please check the original and archive link according to the instructions and then remove this notice.  
4. ↑ Herbert Mang , Günter Hofstetter: Strength theory. 3. Edition. Springer, Berlin / Heidelberg 2008, ISBN 978-3-211-72453-8 , page 228
5. ↑ Herbert Mang , Günter Hofstetter: Strength theory. 3. Edition. Springer, Berlin / Heidelberg 2008, ISBN 978-3-211-72453-8 , page 176
6. ↑ ^{a b c d} Bernhard Pichler: 202.068 structural analysis 2 . WS2013 edition. Vienna 2013, VO_06_ThIIO_Uebertragungsbeektiven ( online platform of the Vienna University of Technology ).
7. ↑ ^{a b c} Bernhard Pichler, Josef Eberhardsteiner: Structural Analysis VO - LVA-Nr . 202.065 . SS2016 edition. TU Verlag, Vienna 2016, ISBN 978-3-903024-17-5 , linear bar theory of planar bar structures (520 pages, graphic center at the Technical University of Vienna [accessed on January 14, 2017]). Grafisches Zentrum at the Technical University of Vienna ( Memento of the original from March 13, 2016 in the Internet Archive ) Info: The archive link was inserted automatically and has not yet been checked. Please check the original and archive link according to the instructions and then remove this notice.