Fabry-Perot interferometer
The Fabry-Pérot interferometer , also known as the Pérot-Fabry interferometer , was developed in 1897 by the French physicists Charles Fabry and Alfred Pérot . It is an optical resonator made up of two partially transparent mirrors . If the mirror spacing cannot be changed (for example glass with vapor-deposited mirrors), these structures are also used as a measuring standard and are then referred to as Fabry-Perot etalon . An incoming light beam is only guided ( transmitted ) through this structure if it fulfills its resonance condition.
This allows the Fabry-Pérot interferometer u. a. Use as an optical filter that filters out a narrowband spectrum from broadband radiation. Mirror shifts also make it possible to adjust the spectral properties of the transmitted radiation. The transmission behavior can be calculated with the Airy formula .
Mode of action
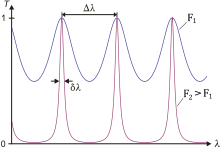
The Fabry-Pérot interferometer consists of two partially reflecting mirrors of high reflectivity , which together form an optical resonator . The transmission spectrum of this arrangement shows narrow transmission maxima for wavelengths which meet the resonance condition, while other spectral ranges are almost completely extinguished in the transmission. This happens through constructive or destructive interference of the partial beams.
The distance between the transmission maxima is called the free spectral range of the resonator. The frequency spacing depends on the mirror spacing and the refractive index :
The finesse is used to characterize the resonator. It is defined as the ratio between the free spectral range and the half width of a single maximum:
- .
An alternative measure is the finesse coefficient , which is determined by
is defined.
The greater the finesse, the more bundles of rays interfere with one another and the sharper the interference rings are. The simplest Fabry-Pérot interferometers achieve finesse of approximately in visible light . With high reflectivities of the mirrors and low attenuation in the resonator, the finesse takes on high values:
With dielectric thin film coverings and curved mirrors, finesse can be achieved.
With increasing finesse, the intensity or field strength of the light waves within the interferometer or resonator increases to values that are significantly higher than those of the light passing through. This fact must be taken into account in applications where performance is paramount (e.g. with laser resonators and modulators ).
The transmitted intensity is calculated as follows:
The resonance maxima are the longitudinal modes of a laser . Depending on its gain bandwidth, it can oscillate or “laser” in one or more of these modes.
Diameter of the interference fringes

If one with (1) used in (2) concerns with an optical path difference between the reflected beams. This gives the maximum order of the rings for a solid :
The innermost ring belongs to the order , so with (4) and (3) the angle is given. The diameter of the innermost ring is then calculated from this, if one takes into account that the maximum angle of incidence of the light rays is assumed to be 0 (perpendicular incidence). So the diameter of the innermost ring is given by:
Applications
The Fabry-Pérot interferometer is used:
- in spectroscopy as a tunable interference filter or for calibrating an unknown or non-linear frequency scale.
- in a modified form in research as a Virtually Imaged Phased Array for spectrometric applications or in communications engineering for wavelength division multiplexing
- as a mechanical modulator for monochromatic radiation, for example a CO 2 laser at a wavelength of 10.6 µm (modulated beam power up to over 100 watts)
- as a laser resonator
- in astronomy : in the H-alpha telescope for solar observation
- as a gravitational wave detector , s. KAGRA
literature
- Werner Lauterborn , Thomas Kurz: Coherent Optics - Fundamentals and Applications. Springer, 2002, ISBN 3-540-43933-1 .
- Wolfgang Zinth, Ursula Zinth: Optics - light rays, waves, photons. de Gruyter Studium, 2018, ISBN 978-3-11-049501-0 .
Individual evidence
- ^ MG Tarallo, N. Poli, M. Schioppo, D. Sutyrin, GM Tino: A high-stability semiconductor laser system for a 88Sr-based optical lattice clock . In: Applied Physics B . tape 103 , no. 1 , 2011, p. 17-25 , doi : 10.1007 / s00340-010-4232-2 .