Hill sphere

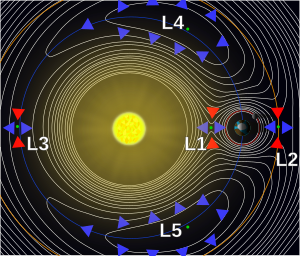
from Wikipedia, the free encyclopedia
Contour plot of the effective potential of the system of earth and sun. The proportions do not correspond to reality. The Hill spheres are the roughly circular lines around the two large masses that touch the Lagrangian points L1 and L2.

The hill sphere , also hill space , is the name given to the surroundings of a body in which its gravitational force is more effective than that of another, massive body that it orbits. The roughly spherical area was named after the mathematician George William Hill , who worked in theoretical astronomy . His work was based mainly on the writings of Édouard Roche .

parameter

The outer boundary of the Hill sphere depends on:

  • the gravitational force caused by the central body,
  • the gravitational force caused by the orbiting body and
  • the centrifugal force in a reference system that moves along with the orbiting body .

Within the Hill sphere, the sum of these three forces is directed towards the orbiting body. The limit of the Hill sphere, the Hill radius , corresponds to the distance to the first or second Lagrange point :

in which

  • a the distance of the centers of mass of the two bodies,
  • m is the mass of the orbiting body and
  • M is the mass of the central body.

Track stability

The orbit of a small body ("moon"), which is located within the Hill sphere of the object ("planet") running around the central body ("sun"), is extremely unstable in the outer area of ​​the sphere, so that it becomes the Hill - Sphere is very likely to leave in a short time. Only within a stability zone can the orbit of the "moon" be represented meaningfully as a disturbed elliptical orbit with temporally variable orbit elements . The size of this zone of stable orbits depends on the orbital direction of the "moon":

  • for prograde orbits, i.e. those that have the same direction of rotation as the “planet” orbits the “sun”, it extends up to a distance of almost 50% of the Hill radius;
  • for retrograde orbits the limit is a little further out at almost 70% of the Hill radius.

Examples

Hill radius of the planets and three dwarf planets of the solar system

For the earth with a mass of m = 5.97 · 10 24  kg in orbit around the sun with a mass M = 1.99 · 10 30  kg at a distance of a = 1 AU = 149.6 · 10 6  km a Hill radius of approximately 1.5 · 10 6  km = 0.01 AU.

Since the moon is approximately 0.37 · 10 6  km from Earth, it is well within the Hill sphere.

The Hill sphere of Jupiter has a mean radius of 0.35  astronomical units . This makes it almost as big as the large semi-major axis of Mercury and takes from the Earth seen more than fifteen times the full moon diameter one. The outermost known Jupiter moon S / 2003 J 2 has a large semi-axis, which corresponds to almost 60% of the Hill radius, and orbits the planet in a retrograde manner . Its orbit point furthest from Jupiter, the apoapsis , is at 68% of the Hill radius, however, at the extreme edge of the stability zone. The outermost known moon, which orbits the planet prograd, is Carpo , whose semi-axis makes up about 32% of the Hill radius and whose apoapsis is just under 48%. However, the orbit of this moon is unusual in many ways and may be unstable over long periods of time. The moons of the Himalia group , which have somewhat smaller orbital axes of about 22% of the Hill radius, reach about 30% of the Hill radius at the furthest orbital points. The most distant moons of Jupiter even reach up to 50% of the Hill radius; their orbits are almost retrograde. Examples here are the Carme group and the satellite (moon) S / 2003 J 2.

The hill spheres of the moons are usually very small and therefore also the probability that lunar satellites can orbit in stable orbits for a long time. This is most likely possible with large moons that move at a great distance from their planet . The largest Hill sphere among the natural satellites of the solar system has the Earth's moon with a radius of 9.2  Earth radii , followed by Saturn's moon Titan (Hill radius 8.2 Earth radii) and Jupiter's moon Callisto (7.9 Earth radii).

See also

Web links

supporting documents

  1. ^ A b Scott S. Sheppard, David Jewitt, Jan Kleyna: Ultra Deep Survey for Irregular Satellites of Uranus: Limits to Completeness . In: The Astronomical Journal . 129, 2005, pp. 518-523. arxiv : astro-ph / 0410059 . doi : 10.1086 / 426329 .