Holomorphic function
Holomorphism (from Gr. Ὅλος holos, "whole" and μορφή morphic, "form") is a property of certain complex-valued functions that are treated in function theory (a branch of mathematics ). A function with an open set is called holomorphic if it is differentiable from complex at every point . Such functions are also called regular in older literature in particular .
Even if the definition is analogous to real differentiability, function theory shows that holomorphism is a very strong property. Namely, it produces a multitude of phenomena that have no real counterpart. For example, every holomorphic function can be (continuously) differentiable as often as desired and can be expanded locally into a power series at every point .
Definitions
Let it be an open subset of the complex plane and a point of this subset. A function is called complex differentiable in the point , if the limit value
exists. It is then referred to as .
The function is holomorphic in point , if an environment of exists in is complex differentiable. If it is completely holomorphic, it is called holomorphic. If further , then one calls a whole function .
Explanations
Relationship between complex and real differentiability
is in a natural way a two-dimensional real vector space with the canonical base and so one can examine a function on an open set for its total differentiability in the sense of the multi-dimensional real analysis . As is well known, (total) means differentiable in if there is a -linear mapping such that
holds, where a function with
is. Now you can see that the function is precisely differentiable in complex if it is totally differentiable there and is even linear. The latter is a strong condition. It means that the representation matrix of with respect to the canonical base has the form
Has.
Cauchy-Riemann differential equations
If one breaks down a function into its real and imaginary parts with real functions , the total derivative has the Jacobi matrix as the representation matrix
Consequently, the function is complex differentiable if and only if it is real differentiable and for the Cauchy-Riemann differential equations
are fulfilled.
Equivalent properties of holomorphic functions of a variable
In an environment of a complex number, the following properties of complex functions are equivalent:
- The function can be differentiated in a complex manner.
- The function can be complexly differentiated as often as required.
- The real and imaginary parts satisfy the Cauchy-Riemann differential equations and are continuously real-differentiable at least once.
- The function can be developed into a complex power series .
- The function is continuous and the path integral of the function over any closed contractible path disappears.
- The function values inside a circular disk can be determined from the function values at the edge with the help of Cauchy's integral formula .
- The function is real differentiable and where the Cauchy-Riemann operator is, which is defined by .
Examples
Whole functions
Whole functions are completely holomorphic. Examples are
- any polynomial with coefficients ,
- the exponential function ,
- the trigonometric functions and ,
- the hyperbolic functions and .
Holomorphic, not whole functions
- Fractional rational functions are holomorphic except at the roots of their denominator polynomial. There they have isolated singularities . If these cannot be lifted, they are poles , so that there are examples of meromorphic functions .
- The logarithmic function can be expanded into a power series in all points and is therefore holomorphic on the set .
Nowhere holomorphic functions
In none of them are complex differentiable and therefore nowhere holomorphic, for example
- the amount function ,
- the projections on the real part or on the imaginary part ,
- the complex conjugation .
The function is only complex differentiable at that point , but not holomorphic there, since it cannot be differentiated in a whole environment of complex.
properties
Are complex differentiable at one point , so too , and . This also applies if there is no zero of . There are also rules of sums , products , quotients and chains .
The following is a list of fundamental properties of holomorphic functions, none of which have any equivalent in real theory. As a result, let it be an area and holomorphic.
Cauchy's integral theorem
If simply connected and a cycle is in , then Cauchy's integral theorem applies
So the theorem is especially valid if there is a star region and a closed path .
Cauchy's integral formula
Let be the open circular disk with radius around the point . If the conclusion of is still entirely in , then Cauchy's integral formula applies to all and
The value of the function (and each of its derivatives) of a point in an area depends only on the function values at the edge of this area.
Holomorphism and analyticity
A consequence of Cauchy's integral formula is that on the complex level the concept of analyticity is equivalent to holomorphism: every in holomorphic function is in analytical . Conversely, every in analytic function can be continued to an in holomorphic function.
Since power series can be complexly differentiable as often as desired (namely by term-wise differentiation), one obtains in particular that holomorphic functions are differentiable as often as desired and all their derivatives are in turn holomorphic functions. Here one can see clear differences to real differential calculus.
Identity set
It turns out that a holomorphic function is uniquely determined by very little information. The identity theorem says that two holomorphic functions in one domain are completely identical if they agree on a suitable real subset . The correspondence set does not even have to be a continuous path : it is sufficient for it to have an accumulation point in . Discrete subsets , however, are not sufficient for this.
additional
- The Liouville's theorem states that every bounded entire function is constant. A simple consequence of this is the fundamental theorem of algebra .
- Theorem of area loyalty : If an area is and not constant, then there is another area.
- One consequence of area loyalty is the maximum principle .
- Weierstrass theorem : If a sequence of holomorphic functions converges compactly to the limit function , then it is holomorphic again and one can swap the formation of the limit and differentiation, that is, the sequence converges compactly to .
- Montel's theorem : If the sequence of holomorphic functions is restricted to local, then there is a compactly convergent subsequence.
- Every harmonic function that is twice continuously differentiable in a simply connected domain is a real part of a complex differentiable function . The real function also fulfills . It is called the conjugate harmonic and a complex potential.
Biholomorphic functions
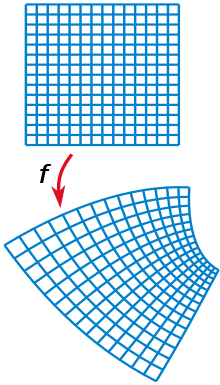
A function that is holomorphic, bijective and whose inverse function is holomorphic is called biholomorphic. In the case of a complex variable, this is equivalent to the mapping being bijective and conformal . From the theorem about implicit functions it already follows for holomorphic functions of a variable that a bijective, holomorphic function always has a holomorphic inverse mapping. In the next section, holomorphic functions of several variables are introduced. In this case, Osgood's theorem guarantees this property. Thus one can say that bijective, holomorphic maps are biholomorphic.
From the point of view of category theory , a biholomorphic map is an isomorphism .
Holomorphism of several variables
In n-dimensional complex space
Be an open subset. A mapping is called holomorphic if it can be expanded into a power series around every point of the domain, that is, there is a polycircle for each , so that
holds for all with coefficients independent of . A function is called holomorphic in the -th variable if it is holomorphic as a function of the remaining variables that are fixed. Holomorphic functions are of course holomorphic in every variable. For the converse, see the equivalent characterizations below.
With the Wirtinger calculus and a calculus is available with which the partial derivatives of a complex function can be treated as with the functions of a variable.
For a function , open, the following statements are equivalent:
- is holomorphic.
- is continuous and holomorphic in every variable ( Osgood's Lemma )
- is holomorphic in every variable ( Hartogs Theorem )
- is continuously differentiable and satisfies the Cauchy-Riemann differential equations for .
For several dimensions in the image area, holomorphism is defined as follows: A mapping , open, is called holomorphic if each of the sub-functions is holomorphic.
Many properties of holomorphic functions of a variable can be transferred, partly in a weakened form, to the case of several variables. Cauchy's integral theorem does not apply to functions and the identity theorem is only valid in a weakened version. For these functions, however, Cauchy's integral formula can be generalized to dimensions by induction . In 1944 Salomon Bochner was even able to prove a generalization of the -dimensional Cauchy's integral formula. This is called the Bochner-Martinelli formula .
In complex geometry
Holomorphic images are also considered in complex geometry . So one can define holomorphic mappings between Riemann surfaces or between complex manifolds analogous to differentiable functions between smooth manifolds . There is also an important counterpart to the smooth differential forms for integration theory , which is called the holomorphic differential form .
literature
- Klaus Jänich : (The first two editions differ significantly from the following. Among other things, from the third edition the four "star" chapters on Wirtinger calculus, Riemann surfaces, Riemann surfaces of a holomorphic nucleus and algebraic functions are missing .)
- Introduction to function theory . 2nd Edition. Springer-Verlag, Berlin / Heidelberg 1980, ISBN 3-540-10032-6 .
- Function Theory - An Introduction . 6th edition. Springer-Verlag, Berlin / Heidelberg 2004, ISBN 3-540-20392-3 .
- Wolfgang Fischer, Ingo Lieb: Function Theory - Complex Analysis in a Variable . 8th edition. Vieweg, Braunschweig / Wiesbaden 2003, ISBN 3-528-77247-6 .
- Eberhard Freitag , Rolf Busam: Function Theory 1 . 3. Edition. Springer, 2000, ISBN 3-540-67641-4 .
- Eberhard Freitag: Function Theory 2. Riemann Surfaces; Multiple complex variables; Abelian functions; Higher modular forms . Springer, 2009, ISBN 978-3-540-87899-5 .
- Reinhold Remmert , Georg Schumacher: Function theory 1 . 5th edition. Springer, Heidelberg 2002, ISBN 3-540-41855-5 .
- Reinhold Remmert, Georg Schumacher: Function Theory 2 . 3. Edition. Springer, Heidelberg 2007, ISBN 978-3-540-40432-3 .

































![{\ displaystyle \ mathbb {C} \ setminus {] {- \ infty}, 0]}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/66a5daa5e481211838fcd8fd950a39a7e3c79356)





















































