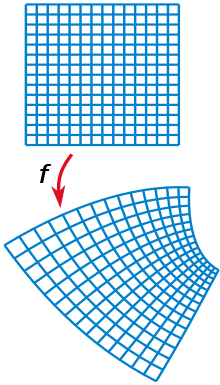
Compliant mapping

Fig. 1: A rectangular mesh (above) and its image (below) after a conformal mapping . Line pairs that intersect at 90 ° are mapped to line pairs that also intersect at 90 °.

{\ displaystyle f}

A conformal mapping is a conformal mapping.

This means, among other things, that from a right-angled coordinate network through a conformal mapping, an i. General The result is a curvilinear coordinate network, but the right-angled network structure is completely retained “on a small scale”, in particular the intermediate angles and the length ratios of any two vectors.

Such images find multiple applications in theoretical physics , u. a. in the theory of complicated electrostatic potentials and the associated electrostatic fields as well as in fluid mechanics .

definition

A linear mapping is called conformal if it applies to all and its determinant is positive ( if it is negative, it is called anti-conformal). Here is the standard scalar product and the Euclidean norm . In other words, (linear) conformal or anti-conformal mappings get the magnitude of the angle between any two vectors; while a conformal maintains the orientation of the angle, it reverses an anti-conformist. ${\ displaystyle L \ colon \ mathbb {R} ^ {m} \ to \ mathbb {R} ^ {n}}$ ${\ displaystyle {\ frac {\ langle Lv, Lw \ rangle} {\ | Lv \ | _ {2} \ | Lw \ | _ {2}}} = {\ frac {\ langle v, w \ rangle} { \ | v \ | _ {2} \ | w \ | _ {2}}}}$ ${\ displaystyle v, w \ in \ mathbb {R} ^ {m}}$ ${\ displaystyle \ langle \ cdot, \ cdot \ rangle}$ ${\ displaystyle \ | \ cdot \ | _ {2}}$

Furthermore is a differentiable map compliant in when their differential in is compliant. ${\ displaystyle f}$ ${\ displaystyle x}$ ${\ displaystyle x}$

properties

If there is an open subset of the complex plane , then the function is conformal if and only if it is holomorphic or anti-holomorphic and its derivative is not equal to zero to whole . The conformal mappings thus form the geometric illustration of the complex differentiable ( analytical or holomorphic ) functions of a complex variable (cf. the illustration of real functions using plane curves). Real or imaginary part of such a function or its locally rectangular coordinate networks can be, for. B. be interpreted as potentials of an electrostatic field or a flow field. Also meromorphic functions are useful because the pole the dipoles , quadrupoles , etc., in general: the multipoles generate these potentials. ${\ displaystyle U}$ ${\ displaystyle \ mathbb {C}}$ ${\ displaystyle f \ colon U \ to \ mathbb {C}}$ ${\ displaystyle U}$

The conformal mappings of the Minkowski space to itself include the Lorentz transformations and translations that leave the metric unchanged, the dilations that scale the metric by a smooth function, and the special conformal transformations that include inversion on a spherical surface ( see ball wave transformation ).

Like the Lorentz transformations and the Poincaré transformations , the conformal transformations also form a Lie group , the conformal group .

Physical applications

Fig. 2: The wing and the circle are connected by a conformal mapping.

The adjacent Figure 2 shows, using an example from “aircraft construction”, that the conformal mapping enables complicated curves to be mapped in a much simpler way. The example shown of a conformal mapping is the Joukowski function (also written as the “Schukowski function”). In this illustration, the Joukowski profile is mapped onto a circle. The speed at which air particles flow around the (two-dimensional) airfoil profile is easier to calculate when it comes to flow around a circular cylinder. This makes it plausible that the conformal mappings have an important meaning in the following areas, as long as one investigates phenomena in the two-dimensional plane:

Fluid dynamics ( aerodynamics , hydrodynamics )
Electrostatics (compare the electrostatic field in analogy to flow fields)
Conduction

Invariance among conformal mappings

In the case of the -dimensional Minkowski space, the following applies: The connected component of the 1 of the group of orientational conformal transformations is isomorphic to the group if the following applies. For this group is infinitely dimensional. It is isomorphic to , where the infinitely dimensional group of orientational diffeomorphisms denotes to itself. ${\ displaystyle d}$ ${\ displaystyle SO (d, 2)}$ ${\ displaystyle d> 2}$ ${\ displaystyle d = 2}$ ${\ displaystyle \ mathrm {Diff} _ {+} (\ mathbb {R}) \ times \ mathrm {Diff} _ {+} (\ mathbb {R})}$ ${\ displaystyle \ mathrm {Diff} _ {+} (\ mathbb {R})}$ ${\ displaystyle \ mathbb {R}}$

In the case of n-dimensional Euclidean space, the corresponding group is isomorphic to , . In the case it is therefore isomorphic to the group of Möbius transformations. ${\ displaystyle d}$ ${\ displaystyle SO (d + 1,1)}$ ${\ displaystyle d \ geq 2}$ ${\ displaystyle d = 2}$

Physical systems that are invariable under conformal mappings have great importance in solid state physics , string theory, and conformal field theory .

Conformal mappings on (semi-) Riemannian manifolds

Let and be two Riemannian manifolds or semi-Riemannian manifolds . and denote the metric tensors . Two metrics and on a manifold are called "conformally equivalent" in Riemannian geometry , if with a positive function defined on which is called the conformal factor . The class of conformal equivalent metrics on is called conformal structure. ${\ displaystyle (M, g)}$ ${\ displaystyle (N, h)}$ ${\ displaystyle g}$ ${\ displaystyle h}$ ${\ displaystyle g}$ ${\ displaystyle h}$ ${\ displaystyle M}$ ${\ displaystyle g = uh}$ ${\ displaystyle M}$ ${\ displaystyle u}$ ${\ displaystyle M}$

A diffeomorphism is called conformal if it holds for all points and vectors of the tangent space . This is also expressed in such a way that the pullback metric on conformal is equivalent to the metric of . The power is intended to indicate that the factor is always greater than 0, i.e. that it is a conforming factor. An example of a conformal mapping is the stereographic projection of the spherical surface onto the projective plane (plane supplemented by a point at infinity). ${\ displaystyle f \ colon M \ to N}$ ${\ displaystyle h_ {f (x)} (\ mathrm {d} f_ {x} (v), \ mathrm {d} f_ {x} (w)) = e ^ {\ sigma (x)} \ cdot g_ {x} (v, w)}$ ${\ displaystyle x \ in M}$ ${\ displaystyle v, w \ in T_ {x} M}$ ${\ displaystyle M}$ ${\ displaystyle M}$ ${\ displaystyle e ^ {\ sigma (x)}}$

The conformal mappings of a manifold into itself are generated by conformal killing vector fields .

literature

Eberhard Freitag , Rolf Busam: Function Theory 1 . 3rd, revised and expanded edition. Springer-Verlag , Berlin a. a. 2000, ISBN 3-540-67641-4 .

Konrad Königsberger : Analysis 2. Springer-Verlag, Berlin / Heidelberg, 2000, ISBN 3-540-43580-8 .

Web links

Representation of a Joukowski profile with flow lines on an analog computer. ( Memento of January 28, 2008 in the Internet Archive ). At: Vaxman.de.
Program with visualization of conforming images, also own formulas. At: 3D-XplorMath.org.

References and footnotes

^ Friedrich Hund : Theoretical Physics. 3 volumes, Stuttgart Teubner, first 1956–1957, Volume 2: Theory of electricity and light, theory of relativity. 4th edition, 1963.

[1] Friedrich Hund : Theoretical Physics. 3 volumes, Stuttgart Teubner, first 1956–1957, Volume 2: Theory of electricity and light, theory of relativity. 4th edition, 1963.