Potential (physics)
The potential or potential (lat. Potentia , " power , power , power ") is in the physics , the ability of a conservative force field , a job to do. It describes the effect of a conservative field on masses or charges regardless of their size and sign . A reaction of the test specimen is thus initially excluded, but can also be taken into account separately. The large Greek letter Phi is usually used as a symbol for the potential .
In mathematics, the term potential refers exclusively to a ( scalar or vectorial ) field , i.e. a position function as a whole. In physical-technical contexts, however, it is used to denote both the field and its individual functional values, such as the electrical or gravitational potential at the relevant point. The following mainly deals with the physical "potential" as a field.
In many textbooks, the potential energy is also referred to as "potential" and the symbol for the potential energy is chosen. A potential (in the real sense) is potential energy per coupling constant , e.g. B. electrical charge or mass .
Basis: the force field
According to Newton , the law applies to a force
- ,
where is a mass and the acceleration that this mass experiences. So it is a question of a force which is exerted on a single object.
In the case of gravity, however, an acceleration (downwards) acts at every point in space, and a mass that is located somewhere in space always experiences a force in that same direction.
Quantities of this kind that are not only located in a single place but are distributed over a space are called fields , and depending on whether the quantities in question are directional or undirected, the fields are divided into vector fields and scalar fields .
Quantities that have no direction such as mass, charge, density or temperature and can be fully described with the help of a single number are also referred to as scalars , and all fields that assign such directionless quantities to places in space are accordingly called scalar fields . For example, you can assign its height above sea level to each point on the earth's surface and thus obtain a scalar height field , or you can assign z. B. assigns its density to each point in space and thus receives a density field .
Forces, on the other hand, are vectors , i.e. directed quantities, and if you assign such a vector instead of a scalar to each point in space, you get a vector field instead of a scalar field . In the case of gravity, for example, all gravity vectors always point in the direction of the center of the earth.
Vector fields, the elements of which are forces, are called force fields , and so the above equation can also be written vectorially with
- ,
where is the force field and the acceleration field . An acceleration field usually depends on the position in space, which means that both and are functions of , so it should be more precisely:
- .
The potential using the example of the electric and the gravitational field
If it is a conservative force such as the Coulomb force or gravity , a force field can also be expressed using a scalar field , for which the following equations then apply, for example (in the case of the electric field , the charge takes on the role of mass in the gravitational field ) :
- or.
- .
A scalar field that fulfills this relationship is called the potential of the vector field .
It is
-
(mostly just written) the Nabla operator
- the expression of the gradient of the field formed with its help .
The application of the Nabla operator to the scalar field generates a vector field that makes a statement about the rate of change of the scalar field in the direction of its steepest rise for each point in space.
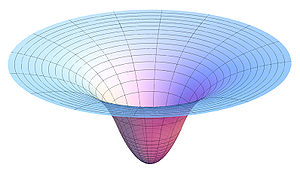
The potential can thus be illustrated well as a hilly landscape, as in the case of the previously mentioned height field: The height of a point is then its potential value, and the force that acts on a body at this point is the vector that is in Shows the direction of the steepest potential gradient, i.e. exactly opposite to the direction of the steepest potential rise .
The force on a charge in the electric field or on a mass in the gravitational field results in
- .
The special importance of the potential lies in the fact that as a scalar field - compared to the three components of a force field - it has only one component, which simplifies many calculations. In addition, its product with the charge or mass directly supplies the potential energy of the specimen in question, and so the equation applies in electrostatics for the potential energy and the electrical potential
- .
In a more general sense, other scalar fields from which vector fields can be derived according to the above equation are also referred to as potentials.
Central potential
A central potential is understood to be a potential that only depends on the distance to the center of force. So it applies with . Movements in a central potential are subject to a conservative central force .
To the omens

The minus signs in the equations
express that the conservative force on a positive charge (positive electrical charge or mass ) - following the principle of the smallest compulsion - always acts in the direction of decreasing potential energy, i.e. opposite to the direction of its gradient or maximum energy increase. In the graphic image of a mountain of potential, acceleration due to gravity and electric field strength (see adjacent figure) therefore always act “downhill”.
For the electric field, however, the situation can become even more complicated because negative central and test charges are also conceivable. When a negative test charge approaches a negative central charge , the potential energy of the test charge increases, although it moves in the direction of the field line, i.e. in the direction of falling electrical potential. The paradox disappears as soon as one takes into account that the product of two negative quantities results in a positive quantity again. The adjacent figure summarizes the relationship between potential energy and electrical potential for the four conceivable sign constellations of the electrical field. As can be seen, the potential energy is always dependent on the sign of both charges, whereas the potential curve depends solely on the sign of the central charge.
A concrete application example of these equations illustrates the content of this relationship a little more clearly: Since the positive direction of coordinate systems on the earth's surface always points vertically upwards and lifting a body higher means that it also gains more potential energy or a higher potential , this potential is approximately at the height above the ground with the amount of acceleration due to gravity .
If you consider the gravitational potential of the earth's gravitational field as an approximate central potential (see above), i.e. depending solely on the distance to the center of the earth or on the height , the gradient from can be reduced to the differential quotient , and the relationship obtained as the equivalent of the above equations
- With
As can be seen from the minus sign, the direction of the acceleration due to gravity is almost opposite to the positive direction of the coordinate system, i.e. pointing towards the center of the earth as expected. In this case, the acceleration calculated from the gravitational potential is exactly equal to the acceleration due to gravity.
Potential energy and potential
Potential energy and potential differ in that,for example, potential energy in the gravitational field relatesto a mass and in the electric field to a charge and depends on the size of this mass or charge, while the potential is a property of the force field independent of a mass or chargesizeof the test specimen.
- The potential is a field representation equivalent to the force field .
The above-mentioned relationship makes it possible to represent a three-dimensional conservative force field with the aid of scalar fields without losing information about the field. This leads to the simplification of many calculations. However, the conclusion about the body causing the field is no longer clear. For example, the external gravitational potential of a homogeneous full sphere is equivalent to the potential of a point mass.
The two variables are linked by the concept of work :
- The power is the ability of a body to perform work from a physical standpoint.
- The potential is used to describe the ability of a field to make a body do work.
The relationship between potential energy and potential is
- .
The first term refers to an electric field (charge ), the second to a gravitational field (mass ).
Potential difference
We always speak of potential difference or potential difference when two or more objects have different potentials from one another. A potential difference is a body-independent measure for the strength of a field and describes the work capacity of an object in this. There is therefore no potential difference along equipotential surfaces (surfaces with the same potential): Objects (bodies, charges) can be moved along them without any effort. In electrostatics, the potential difference is defined as the electrical voltage between two isolated charge carriers (objects of different potential):
- .
Relationship with the charge distribution
| character | description |
|---|---|
| Laplace operator | |
| Permittivity | |
| potential | |
| Gravitational constant | |
| Charge or mass density |
The relationship between the potential and the charge or mass density is established for the Coulomb and gravitational forces using the Poisson equation , a partial differential equation of the second order. In electrostatics it is
- ,
whereas in the classical theory of gravity they take the form
owns.
In order for the above equation to apply in electrostatics, it must be constant. If this requirement is not met, the following expression must be used instead:
Example: gravitational potential of a homogeneous sphere
Since solving the Poisson equation is already relatively complex in simple cases, a detailed example is presented here. To do this, we consider an idealized celestial body as a perfect sphere with homogeneous density and a radius .
Outer solution
Is in the outer space around the ball and so that the Poisson equation in the Laplace equation merges
- .
Since the given problem has spherical symmetry, we can simplify it by considering it in spherical coordinates . All that is required is to insert the corresponding Laplace operator into the equation. This then has the form
- .
Obviously, the field cannot depend on the angles , since the sphere is symmetrical. This means that the derivatives from according to the angular coordinates disappear and only the radial part remains.
- ,
which is further simplified by multiplying with on both sides .
Integration after r delivers
- ,
where is a constant of integration. Further integration after r yields
- ,
where , so that the minus sign disappears and is again a constant of integration.
Because the potential should approach zero at an infinite distance, it must be. The following applies to the external solution
- .
However, in order to compute the constant, we must first find the inner solution.
Inner solution
Inside the sphere is and , so that Poisson's equation holds, with
- .
- .
Integrating twice after r yields in the same way as before
- ,
where here and again are constants of integration. Since the potential in the center of the sphere ( ) should have a finite value , must be. Otherwise the potential would be infinite. So we have
- .
and thus
- .
Determination of the constants
We first differentiate
for the outer solution and
for the inner solution. At the edge of the sphere, the inner potential must transition smoothly into the outer. This means that the first derivatives at must match.
- ,
where we use here that the mass is the product of volume and density, with
- .
From this it follows
- ,
so that is the familiar external solution
results. In order to determine the constant of the inner solution, we use the fact that the potential must be continuous , that is , the two solutions at must be identical, that is, the following applies:
- .
And thus
- .
This finally results for the inner solution
- ,
whereby the first summand was rewritten over the volume.
The inner solution corresponds to a harmonic oscillator potential . This means that if you bored a hole through a homogeneous celestial body (a moon or small planet) and dropped an object into it, it would swing (fall) back and forth through the center. Assuming a frictionless movement, the spatial function of the body results
Gravity in a hollow sphere
What the situation looks like inside a hollow sphere can now also be read directly from our solution for . Generally we had
- ,
since we are now inside the sphere, we cannot go out into infinity, which has previously disappeared. However, the potential in the center must again assume a finite value, so this time will. Then there is the potential
- ,
so constant. The derivative of the potential according to the radius gives the acceleration - the derivative of a constant is zero. So you are weightless inside a hollow sphere. This can be understood from the fact that opposing particles in the walls cancel their gravitation. If it was not a perfect sphere, this would not be the case and one would experience small accelerations.
Web links
- Helmut Föll: Potential - definition math.-vectorial. In: Introduction to Materials Science II. Accessed April 1, 2009 .
Individual evidence
- ^ Bergmann-Schaefer: Textbook of Experimental Physics , Volume 1, limited preview in the Google book search.
- ↑ David Halliday, Robert Resnick: Physics, Part 2 . Walter de Gruyter, 1994, ISBN 3-11-013897-2 , p. 869 ( limited preview in Google Book search).





















































![\ text {div} \ left [\ varepsilon \ cdot \ text {grad} \ \ Phi (\ vec r) \ right] = - \ rho (\ vec r)](https://wikimedia.org/api/rest_v1/media/math/render/svg/90337593a2992721efb665811b2a63841bde8a96)