Mohorovičić discontinuity
The Mohorovičić discontinuity [ ˈmɔhɔrɔʋitʃitɕ ], usually referred to as Moho for short , represents the interface between the earth's crust and mantle within the shell structure of the earth . On the one hand, a discontinuity in the speed of earthquake waves is decisive for this transition , on the other hand it means a change in the mineralogical composition of the rock . The two boundaries do not always coincide, then the transition is not sudden, but continuous over a larger area.
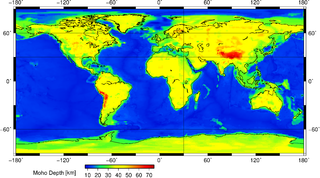
Under the oceans the depth range of the Mohorovičić discontinuity is quite low (≈ 10 km or less), under the continents it is relatively high (≈ 50 km and more), and under tectonically active areas this limit can also be shifted significantly upwards.
discovery
The discontinuity was founded in 1910 by the Croatian geophysicist Andrija Mohorovičić discovered when he seismograms of the earthquake of Pokupsko near the Croatian capital Zagreb from 8. October 1909 analyzed. Mohorovičić noticed that some P and S earthquake waves ( longitudinal and transverse waves ) arrived significantly later than expected, and he suspected that they had broken at a border about 54 km deep . Subsequent investigations confirmed the existence of such a layer boundary at a depth of 30–50 km under continents and in about 5–7 km under oceans.
Earth science background
The difference in speed is caused by the physical differences between crustal and mantle rocks. In many sources (sometimes even textbooks) the higher density of the mantle is wrongly stated as the cause of the higher speed. However, a pure increase in density would reduce the speed. The reason is much more a much higher modulus of elasticity due to different mineralogy. The mantle consists of rocks that are summarized under the name peridotite . The oceanic crust on top, on the other hand, consists mainly of basalt / gabbro and the continental crust of granitic rocks.
Their correspondence as the crust-mantle boundary is only almost correct in some areas, since the relatively sudden change in the p-wave velocity by which it is defined corresponds to a chemical change. However, due to the development, z. As the cumulates , resulting in magma chambers at the mid-ocean ridges absaigern , attributed to the oceanic crust, though they correspond more to chemically the mantle rocks and are thus separated by the Moho from the other crustal rocks.
Since the acoustic impedance changes sharply and abruptly at the Moho discontinuity, seismic waves are not only refracted, but also reflected . The reflections can be observed in a seismogram what a location allows the speed jump. The Moho discontinuity is one of the most prominent discontinuities in the earth's body.
Depth variation of the interface
Under the oceans, the Moho area is on average only 5–7 km deep, under continental areas its depth is 30–50 km. Near the coast , the crust ends at a depth of 20–30 km, in Saxony it is 32 km and under mountain ranges (such as the Andes or Himalayas ) it can reach 70–80 km.
The reason for the changing depth of the Mohorovičić discontinuity is the various forms of formation of the earth's crust: the oceanic crust is formed by the melting of rising mantle material on the mid-ocean ridge , whereas the continental crust is still today due to magmatism at the continental margins ( subduction or collision zones ). The depth of the Moho discontinuity correlates approximately with the height of the topography , because the lighter crust, like a floating iceberg, “dips” deeper into the upper mantle , the higher its mountains rise (see mountain roots and isostasis ).
literature
- László Egyed : Solid Earth Physics . Akadémiai Kiadó, Budapest 1969.
- Walter Kertz : Introduction to Geophysics . Spectrum Academic Publishing House, 1970/1992.
Web links
- Modeling of the Eastern Saxothuringian and Moho . (PDF file; 1.63 MB)
References and footnotes
- ↑ Bruce A. Bolt : Earthquakes - Keys to Geodynamics . Spectrum Akademischer Verlag, Heidelberg 1995, ISBN 3-86025-353-0 , p. 95 f.