Projective straight line

In mathematics , in particular projective geometry , the projective straight line is a one-dimensional projective space .

definition

Let it be a body , for example the body of real or complex numbers or a finite body . It is the (up to isomorphism unique) two-dimensional - vector space . The projective straight line is the set of one-dimensional subspaces of . ${\displaystyle K}$ ${\displaystyle V=K^{2}}$ ${\displaystyle K}$ ${\displaystyle P^{1}K}$ ${\displaystyle V}$

In other words: the projective straight line is the quotient space

${\displaystyle P^{1}K=(K^{2}\setminus \left\{0\right\})/\sim }$

regarding the equivalence relation

${\displaystyle (x_{1},y_{1})\sim (x_{2},y_{2})\Longleftrightarrow \exists \lambda \in K\setminus \{0\}:x_{1}=\lambda x_{2},y_{1}=\lambda y_{2}}$.

This equivalence relation identifies two points precisely when they lie in the same one-dimensional sub-vector space, i.e. on the same straight line through the zero point.

Homogeneous coordinates

Every point of the projective line can be used in homogeneous coordinates as

${\displaystyle \left[x:y\right]}$

with are shown, wherein for all applicable. ${\displaystyle x,y\in K,(x,y)\not =(0,0)}$ ${\displaystyle \left[x:y\right]=\left[\lambda x:\lambda y\right]}$ ${\displaystyle \lambda \in K\setminus \{0\}}$

Number line extended by the point at infinity

On the Riemann number sphere are complex numbers including ∞ represented.

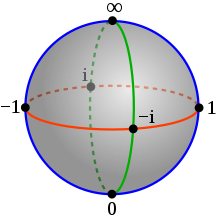
The projective straight line can be identified with the straight line extended by a " point at infinity " . Namely, one can go through the straight line with the in homogeneous coordinates ${\displaystyle P^{1}K}$ ${\displaystyle K\cup \left\{\infty \right\}}$ ${\displaystyle K^{1}}$ ${\displaystyle K=K^{1}}$

${\displaystyle \left\{[x:1]\in \mathbf {P} ^{1}(K)\mid x\in K\right\}}$

given subset of identify. This subset then contains all the points except for one, the so-called "point at infinity": ${\displaystyle P^{1}K}$ ${\displaystyle P^{1}K}$

${\displaystyle \infty =[1:0].}$

Examples

• The real projective straight line is homeomorphic to the circle . ${\displaystyle P^{1}\mathbb {R} }$ ${\displaystyle S^{1}}$
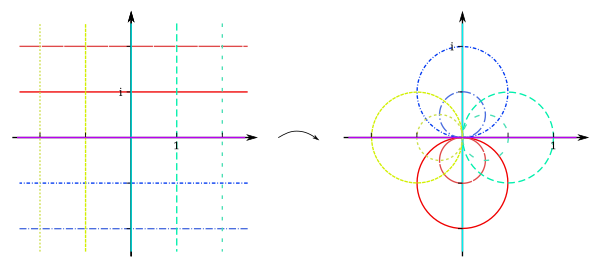
• The complex projective straight line is called the Riemann sphere , it is homeomorphic to the 2-sphere . ${\displaystyle P^{1}\mathbb {C} }$ ${\displaystyle S^{2}}$
• The projective straight line over the finite body has elements. ${\displaystyle P^{1}F_{q}}$ ${\displaystyle F_{q}}$ ${\displaystyle q+1}$

Automorphisms

Möbius transformation on ${\displaystyle P^{1}\mathbb {C} =\mathbb {C} \cup \left\{\infty \right\}}$

The general linear group acts through linear maps . The projective linear group is the factor group , with the normal (even central ) subgroup of the scalar multiples of identity being with off . The effect of on induces a well-defined effect of on . The automorphisms of are by definition the mappings described by elements of . ${\displaystyle GL(2,K)}$ ${\displaystyle K^{2}}$ ${\displaystyle \mathrm {PGL} (2,K)}$ ${\displaystyle \mathrm {GL} (2,K)/K^{\times }}$ ${\displaystyle K^{\times }}$ ${\displaystyle k\cdot \mathrm {id} _{K^{2}}}$ ${\displaystyle \mathrm {id} :K^{2}\rightarrow K^{2}}$ ${\displaystyle k}$ ${\displaystyle K\setminus \{0\}}$ ${\displaystyle GL(2,K)}$ ${\displaystyle K^{2}}$ ${\displaystyle PGL(2,K)}$ ${\displaystyle P^{1}K}$ ${\displaystyle P^{1}K}$ ${\displaystyle PGL(2,K)}$ ${\displaystyle P^{1}K\to P^{1}K}$

In homogeneous coordinates, the matrices act as fractional-linear transformations :

${\displaystyle \left({\begin{matrix}a&b\\c&d\end{matrix}}\right)z={\frac {az+b}{cz+d}}}$

after identification . ${\displaystyle \left[x_{0}:x_{1}\right]\simeq z:={\frac {x_{0}}{x_{1}}}\in K\cup \left\{\infty \right\}}$

The automorphism group acts transitively on triples of different points in pairs.

A fundamental invariant of projective geometry is the double ratio of 4-tuples in pairs of different points. If K is algebraically closed, two such 4-tuples can be converted into one another by an automorphism if and only if their double ratio matches.

In this case , the automorphisms of are called Möbius transformations . ${\displaystyle K=\mathbb {C} }$ ${\displaystyle P^{1}\mathbb {C} }$

Projective straight lines in the projective plane

The projective straight line through two given points and the projective plane is determined by interpreting the two points as straight lines im (and describing them by their straight line equation ), calculating the plane containing them (see plane equation ) and then mapping this plane to a projective straight line in projected. ${\displaystyle \left[x_{1}:y_{1}:z_{1}\right]}$ ${\displaystyle \left[x_{2}:y_{2}:z_{2}\right]}$ ${\displaystyle K^{3}}$ ${\displaystyle K^{3}}$ ${\displaystyle P^{2}K}$

Similarly, one determines projective straight lines through two given points in a higher-dimensional projective space.

literature

• Klein, Felix: Elementary mathematics from a higher point of view. Volume two: Geometry. Third edition. Elaborated by E. Hellinger. Made ready for printing and provided with additions by Ms. Seyfarth. The basic teachings of the mathematical sciences, Volume 15 Springer-Verlag, Berlin 1968
• Aczél, J .; Gołąb, S .; Kuczma, M .; Siwek, E .: The double ratio as a solution to a functional equation. Ann. Polon. Math. 9 1960/1961 183-187.
• Kerby, William: A Note on Groups PGL (2, F). Results Math. 15 (1989) no. 3-4, 291-293.

Web links

• Projective Geometry - 2D