Orbital motion (water waves)
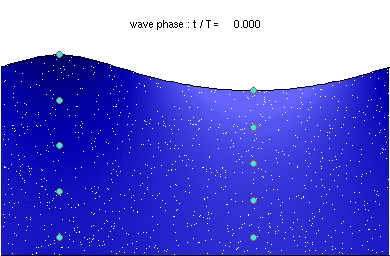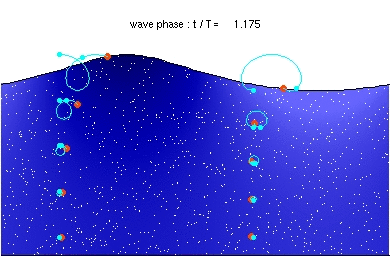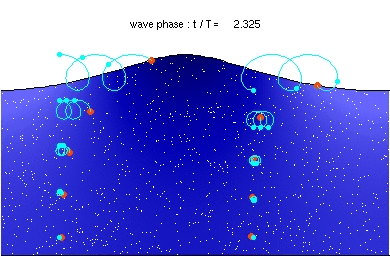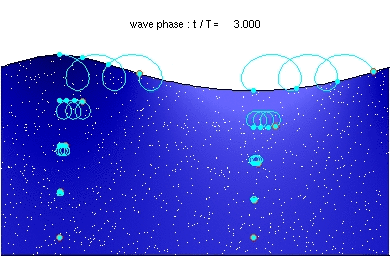
Under orbital motion (from the Latin Orbis , circle) in water waves understood the movement of the water particles. In deep water, the trajectories of the water particles are almost circular when they pass a wave and in shallow water they are almost elliptical . The deviation from the exactly circular or exactly elliptical orbit shape is due to a superimposed drift speed or mass transport speed , which is in the same direction as the wave advancement speed . The drift speed increases with increasing wave steepness ( wave height, wave length ) and causes the formation of spiral orbital orbital paths, see animations opposite.
Particle movement in the wave field
Wave theories used in practice assume that any orbital movement at a depth that corresponds to about half the wavelength is negligibly small and that wave-generated mixing - in contrast to the effects of other currents - no longer takes place below this mark. As an approximation, the decrease in the amplitude of movement with increasing distance from the water surface is assumed according to an exponential law.
It is often sufficient to disregard mass transport; especially for waves over great depths of water ( deep water waves ), for which the trochoidal theory according to Gerstner delivers satisfactory results both for the circular water particle kinematics and for the surface contour, see picture above. The wave period ( ; reciprocal of the frequency ) is the orbital period, which corresponds to the rise of the wave to a full wavelength. Thus the orbital velocity is at the water surface
and the wave advance speed
- .
In the case of periodic waves that run into areas of decreasing water depth, the previously circular orbits change to open ellipses until the orbital movement in the course of the breakwater loses its circular character because its horizontal component increases at the expense of the vertical component.
After the breaking process (not yet shown here) the wave movement is aperiodic ; the movement of the individual water particles extends over the entire water depth approximately equally horizontally in the direction of the coast.
Technical importance
For practical applications, the resulting orbital velocity is broken down into two mutually perpendicular components. Since no velocity components perpendicular to it are possible at a fixed limit, the tangential component is roughly doubled here . Accordingly, the amplitude of the horizontal oscillation of the water particles at the bottom is about twice as large as the amplitude that would exist at the same depth if the bottom were not there. This situation is of particular importance because of wave generated Sohl currents of sediment will transport causes.
The same applies to the doubling of the vertical oscillation amplitude of the water particles on a vertical wall, where a standing wave ( clapotis ) is formed.
To estimate the wave forces that are exerted on submerged building structures (e.g. pile structures , underwater pipelines ), the orbital acceleration is used in the relevant force approaches in addition to the local orbital velocity .
history
The trochoidal wave theory was developed by Franz Josef von Gerstner in 1804 and is to be regarded as the basis for all subsequent wave theories , as it already contains the decrease in orbital orbital orbital radii towards the sea floor. Gerstner assumed the rotation of the water particles around orbital centers and obtained the form of a cyclic curve for the water surface .
All other approximate solutions known today are also based on the trajectory lines of the water particles or using the speed potential . The linear wave theory according to Airy / Laplace (1845), which is based on a cosine function as a waveform and does not contain any mass transport, is preferred . In contrast to this are the higher order wave theories according to Stokes (1880). For shallow water waves was of Korteweg and De Vries (1895), the Cnoidaltheorie developed for very shallow water near the breaker zone of Munk (1949), the single wave theory .
literature
- Fritz Büsching : About orbital velocities of irregular surf waves. Leichtweiß-Institut für Wasserbau, Braunschweig 1974 ( Leichtweiß-Institut für Wasserbau der Technische Universität Braunschweig Mitteilungen 42, ISSN 0343-1223 ).
- Andreas Malcherek: Tides and waves - The hydromechanics of the coastal waters . 1st edition. Wiesbaden: Vieweg + Teubner, 2010. ISBN 978-3-8348-0787-8
Web links
- Trochoidal wave theory in "Energy of regular waves" ( Memento from November 5, 2010 in the Internet Archive ), University of Leipzig , Section: 4.2.3.4 (PDF, 1.00 MB)
- Orbital paths and orbital velocities (PDF, 709 kB)