Harmonics
A harmonic is in the classic physics and technology a harmonic oscillation , whose frequency is an integer multiple of a fundamental frequency is. A harmonic above the fundamental frequency is also called harmonic , harmonic and overtone in music .
As a function of time, the harmonic describes a purely sinusoidal oscillation. Harmonics play a role in music as well as in mechanics , electrical engineering and optics .
Designations
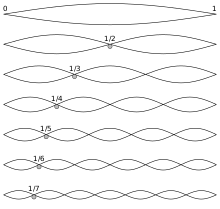
The following designations apply: The fundamental oscillation of frequency f is called the 1st harmonic, an oscillation of twice the frequency ( 2f ) is called the 2nd harmonic or 1st harmonic. In general, the oscillation with n times the frequency of the fundamental frequency ( nf ) is the nth harmonic, i.e. the (n − 1). Harmonic. All harmonics except the 1st harmonic are called higher harmonics.
The fundamental is the 1st harmonic, an octave above is the 2nd harmonic, which is the 1st overtone. The overtone is numerically always one number lower than the harmonic. Even harmonics are odd overtones and vice versa.
Basics
With the DFT you can set any signal curves that e.g. B. be generated with a musical instrument as a sound or an oscillator as an electrical audio signal or other signal, decompose into their frequency spectrum . Technically, this analysis can be carried out with a spectrum analyzer .
For every periodic signal it can be seen that it can be broken down into a sinusoidal fundamental frequency f and many other sinusoidal harmonic frequencies with integral multiples of the fundamental frequency 2 f , 3 f , 4 f , etc. In the analysis, any periodic signal curves turn out to be the sum of u. U. infinitely many sinusoidal signals. It is also possible to reverse this fact for the synthesis of periodic signals, but the original cannot be reproduced with absolute precision by analysis and subsequent synthesis. In contrast to the analysis of periodic signal curves, the decomposition of a non-periodic signal results in a continuous frequency spectrum that can contain all frequencies.
In the case of harmonically complex tones , the frequencies are in an integer ratio to each other and to the basic frequency. In music, tones sounding at the same time with such frequency relationships are perceived as harmonic sound and the harmonics are referred to as overtones. Hence the term in the more general context described here. In the case of tones that are approximately harmonically complex , higher frequency components do not have an exact integer relation to the basic frequency and already have a non-negligible proportion of inharmonicity . In the case of low harmonic complex tones , tone signals have partial tone frequencies that already deviate considerably from the harmonic pattern. This includes all sounds that are created by striking bells, rods or tubes or membrane-like bodies.
In music, the signal is a sound. Every sound is made up of the fundamental and the overtones. Here the relative strengths, physically the amplitude ratios of the overtones, determine the timbre of the tone. With terms such as partials , partial tones or harmonic frequencies, the basic frequency is counted in audio technology. If one speaks of overtones, the basic frequency is not counted and only the multiples of the basic frequency are considered. In the literature, there are also terms such as subharmonic tone series , which is based on the mathematical definitions for subharmonic function .
In electrical engineering and communications engineering , the proportion of signals with harmonic frequencies that are added to the original signal when passing through a system (e.g. amplifier or transmission link) determines how much this sinusoidal input signal (with the fundamental frequency) is distorted when passing through . These distortions are rated as distortion factor . The resulting integer multiples of the basic frequency are superimposed at the output of the system of the basic frequency. In power electronics , the harmonic frequencies generated by rectifiers , for example , have disruptive repercussions on the public supply network operated with AC voltage. The harmonic frequencies that occur above the mains frequency are reduced by means of the power factor correction.
Terminology
Often the terms harmonics , harmonics and harmonics are used synonymously for vibrations with an integral multiple of a fundamental frequency. In general, the terms are further differentiated, so that the 1st harmonic of the vibration at the fundamental frequency ( fundamental wave ) and the first harmonic represents the vibration at twice the fundamental frequency. In general, the nth harmonic corresponds to (n − 1). Harmonic.
A distinction must be made between harmonics and harmonics in two ways:
- When talking about the processes involved in sending the signal (i.e. vibrations ), the corresponding concept is called harmonics : "Vibration of the transmitter with a harmonic (oscillation) frequency" - not to be confused with harmonic oscillation . If one looks at the carrier of the signal, such as air for tones, the electromagnetic field for radio signals, etc., then one speaks of harmonics .
- A harmonic is a higher harmonic of the periodic dependence of a quantity over time.
- A harmonic is the higher harmonic of the periodic dependence of a variable in the location.
Example: Concert pitch a 'and the first four harmonics
This table shows the fundamental a ' (that is the concert pitch with the fundamental frequency f = 440 Hz) and its first three overtones with their respective order n and their frequencies. The n . Harmonic generally has the frequency n · f .
| frequency | 1 x f = 440 Hz | 2 · f = 880 Hz | 3 * f = 1320 Hz | 4 x f = 1760 Hz |
|---|---|---|---|---|
| order | n = 1 | n = 2 | n = 3 | n = 4 |
| Keynote | 1st overtone | 2nd overtone | 3rd overtone | |
| 1st partial | 2nd partial | 3rd partial | 4th partial | |
| 1st harmonic | 2nd harmonic | 3rd harmonic | 4th harmonic |
See also
literature
- Michael Dickreiter, Volker Dittel, Wolfgang Hoeg, Martin Wöhr (eds.): Handbuch der Tonstudiotechnik , 8th, revised and expanded edition, 2 volumes, publisher: Walter de Gruyter, Berlin / Boston, 2014, ISBN 978-3-11- 028978-7 or e- ISBN 978-3-11-031650-6
- Dieter Meschede (Ed.): Gerthsen . Physics. 22nd, completely revised edition. Springer, Berlin et al. 2004, ISBN 3-540-02622-3 .
Web links
- Harmonics, partials, partials and overtones (PDF; 255 kB)
- The partial tone density / The partial tone series (PDF; 47 kB)
- Calculate the harmonics from the fundamental frequency in Hz - fundamental frequencies, harmonics and overtones
- Differentiate harmonics, partials, partials and overtones (PDF; 42 kB)
Individual evidence
- ↑ Jörg Jahn: Energy conditioning in low-voltage networks with special consideration of the integration of distributed energy producers in weak network extensions . kassel university press GmbH, 2007, ISBN 978-3-89958-377-9 . , P. 14.
- ↑ Ludwig Bergmann, Clemens Schaefer: Mechanics - Acoustics - Heat theory . Walter de Gruyter, January 1, 1945, ISBN 978-3-11-151095-8 . , P. 390.
- ↑ Manfred Albach: Fundamentals of electrical engineering 2: Periodic and non-periodic signal forms . Pearson Deutschland GmbH, June 2011, ISBN 978-3-86894-080-0 . , P. 130.
- ↑ Germar Müller, Bernd Ponick: Theory of electrical machines . Wiley-VCH, January 14, 2009, ISBN 978-3-527-40526-8 , pp. 56-. , P.56.
