Spherical surface functions

Representation of the first spherical surface functions. The colors indicate the sign of the spherical surface function (red corresponds to positive, green corresponds to negative)

Illustration of some spherical surface functions (rotating around the z-axis). Is shown , where corresponds to the row and the column. Rows and columns are numbered starting with zero. ${\displaystyle Y_{l,m}}$ ${\displaystyle l}$ ${\displaystyle m}$

The spherical surface functions are a complete and orthonormal set of eigenfunctions of the angular component of the Laplace operator . This angular component is shown when the Laplace operator is written in spherical coordinates . The eigenvalue equation is:

${\displaystyle \left({\frac {\partial ^{2}}{\partial \vartheta ^{2}}}+{\frac {\cos \vartheta }{\sin \vartheta }}{\frac {\partial }{\partial \vartheta }}+{\frac {1}{\sin ^{2}\vartheta }}{\frac {\partial ^{2}}{\partial \varphi ^{2}}}\right)Y_{lm}(\vartheta ,\varphi )=-l(l+1)Y_{lm}(\vartheta ,\varphi )}$

The eigenfunctions are the spherical surface functions , with normalization factors and the assigned Legendre polynomials (details see below): ${\displaystyle Y_{lm}(\vartheta ,\varphi )}$ ${\displaystyle N_{lm}}$ ${\displaystyle P_{lm}(z)}$

${\displaystyle Y_{lm}:\;\left[0,\pi \right]\times \left[0,2\pi \right]\rightarrow \mathbb {C} ,\quad (\vartheta ,\varphi )\mapsto {\frac {1}{\sqrt {2\pi }}}\,N_{lm}\,P_{lm}(\cos \vartheta )\,e^{\mathrm {i} m\varphi }}$ ${\displaystyle \quad {\text{mit}}\quad N_{lm}:={\sqrt {{\tfrac {2l+1}{2}}\,{\tfrac {(l-m)!}{(l+m)!}}}}}$

In theoretical physics in particular, the spherical surface functions are of great importance for the solution of partial differential equations . They occur, for example, in the calculation of atomic orbitals , since the descriptive time-independent Schrödinger equation contains the Laplace operator and the problem can best be solved in spherical coordinates. The boundary value problems that occur in electrostatics can also be elegantly solved by developing according to spherical surface functions. In geophysics and geodesy , the spherical surface functions are used to approximate the geoid and the magnetic field.

Relation to the Laplace operator

The angular component of the Laplace operator is shown when it is written in spherical coordinates :

${\displaystyle \Delta ={\frac {\partial ^{2}}{\partial r^{2}}}+{\frac {2}{r}}{\frac {\partial }{\partial r}}+{\frac {1}{r^{2}}}\left({\frac {\partial ^{2}}{\partial \vartheta ^{2}}}+{\frac {\cos \vartheta }{\sin \vartheta }}{\frac {\partial }{\partial \vartheta }}+{\frac {1}{\sin ^{2}\vartheta }}{\frac {\partial ^{2}}{\partial \varphi ^{2}}}\right)=\Delta _{r}+{\frac {1}{r^{2}}}\Delta _{\vartheta ,\varphi }}$

The right, bracketed part is referred to here as the angle part . It is directly proportional to the square of the angular momentum operator . ${\displaystyle \Delta _{\vartheta ,\varphi }}$ ${\displaystyle {\hat {\mathbf {L} }}^{2}=-\hbar ^{2}\Delta _{\vartheta ,\varphi }}$

The Laplace equation in spherical coordinates

${\displaystyle \Delta f(r,\vartheta ,\varphi )\ =0}$

In addition to the trivial solution,, has a wide variety of solutions with many technical applications. ${\displaystyle f=0}$

The following product approach is used to solve this, whereby it only depends on the radius and only on the polar and azimuth angles: ${\displaystyle R_{l}(r)}$ ${\displaystyle Y_{lm}(\vartheta ,\varphi )}$

${\displaystyle f(r,\vartheta ,\varphi )=R_{l}(r)Y_{lm}(\vartheta ,\varphi )}$

This results in inserted:

${\displaystyle \Delta R_{l}(r)Y_{lm}(\vartheta ,\varphi )=Y_{lm}(\vartheta ,\varphi )\Delta _{r}R_{l}(r)+{\frac {R_{l}(r)}{r^{2}}}\Delta _{\vartheta ,\varphi }Y_{lm}(\vartheta ,\varphi )=0}$

Multiplication of and division by gives: ${\displaystyle r^{2}}$ ${\displaystyle R_{l}(r)Y_{lm}(\vartheta ,\varphi )}$

${\displaystyle {\frac {r^{2}\Delta _{r}R_{l}(r)}{R_{l}(r)}}+{\frac {\Delta _{\vartheta ,\varphi }Y_{lm}(\vartheta ,\varphi )}{Y_{lm}(\vartheta ,\varphi )}}=0}$

This equation can only be fulfilled if the radius and angle can be varied independently of one another in both summands. Both summands must therefore assume the same constant value that is chosen (this determination will prove useful later): ${\displaystyle l(l+1)}$

${\displaystyle {\frac {r^{2}\Delta _{r}R_{l}(r)}{R_{l}(r)}}=l(l+1)=-{\frac {\Delta _{\vartheta ,\varphi }Y_{lm}(\vartheta ,\varphi )}{Y_{lm}(\vartheta ,\varphi )}}}$

With this procedure, which is called the separation approach , the original problem, namely the solution of the Laplace equation ( partial differential equation with three independent variables), was reduced to the simpler problem of solving an ordinary differential equation (radial equation)

${\displaystyle \Delta _{r}R_{l}(r)={\frac {l(l+1)}{r^{2}}}R_{l}(r)}$

and a partial differential equation with two independent variables (angle-dependent equation), which is just satisfied by the spherical surface functions.

${\displaystyle \Delta _{\vartheta ,\varphi }Y_{lm}(\vartheta ,\varphi )=-l(l+1)Y_{lm}(\vartheta ,\varphi )}$

Now, due to the orthogonality and completeness of the spherical surface functions, it can be shown that every square-integrable function can be composed of these special functions as a sum:

${\displaystyle f(r,\vartheta ,\varphi )\ =\sum _{l,m}R_{l}(r)Y_{lm}(\vartheta ,\varphi )}$

Due to the linearity of the Laplace operator, any number of solutions to the Laplace equation can be constructed by adding the solutions to the radial equation multiplied by the spherical surface functions. This automatically results in a representation of the solution space of the Laplace equation.

The spherical functions have been dealt with in particular by Legendre (spherical functions of the first kind), Laplace (spherical functions of the second kind) and Carl Gottfried Neumann (spherical functions with several variables).

Solution of the eigenvalue equation

The eigenvalue equation

${\displaystyle \left({\frac {\partial ^{2}}{\partial \vartheta ^{2}}}+{\frac {\cos \vartheta }{\sin \vartheta }}{\frac {\partial }{\partial \vartheta }}+{\frac {1}{\sin ^{2}\vartheta }}{\frac {\partial ^{2}}{\partial \varphi ^{2}}}\right)Y_{lm}(\vartheta ,\varphi )=-l(l+1)Y_{lm}(\vartheta ,\varphi )}$

is separated with the following product approach:

${\displaystyle Y_{lm}(\vartheta ,\varphi )=\Theta _{lm}(\vartheta )\Phi _{m}(\varphi )}$

Re-sorting delivers:

${\displaystyle \underbrace {{\frac {\sin ^{2}\vartheta }{\Theta _{lm}(\vartheta )}}\left({\frac {\partial ^{2}}{\partial \vartheta ^{2}}}+{\frac {\cos \vartheta }{\sin \vartheta }}{\frac {\partial }{\partial \vartheta }}\right)\Theta _{lm}(\vartheta )+\sin ^{2}(\vartheta )(l(l+1))} _{m^{2}}=\underbrace {-{\frac {1}{\Phi _{m}(\varphi )}}{\frac {\partial ^{2}}{\partial \varphi ^{2}}}\Phi _{m}(\varphi )} _{m^{2}}}$

In order to be able to vary both sides separately, both sides must assume the same constant value. This separation constant is chosen as. There are two ordinary differential equations, the polar equation ${\displaystyle m^{2}}$

${\displaystyle {\frac {1}{\Theta _{lm}(\vartheta )}}\left({\frac {\partial ^{2}}{\partial \vartheta ^{2}}}+{\frac {\cos \vartheta }{\sin \vartheta }}{\frac {\partial }{\partial \vartheta }}\right)\Theta _{lm}(\vartheta )={\frac {m^{2}}{\sin ^{2}\vartheta }}-l(l+1)}$

and the azimuthal equation .

${\displaystyle {\frac {\partial ^{2}}{\partial \varphi ^{2}}}\Phi _{m}(\varphi )=-m^{2}\Phi _{m}(\varphi )}$

The azimuthal equation is solved by, whereby, because of the additional condition of uniqueness on the surface of the sphere , they are restricted to whole numbers . With you get the normalized solution of the azimuthal equation: ${\displaystyle \Phi _{m}(\varphi )=A\exp(\mathrm {i} m\varphi )}$ ${\displaystyle m}$ ${\displaystyle \Phi _{m}(\varphi +2\pi )=\Phi _{m}(\varphi )}$ ${\displaystyle \exp(\mathrm {i} m2\pi )=1}$ ${\displaystyle \int _{0}^{2\pi }|\Phi _{m}(\varphi )|^{2}\mathrm {d} \varphi {\overset {!}{=}}1}$

${\displaystyle \Phi _{m}(\varphi )={\frac {1}{\sqrt {2\pi }}}\exp(\mathrm {i} m\varphi ),\quad m\in \mathbb {Z} }$

The polar equation can be solved with a power series approach. The solutions are only finite, unambiguous and continuous if

${\displaystyle l\in \mathbb {N} _{0},\quad |m|\leq l}$.

Then the solutions are the assigned Legendre polynomials and with one gets the normalized solution of the polar equation: ${\displaystyle P_{lm}(\cos \vartheta )}$ ${\displaystyle \int _{0}^{\pi }|\Theta _{lm}(\vartheta )|^{2}\sin(\vartheta )\mathrm {d} \vartheta {\overset {!}{=}}1}$

${\displaystyle \Theta _{lm}(\vartheta )={\sqrt {{\frac {2l+1}{2}}\cdot {\frac {(l-m)!}{(l+m)!}}}}\,\,P_{lm}(\cos \vartheta )}$

The total solution of the angle component is the product of the two solutions obtained, namely the spherical surface functions.

${\displaystyle Y_{lm}(\vartheta ,\varphi )=\Theta _{lm}(\vartheta )\Phi _{m}(\varphi )={\frac {1}{\sqrt {2\pi }}}{\sqrt {{\frac {2l+1}{2}}\cdot {\frac {(l-m)!}{(l+m)!}}}}\,\,P_{lm}(\cos \vartheta )\exp(\mathrm {i} m\varphi )}$

presentation

3D plot of the spherical surface functions (here instead of and instead of ) for degrees ${\displaystyle n}$ ${\displaystyle l}$ ${\displaystyle \theta }$ ${\displaystyle \vartheta }$ ${\displaystyle Y_{nm}(\theta ,\varphi )\propto P_{nm}(\cos \theta )\exp(im\varphi )}$ ${\displaystyle n=5}$

The representation of the spherical surface functions results from the solution of the eigenvalue equation mentioned above. The concrete invoice delivers: ${\displaystyle Y_{lm}:S^{2}\rightarrow \mathbb {C} }$

${\displaystyle Y_{lm}(\vartheta ,\varphi ):={\frac {1}{\sqrt {2\pi }}}N_{lm}P_{lm}(\cos \vartheta )e^{\mathrm {i} m\varphi }}$

Are there

${\displaystyle P_{lm}(x):={\frac {(-1)^{m}}{2^{l}l!}}(1-x^{2})^{\frac {m}{2}}{\frac {\mathrm {d} ^{l+m}}{\mathrm {d} x^{l+m}}}(x^{2}-1)^{l}}$

the assigned Legendre polynomials and

${\displaystyle N_{lm}:={\sqrt {{\frac {2l+1}{2}}\cdot {\frac {(l-m)!}{(l+m)!}}}}}$

are normalization factors. Sometimes the calculation is via:

${\displaystyle P_{lm}(x)=(1-x^{2})^{\frac {\left|m\right|}{2}}\left({\frac {\partial }{\partial x}}\right)^{\left|m\right|}P_{l}(x)}$

With

${\displaystyle P_{l}(x)={\frac {1}{2^{l}}}\sum _{k=0}^{\lfloor l/2\rfloor }(-1)^{k}{\frac {(2l-2k)!}{k!(l-k)!(l-2k)!}}x^{l-2k}}$

more advantageous ( ), since multiple derivation is not required. ${\displaystyle \lfloor l/2\rfloor :={\mathrm {abrunden} }(l/2)}$ ${\displaystyle l}$

Another definition is about homogeneous, harmonic polynomials . These are clearly determined by their value on the sphere. Every homogeneous harmonic polynomial of degree n can be written as a linear combination of spherical surface functions multiplied by and vice versa. For example, if you choose the function, which is constant 1, as the basis of the one-dimensional vector space of the 0-homogeneous harmonic polynomials and x, y and z as the basis of the three-dimensional vector space of the 1-homogeneous, you get the functions in spherical coordinates after dividing ${\displaystyle r^{n}}$ ${\displaystyle r^{n}}$

${\displaystyle 1{\frac {}{}}}$

${\displaystyle \cos {\varphi }\sin \vartheta =\Re {(e^{\mathrm {i} \varphi })}\sin \vartheta }$,

${\displaystyle \sin \varphi \sin \vartheta =\Im {(e^{\mathrm {i} \varphi })}\sin \vartheta }$,

${\displaystyle \cos \vartheta {\frac {}{}}}$.

For the homogeneous polynomials of degree 2 you can quickly recognize the terms in the list below , only with a wrong prefactor. ${\displaystyle x^{2}-y^{2},xy,x^{2}+y^{2}-2z^{2}{\frac {}{}}}$

properties

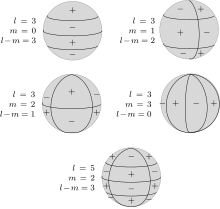
Representation of the spherical surface functions

The spherical surface functions have the following properties:

• Orthogonality relation : ( is the Kronecker delta ) ${\displaystyle \delta _{ij}}$

${\displaystyle {\begin{aligned}&\int Y_{lm}^{*}(\vartheta ,\varphi )\,Y_{l'm'}(\vartheta ,\varphi )\mathrm {d} \Omega \\&\quad =\int _{0}^{2\pi }\int _{0}^{\pi }Y_{lm}^{*}(\vartheta ,\varphi )\,Y_{l'm'}(\vartheta ,\varphi )\,\sin {\vartheta }\,\mathrm {d} \vartheta \,\mathrm {d} \varphi \\&\quad =\delta _{l\,l'}\,\delta _{mm'}\\\end{aligned}}}$

• Completeness : ( is the delta distribution ) ${\displaystyle \delta (x)}$

${\displaystyle \sum _{l=0}^{\infty }\sum _{m=-l}^{l}Y_{lm}^{*}(\vartheta ',\varphi ')\,Y_{lm}(\vartheta ,\varphi )=\delta (\varphi -\varphi ')\delta (\cos {\vartheta }-\cos {\vartheta '})}$

• Parity : The transition looks in spherical coordinates as follows: . Under this transformation, the spherical surface functions behave as follows: ${\displaystyle {\vec {r}}\rightarrow -{\vec {r}}}$ ${\displaystyle (r,\vartheta ,\varphi )\rightarrow (r,\pi -\vartheta ,\pi +\varphi )}$

${\displaystyle Y_{lm}(\pi -\vartheta ,\pi +\varphi )=(-1)^{l}\cdot Y_{lm}(\vartheta ,\varphi )}$

• Complex conjugation : The respective can be obtained from : ${\displaystyle Y_{l,-m}}$ ${\displaystyle Y_{lm}}$

${\displaystyle Y_{l,-m}(\vartheta ,\varphi )=(-1)^{m}\cdot Y_{lm}^{*}(\vartheta ,\varphi )}$

Development according to spherical surface functions

The spherical surface functions form a complete system of functions. Therefore, all square-integrable functions (with and in the sense of spherical coordinates) can be expanded according to the spherical surface functions: ${\displaystyle f(\vartheta ,\varphi )}$ ${\displaystyle \vartheta }$ ${\displaystyle \varphi }$

${\displaystyle f(\vartheta ,\varphi )=\sum _{l=0}^{\infty }\sum _{m=-l}^{+l}c_{lm}Y_{lm}(\vartheta ,\varphi )}$

The expansion coefficients are calculated as follows: ${\displaystyle c_{lm}}$

${\displaystyle c_{lm}=\int _{\varphi =0}^{2\pi }\int _{\vartheta =0}^{\pi }Y_{lm}^{*}(\vartheta ,\varphi )\cdot f(\vartheta ,\varphi )\cdot \sin \vartheta \,\mathrm {d} \vartheta \,\mathrm {d} \varphi }$

It is the complex conjugate to . The representation of a function with - and - function as a Fourier series is an analogue to the development of a two-dimensional function with on a spherical surface. ${\displaystyle Y_{lm}^{*}(\vartheta ,\varphi )}$ ${\displaystyle Y_{lm}(\vartheta ,\varphi )}$ ${\displaystyle f(x)}$ ${\displaystyle \sin }$ ${\displaystyle \cos }$ ${\displaystyle f(\vartheta ,\varphi )}$ ${\displaystyle Y_{lm}(\vartheta ,\varphi )}$

Addition theorem

One result for the spherical surface functions is the addition theorem. For this purpose, two unit vectors and are represented by spherical coordinates and . The following then applies to the angle between these two vectors ${\displaystyle {\vec {x}}}$ ${\displaystyle {\vec {x}}'}$ ${\displaystyle (\vartheta ,\,\varphi )}$ ${\displaystyle (\vartheta ',\,\varphi ')}$ ${\displaystyle \gamma }$

${\displaystyle \cos \gamma =\cos \vartheta \cos \vartheta '+\sin \vartheta \sin \vartheta '\cos(\varphi -\varphi ')\,.}$

The addition theorem for spherical surface functions now says

${\displaystyle P_{l}(\cos \gamma )={\frac {4\pi }{2l+1}}\sum _{m=-l}^{l}Y_{lm}(\vartheta ,\varphi )Y_{lm}^{*}(\vartheta ',\varphi ').}$

The theorem can also be written with the assigned Legendre functions instead of the spherical surface functions ${\displaystyle Y_{lm}}$ ${\displaystyle P_{lm}}$

${\displaystyle P_{l}(\cos \gamma )=P_{l}(\cos \vartheta )P_{l}(\cos \vartheta ')+2\sum _{m=1}^{l}{\frac {(l-m)!}{(l+m)!}}P_{lm}(\cos \vartheta )P_{lm}(\cos \vartheta ')\cos(m(\varphi -\varphi ')).}$

For is obtained from the addition theorem ${\displaystyle \gamma =0}$

${\displaystyle \sum _{m=-l}^{l}|Y_{lm}(\vartheta ,\varphi )|^{2}={\frac {2l+1}{4\pi }}.}$

This can be seen as a generalization of identity on three dimensions and is known as the Unsöld theorem (after Albrecht Unsöld ). ${\displaystyle \cos ^{2}\vartheta +\sin ^{2}\vartheta =1}$

The first spherical surface functions

The first spherical surface functions

Y lm	l = 0	l = 1	l = 2	l = 3
m = −3				${\displaystyle {\sqrt {\tfrac {35}{64\pi }}}\sin ^{3}{\vartheta }\,e^{-3\mathrm {i} \varphi }}$
m = −2			${\displaystyle {\sqrt {\tfrac {15}{32\pi }}}\sin ^{2}{\vartheta }\,e^{-2\mathrm {i} \varphi }}$	${\displaystyle {\sqrt {\tfrac {105}{32\pi }}}\sin ^{2}{\vartheta }\cos {\vartheta }\,e^{-2\mathrm {i} \varphi }}$
m = −1		${\displaystyle {\sqrt {\tfrac {3}{8\pi }}}\sin {\vartheta }\,e^{-\mathrm {i} \varphi }}$	${\displaystyle {\sqrt {\tfrac {15}{8\pi }}}\sin {\vartheta }\,\cos {\vartheta }\,e^{-\mathrm {i} \varphi }}$	${\displaystyle {\sqrt {\tfrac {21}{64\pi }}}\sin {\vartheta }\left(5\cos ^{2}{\vartheta }-1\right)\,e^{-\mathrm {i} \varphi }}$
m = 0	${\displaystyle {\sqrt {\tfrac {1}{4\pi }}}}$	${\displaystyle {\sqrt {\tfrac {3}{4\pi }}}\cos {\vartheta }}$	${\displaystyle {\sqrt {\tfrac {5}{16\pi }}}\left(3\cos ^{2}{\vartheta }-1\right)}$	${\displaystyle {\sqrt {\tfrac {7}{16\pi }}}\left(5\cos ^{3}{\vartheta }-3\cos {\vartheta }\right)}$
m = 1		${\displaystyle -{\sqrt {\tfrac {3}{8\pi }}}\sin {\vartheta }\,e^{\mathrm {i} \varphi }}$	${\displaystyle -{\sqrt {\tfrac {15}{8\pi }}}\sin {\vartheta }\,\cos {\vartheta }\,e^{\mathrm {i} \varphi }}$	${\displaystyle -{\sqrt {\tfrac {21}{64\pi }}}\sin {\vartheta }\left(5\cos ^{2}{\vartheta }-1\right)\,e^{\mathrm {i} \varphi }}$
m = 2			${\displaystyle {\sqrt {\tfrac {15}{32\pi }}}\sin ^{2}{\vartheta }\,e^{2\mathrm {i} \varphi }}$	${\displaystyle {\sqrt {\tfrac {105}{32\pi }}}\sin ^{2}{\vartheta }\cos {\vartheta }\,e^{2\mathrm {i} \varphi }}$
m = 3				${\displaystyle -{\sqrt {\tfrac {35}{64\pi }}}\sin ^{3}{\vartheta }\,e^{3\mathrm {i} \varphi }}$

Applications

Quantum mechanics

As eigenfunctions of the angular component of the Laplace operator, the spherical surface functions are also eigenfunctions of the angular momentum operator for the secondary quantum number as eigenvalue. Therefore, they play a major role in describing atomic states. Furthermore is ${\displaystyle l}$

${\displaystyle {\hat {\mathbf {L} }}^{2}Y_{l,m}(\theta ,\varphi )=\hbar ^{2}l(l+1)Y_{l,m}(\theta ,\varphi )}$

${\displaystyle {\hat {L}}_{z}Y_{l,m}(\theta ,\varphi )=\hbar mY_{l,m}(\theta ,\varphi )}$

Solution of Laplace's equation

For each , the function is the solution of Laplace's equation in three dimensions, because the function just satisfies the above equation ${\displaystyle l}$ ${\displaystyle r^{l}Y_{lm}(\theta ,\varphi )}$ ${\displaystyle R_{l}(r)=r^{l}}$

${\displaystyle \Delta _{r}R_{l}(r)={\frac {l(l+1)}{r^{2}}}R_{l}(r)}$.

Every solution of the Laplace equation can now be uniquely as

${\displaystyle \sum _{l,m}c_{lm}r^{l}Y_{lm}}$

represent. Thus, with the spherical surface functions, the Laplace equation can be solved with spherical Dirichlet boundary conditions : If the boundary conditions fix the value of the solution , which should be defined on the closed unit sphere, to a certain square-integrable function on the unit sphere, then spherical surface functions can be developed which results in the coefficients and thus in an unambiguous way entirely . On the basis of this knowledge of solvability with spherical boundary conditions, the general solvability of the Dirichlet problem of the Laplace equation for sufficiently smooth boundary conditions can be shown; this proof goes back to Oskar Perron . The Dirichlet problem is used in electrostatics and magnetostatics . To solve the Laplace equation, in which a function is sought that is defined outside a sphere and disappears at infinity, under given boundary conditions, the approach is a decomposition ${\displaystyle f}$ ${\displaystyle f|_{S_{2}}}$ ${\displaystyle f|_{S_{2}}}$ ${\displaystyle c_{lm}}$ ${\displaystyle f}$

${\displaystyle \sum _{l,m}c_{lm}r^{-l-1}Y_{lm}}$

possible, which also always provides a solution to the Laplace equation for the given boundary conditions.

See also : Spherical multipole expansion

Nomenclature in geophysics

Spherical surface functions are also used in geophysics . A distinction is made here between:

• zonal ( ): independent of longitude ${\displaystyle m=0}$ ${\displaystyle \varphi }$
• sectoral ( ): ${\displaystyle m=l}$

${\displaystyle Y_{ll}(\vartheta ,\varphi ):={\frac {(-1)}{l!}}^{l}{\sqrt {\frac {(2l+1)!}{4^{l+1}\pi }}}\sin ^{l}\vartheta e^{\mathrm {i} l\varphi }}$

• tesseral (otherwise): depending on latitude and longitude

literature

• Eduard Heine : Handbook of the ball functions Georg Reimer, Berlin 1861, 2nd edition 1878.
• Albert Wangerin : Theory of Potential and Spherical Functions II Volume . Walter de Gruyter, Berlin 1921. ( University of Michigan )
• EW Hobson : The Theory of Spherical and Ellipsoidal Harmonics . Cambridge University Press, 1931, Chelsea 1955
• Josef Lense : spherical functions . Geest u. Portig 1954
• Claus Müller : Spherical Harmonics . Springer Verlag, Lecture Notes in Mathematics No. 17, 1966

Spherical surface functions are also covered in many textbooks in theoretical physics, e.g. B .:

• Arnold Sommerfeld : Lectures on Theoretical Physics , Volume 6 Partial Differential Equations of Physics . Harri Deutsch, 1992
• Claude Cohen-Tannoudji , Bernard Diu, Franck Laloë: Quantum Mechanics 1 . 2nd edition, Walter de Gruyter, Berlin / New York 1999, p. 649 ff.
• Torsten Fließbach : Electrodynamics . 4th edition, Spektrum, Munich 2005, p. 99 ff.

Web links

• Visualization of Spherical Harmonics
• Hydrogen Atom Orbital Viewer

Individual evidence

1. ↑ Albrecht Unsöld: Contributions to the quantum mechanics of atoms . In: Annals of Physics . tape 387 , no. 3 , 1927, pp. 376-377 , doi : 10.1002 / andp.19273870304 .
2. ^ Oskar Perron: A new treatment of the first boundary value problem for Δu = 0 . In: Mathematical Journal . tape 18 , no. 1 . Springer , 1923, ISSN  0025-5874 , p. 42-54 , doi : 10.1007 / BF01192395 .