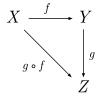Morphism

In category theory (a branch of mathematics ) one considers so-called (abstract) categories , which are given by a class of objects and for two objects and a class of morphisms from to (also referred to as arrows ). ${\ displaystyle X}$ ${\ displaystyle Y}$ ${\ displaystyle X}$ ${\ displaystyle Y}$

One writes:

{\ displaystyle f \ colon X \ to Y}

.

The category also includes a partial combination of morphisms that must meet certain conditions.

If one interprets sets with the same structure as objects and the functions between the underlying sets, which are compatible with their structure , as associated morphisms, one speaks of a concrete category . The combination of the morphisms then corresponds to the usual execution of functions one after the other . But there are also very differently formed concrete categories in which morphisms do not appear as functions between the objects, for example the category Toph , whose objects are topological spaces and whose morphisms are homotopy classes of continuous functions, or the category Rel , whose objects are sets and whose morphisms are relations are.

Examples

Concrete examples of morphisms are homomorphisms of the categories that are studied in algebra (e.g. groups or rings ), continuous functions between topological spaces , differentiable functions between differentiable manifolds .

Each quasi-order defines a category in which the objects are the elements of and a morphism exists if and only if . ${\ displaystyle (M, \ lesssim)}$ ${\ displaystyle M}$ ${\ displaystyle x \ to y}$ ${\ displaystyle x \ lesssim y}$

In a functor category , the morphisms are the natural transformations between the functors.

For some categories there are special names for morphisms.

A homeomorphism is an isomorphism between topological spaces.
A diffeomorphism is an isomorphism between differentiable manifolds.
An isometry is an isomorphism in the category of metric spaces with the non-expanding continuous mappings .
A linear mapping is a (homo-) morphism between vector spaces .

shortcut

The connection (execution, composition) of morphisms, in characters:, is often shown in a commutative diagram , for example ${\ displaystyle \ circ}$

Types

Every object of a category has an identical morphism , written which is a right-neutral element for all morphisms and a left-neutral element of the composition for all morphisms , so that and is always valid. ${\ displaystyle X}$ ${\ displaystyle \ operatorname {id} _ {X} \ colon X \ to X,}$ ${\ displaystyle f \ colon X \ to Y}$ ${\ displaystyle g \ colon Y \ to X}$ ${\ displaystyle f \ circ \ operatorname {id} _ {X} = f}$ ${\ displaystyle \ operatorname {id} _ {X} \ circ g = g}$

If a morphism has a right inverse , i. H. if there is a morphism with are, it means retraction . Similarly, a cut (section, coretraction) denotes a morphism that has a left inverse. ${\ displaystyle f \ colon X \ to Y}$ ${\ displaystyle g \ colon Y \ to X}$ ${\ displaystyle f \ circ g = \ operatorname {id} _ {Y}}$ ${\ displaystyle f}$

If there is both a retraction and a section, then it is called isomorphism . In this case, the objects and can be viewed as similar within their category (isomorphisms are, for example, the bijective images in the concrete category of sets ). ${\ displaystyle f \ colon X \ to Y}$ ${\ displaystyle f}$ ${\ displaystyle X}$ ${\ displaystyle Y}$

A morphism from to is called an endomorphism from ${\ displaystyle X}$ ${\ displaystyle X}$ ${\ displaystyle X.}$

An endomorphism that is also an isomorphism is called an automorphism .

A morphism with the following property is called an epimorphism : ${\ displaystyle f \ colon X \ to Y}$
If there are any morphisms with , then is always (e.g. every surjective homomorphism is an epimorphism). ${\ displaystyle g, h \ colon Y \ to Z}$ ${\ displaystyle g \ circ f = h \ circ f}$ ${\ displaystyle g = h}$

A morphism with the following property is called a monomorphism : ${\ displaystyle f \ colon X \ to Y}$
If there are any morphisms with , then is always (e.g. every injective homomorphism is a monomorphism). ${\ displaystyle g, h \ colon W \ to X}$ ${\ displaystyle f \ circ g = f \ circ h}$ ${\ displaystyle g = h}$

An epimorphism is called extremal if off and is a monomorphism, it always follows: is an isomorphism. ${\ displaystyle f}$ ${\ displaystyle f = v \ circ w}$ ${\ displaystyle v}$ ${\ displaystyle v}$

A monomorphism is called extremal , if from and is an epimorphism, it always follows is an isomorphism. ${\ displaystyle f}$ ${\ displaystyle f = w \ circ v}$ ${\ displaystyle v}$ ${\ displaystyle v}$

If both an epimorphism and a monomorphism, then it is a bimorphism . Not every bimorphism is an isomorphism. However, every morphism is an isomorphism, which is epimorphism and section, or monomorphism and retraction. ${\ displaystyle f}$ ${\ displaystyle f}$
An example of a bimorphism that is not an isomorphism is the embedding of the integers in the rational numbers as a homomorphism of rings.

literature

Martin Brandenburg: Introduction to Category Theory . With detailed explanations and numerous examples. Springer Spectrum, Berlin 2015, ISBN 978-3-662-47067-1 .
Samuel Eilenberg , Saunders Mac Lane : General theory of natural equivalences . In: Transactions of the American Mathematical Society . tape 58 , no. 2 , September 1945, p. 231-294 .
Saunders Mac Lane: Categories for the Working Mathematician ( Graduate Texts in Mathematics . Volume 5 ). 2nd Edition. Springer, New York 1998, ISBN 0-387-90035-7 .