Fourteen

A regular fourteenth corner

A fourteen- sided or tetradecagon is a polygon with 14 sides and 14 corners. Often a flat, regular fourteenth corner is meant with all sides of the same length and all corner points on a common circumference .

Regular fourteen-sided

According to Carl Friedrich Gauß and Pierre-Laurent Wantzel, the regular fourteenth corner is not a constructible polygon , because its number of sides is not a product of a power of two with pairwise different Fermat's prime numbers . ${\displaystyle n=14}$

Sizes

Sizes of a regular fourteen-sided
Interior angle	${\displaystyle {\begin{aligned}\alpha &={\frac {n-2}{n}}\cdot 180^{\circ }={\frac {12}{14}}\cdot 180^{\circ }\\\alpha &=154{,}285714^{\circ }\end{aligned}}}$
Central angle (Center angle)	${\displaystyle {\begin{aligned}\mu &={\frac {360^{\circ }}{14}}\\\mu &=25{,}714285^{\circ }\end{aligned}}}$
Side length	${\displaystyle {\begin{aligned}a&=R\cdot 2\cdot \sin \left({\frac {180^{\circ }}{14}}\right)\\a&\approx 0{,}4450418\cdot R\end{aligned}}}$
Perimeter radius	${\displaystyle {\begin{aligned}R&={\frac {a}{2\cdot \sin \left({\frac {180^{\circ }}{14}}\right)}}\\R&\approx {\frac {a}{0{,}4450418}}\end{aligned}}}$
Inscribed radius	${\displaystyle {\begin{aligned}r&=R\cdot \cos \left({\frac {180^{\circ }}{14}}\right)\\r&\approx 0{,}9749279\cdot R\end{aligned}}}$
height	${\displaystyle {\begin{aligned}h&=2\cdot r\\h&\approx 1{,}9498558\cdot R\end{aligned}}}$
Area	with side length ${\displaystyle a}$ ${\displaystyle {\begin{aligned}A&={\frac {7}{2}}a^{2}\cot {\frac {\pi }{14}}\\&={\frac {7}{6}}a^{2}\left({\sqrt {7}}+4{\sqrt {7}}\cos \left({{\frac {2}{3}}\arctan {\frac {\sqrt {3}}{9}}}\right)\right)\\A&\approx 15{,}3345\cdot a^{2}\end{aligned}}}$ with perimeter radius ${\displaystyle R}$ ${\displaystyle {\begin{aligned}A&=14\cdot R^{2}\cdot \sin \left({\frac {180^{\circ }}{14}}\right)\cdot \cos \left({\frac {180^{\circ }}{14}}\right)\\A&\approx 3{,}03718617\cdot R^{2}\end{aligned}}}$

Mathematical relationships

Interior angle

The interior angle is enclosed by two adjacent side lengths . ${\displaystyle \alpha }$ ${\displaystyle a}$

${\displaystyle \alpha ={\frac {n-2}{n}}\cdot 180^{\circ }={\frac {14-2}{14}}\cdot 180^{\circ }={\frac {12}{14}}\cdot 180^{\circ }=154{\frac {4}{14}}^{\circ }}$.

Central angle

The central angle or center angle is enclosed by two adjacent circumferential radii . ${\displaystyle \mu }$ ${\displaystyle R}$

${\displaystyle \mu ={\frac {360^{\circ }}{n}}={\frac {360^{\circ }}{14}}=25{\frac {10}{14}}^{\circ }}$.

Side length

The side length is calculated ${\displaystyle a}$

${\displaystyle a=R\cdot 2\cdot \sin \left({\frac {180^{\circ }}{14}}\right)}$.

Perimeter radius

The radius of the perimeter is obtained by transforming the formula for the side length . ${\displaystyle R}$ ${\displaystyle a}$

${\displaystyle R={\frac {a}{2}}\cdot \csc \left({\frac {180^{\circ }}{14}}\right)}$.

Inscribed radius

The incircle radius is the height of an isosceles partial triangle with the two legs equal to the circumferential radius and the base line equal to the side length . ${\displaystyle r}$ ${\displaystyle R}$ ${\displaystyle a}$

${\displaystyle {\begin{aligned}r&=R\cdot \cos \left({\frac {\mu }{2}}\right)=R\cdot \cos \left({\frac {180^{\circ }}{14}}\right)\end{aligned}}}$.

height

The height of a regular fourteenth corner results from the sum of two inscribed radii ${\displaystyle h}$ ${\displaystyle r}$

${\displaystyle h=2\cdot r}$.

Area

Using the side length ${\displaystyle a}$

The area of ​​a regular fourteen-sided triangle is given by the formula ${\displaystyle a}$

${\displaystyle {\begin{aligned}A&={\frac {7}{2}}a^{2}\cot {\frac {\pi }{14}}={\frac {7}{6}}a^{2}\left({\sqrt {7}}+4{\sqrt {7}}\cos \left({{\frac {2}{3}}\arctan {\frac {\sqrt {3}}{9}}}\right)\right)\end{aligned}}}$.

Using the perimeter radius ${\displaystyle R}$

The area of ​​a triangle is generally calculated . The results of the side length and the incircle radius are used to calculate the fourteen- sided , which is used for the height . ${\displaystyle A_{\Delta }={\frac {1}{2}}a\cdot h_{a}}$ ${\displaystyle a}$ ${\displaystyle r}$ ${\displaystyle r}$ ${\displaystyle h_{a}}$

${\displaystyle a=R\cdot 2\cdot \sin \left({\frac {180^{\circ }}{14}}\right)}$

${\displaystyle h_{a}=r=R\cdot \cos \left({\frac {180^{\circ }}{14}}\right)\;}$ from this follows for the area of ​​a partial triangle

${\displaystyle {\begin{aligned}A_{\Delta }&={\frac {1}{2}}\cdot R\cdot 2\cdot \sin \left({\frac {180^{\circ }}{14}}\right)\cdot R\cdot \cos \left({\frac {180^{\circ }}{14}}\right)\end{aligned}}\;}$ summarized it results

${\displaystyle A_{\Delta }=R^{2}\cdot \sin \left({\frac {180^{\circ }}{14}}\right)\cdot \cos \left({\frac {180^{\circ }}{14}}\right)}$

and for the area of ​​the entire fourteenth corner

${\displaystyle A=14\cdot A_{\Delta }=14\cdot R^{2}\cdot \sin \left({\frac {180^{\circ }}{14}}\right)\cdot \cos \left({\frac {180^{\circ }}{14}}\right)}$.

Geometric constructions

A regular fourteenth corner cannot be represented as a construction with compasses and ruler alone ; it is not a constructible polygon . However, if you add an additional aid to these classic (Euclidean) tools, such as B. a tomahawk to divide the angle into three or a ruler with a certain mark, an exact construction is possible. Basically, from the construction of a heptagon , z. B. by halving the central angle, a regular fourteenth corner can be obtained.

Tomahawk as an additional tool

Andrew M. Gleason published in 1988 in the mathematical magazine The American Mathematical Monthly two elegant structures to the regular polygons heptagon and Dreizehneck who need to solve a tripartite division of the angle. The principle of tripartite division is not specified in either of the two constructions.

Fig. 1: Construction of the fourteenth corner , a modification of the construction of the heptagon with tomahawk .		animation

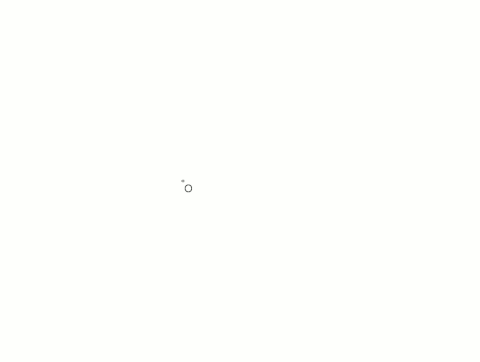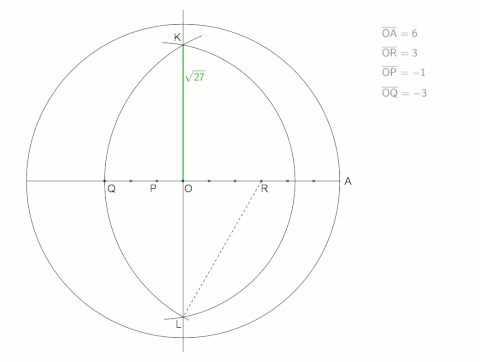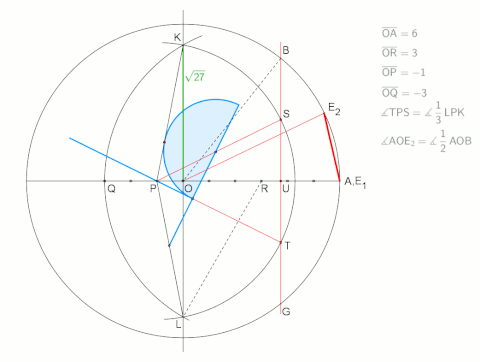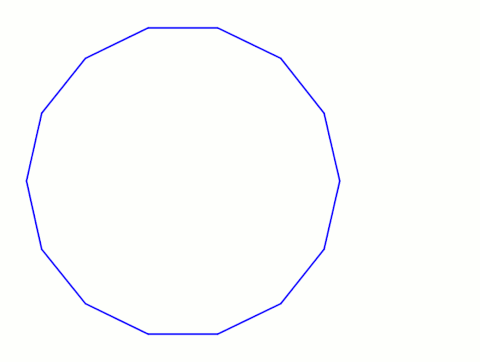
With a given radius (see picture 1)

The following construction plan for the fourteenth is almost identical to the original of the heptagon by Andrew M. Gleason:

It begins in the coordinate origin of a Cartesian coordinate system at the point with a circle with radius. The points and are then determined . Then the points and are determined, they are corner points of two equilateral triangles with a base . After connecting the dots and using the original drawing from The American Mathematical Monthly magazine, see itemization, this point is between and , drawn around an arc from to . Now divide the angle into thirds with a freely selectable method (e.g. curves, tomahawk, etc.), resulting in the points and . A straight line through and results in and , which together with the vertices of a regular heptagon. ${\displaystyle O}$ ${\displaystyle 6.}$ ${\displaystyle A(6,0),P(-1,0),Q(-3,0)}$ ${\displaystyle R(3,0)}$ ${\displaystyle K(0,{\sqrt {27}})}$ ${\displaystyle L(0,-{\sqrt {27}})}$ ${\displaystyle {\overline {QR}}}$ ${\displaystyle K}$ ${\displaystyle L}$ ${\displaystyle P}$ ${\displaystyle (}$ ${\displaystyle P}$ ${\displaystyle O)}$ ${\displaystyle P}$ ${\displaystyle K}$ ${\displaystyle L}$ ${\displaystyle LPK}$ ${\displaystyle S}$ ${\displaystyle T}$ ${\displaystyle S}$ ${\displaystyle T}$ ${\displaystyle B}$ ${\displaystyle G}$ ${\displaystyle G}$

Now you need to halve the central angle of the heptagon and you get the second corner point of the fourteenth you are looking for. The other corner points can be found one after the other by using the circular arc . ${\displaystyle AOB}$ ${\displaystyle E_{2}}$ ${\displaystyle OAE_{2}}$

Marked ruler as an additional aid

Fig. 2: Regular fourteen-sided, continuation of the Neusis construction according to David Johnson Leisk (Crockett Johnson), for the heptagon see animation

David Johnson Leisk, usually known as Crockett Johnson , published in 1975 a so-called Neusis construction of a heptagon (Heptagon), in which the side length is given. For this he used a compass and a special ruler with a mark on it.

With a given side length (see Figure 2)

• Build perpendicular to the page length in point of the route , it is the same length as the side length ${\displaystyle {\overline {E_{1}E_{2}}}}$ ${\displaystyle E_{1}}$ ${\displaystyle {\overline {E_{1}I}}}$ ${\displaystyle {\overline {E_{1}E_{2}}}.}$
• Connect the point with z. B. for one side , the diagonal has the value ${\displaystyle E_{2}}$ ${\displaystyle I,}$ ${\displaystyle {\overline {E_{1}E_{2}}}=1}$ ${\displaystyle {\sqrt {2}}.}$
• Halve the length of the side , you get the point ${\displaystyle {\overline {E_{1}E_{2}}}}$ ${\displaystyle H.}$
• Make a perpendicular to the length of the side in the point ${\displaystyle {\overline {E_{1}E_{2}}}}$ ${\displaystyle H.}$
• Draw the arc with the radius around the point and through the point ${\displaystyle a}$ ${\displaystyle {\overline {E_{2}I}}}$ ${\displaystyle E_{2}}$ ${\displaystyle I.}$
• Place the ruler marked with the point (distance from corner of ruler to point corresponds to ) on the drawing. Turn and push the ruler until its corner to the perpendicular bisectors is applied, the marker point on the circular arc lies and the edge of the ruler through the point runs, it results in the point ${\displaystyle J}$ ${\displaystyle J}$ ${\displaystyle {\overline {E_{1}E_{2}}}}$ ${\displaystyle J}$ ${\displaystyle a}$ ${\displaystyle E_{1}}$ ${\displaystyle O.}$
• Connect the point with the point , the resulting angle , denoted by (theta), corresponds to a quarter of the central angle of a heptagon, due to the 2nd theorem of rays it also corresponds to half of the sought central angle of the fourteenth. ${\displaystyle E_{1}}$ ${\displaystyle O}$ ${\displaystyle E_{1}OH}$ ${\displaystyle \theta }$
• Draw a circle around the point, it is the circumference of the resulting fourteenth corner. ${\displaystyle O}$ ${\displaystyle E_{1},}$
• Determine the remaining twelve corner points of the heptagon with the side length and finally connect the neighboring corner points with each other. This creates the regular fourteenth corner ${\displaystyle {\overline {E_{1}E_{2}}}}$ ${\displaystyle E_{1}\dots E_{14}.}$

Approximation construction

Image 3: Fourteen-sided, approximate construction with a universal method

Figure 3 shows a fourteenth-corner in its circumference, created with a universal method.

First, the distance , and later the diameter of the fourteenth to be searched for, is divided into parts of equal length using the theorem of rays (not shown in the drawing) or determined by lining up distances of equal length. Either the even or the odd numbers (division points) are marked on. In this example, the odd numbers and are entered. B. the later corner points and not near the point . The dividing point corresponds to the center point of the diameter. Now it is drawn around the center point and through the circumference. The two following arcs around or with the radius intersect at the points and. After they are connected, the central axis is obtained and the corner points and the resulting fourteen-sided as intersection points . ${\displaystyle {\overline {AB}}}$ ${\displaystyle 14}$ ${\displaystyle 14}$ ${\displaystyle {\overline {AB}}}$ ${\displaystyle 1,3,5,7,9,11}$ ${\displaystyle 13}$ ${\displaystyle E_{5}}$ ${\displaystyle E_{6}}$ ${\displaystyle B}$ ${\displaystyle 7}$ ${\displaystyle O}$ ${\displaystyle {\overline {AB}}.}$ ${\displaystyle O}$ ${\displaystyle A}$ ${\displaystyle A}$ ${\displaystyle B}$ ${\displaystyle {\overline {AB}}}$ ${\displaystyle C}$ ${\displaystyle D.}$ ${\displaystyle CD}$ ${\displaystyle E_{2}}$ ${\displaystyle E_{9}}$

It continues with the definition of the corner points on the perimeter. The ruler is placed at the point and at the odd number . Then a line is drawn along the ruler to the opposite half of the perimeter line, results in the corner point. This procedure is repeated when determining the corner point and starting from the point when defining the corner points and the corner point is connected with . Thus, the segment is the first approximately constructed side length of the fourteen-sided sought ${\displaystyle C}$ ${\displaystyle 5}$ ${\displaystyle E_{1}.}$ ${\displaystyle E_{3}}$ ${\displaystyle D}$ ${\displaystyle E_{8}}$ ${\displaystyle E_{10.}}$ ${\displaystyle E_{1}}$ ${\displaystyle E_{2}}$ ${\displaystyle {\overline {E_{1}E_{2}}}}$ ${\displaystyle a}$

First, the missing corner points are to be determined on the lower half of the circumference in the order and by repeatedly removing the side length and then the still missing corner points in the sequence and on the upper half of the circumference. Finally, the neighboring corner points are connected to one another. ${\displaystyle E_{4},}$ ${\displaystyle E_{5},}$ ${\displaystyle E_{14}}$ ${\displaystyle E_{13}}$ ${\displaystyle a}$ ${\displaystyle E_{7},}$ ${\displaystyle E_{6},}$ ${\displaystyle E_{11}}$ ${\displaystyle E_{12}}$

Two of the sides of this fourteenth are the same length, but one is different from the other; these are the sides and the other twelve side lengths are the same length. ${\displaystyle {\overline {E_{5}E_{6}}}}$ ${\displaystyle {\overline {E_{12}E_{13}}}.}$

Largest and smallest absolute error of the side lengths for a circumferential radius with : ${\displaystyle R=1\;\mathrm {m} }$

${\displaystyle {\overline {E_{5}E_{6}}}}$ and ${\displaystyle {\overline {E_{12}E_{13}}}\approx -2{,}7\;\mathrm {mm} }$

The remaining twelve side lengths ${\displaystyle \approx 0{,}5\;\mathrm {mm} }$

Regular overturned fourteen-sided

A regular overturned fourteenth corner results if at least one is skipped over each time when connecting the fourteen corner points and the chords thus created are of the same length. Such regular stars are noted with Schläfli symbols , indicating the number of corner points and connecting every -th point. ${\displaystyle \left\{n/k\right\}}$ ${\displaystyle n}$ ${\displaystyle k}$

There are only two regular fourteen-pointed stars.

The star with the symbols {14.2} and {14/12} are regular Seven corner , {14.4} and {14/10} and {14.6} and {14.8} are regular Heptagramme .

• Regular fourteen-pointed stars
• 

  ${\displaystyle \left\{14/3\right\}{,}\ \left\{14/11\right\}}$

• 

  ${\displaystyle \left\{14/5\right\}{,}\ \left\{14/9\right\}}$

literature

• H. Maser: The division of the circle ..., Article 365. , in Carl Friedrich Gauss' investigations on higher arithmetic , published by Julius Springer, Berlin 1889; Göttingen Digitization Center, University of Göttingen; accessed on March 15, 2018.

Web links

Commons : Regular fourteen-sided  collection of images, videos, and audio files

Individual evidence

1. ↑ Andrew Gleason : Angle Trisection, the Heptagon, and the Triskaidecagon . In: The American Mathematical Monthly . tape 95 , no. 3 , 1988, pp. 185–194 , 186 ff. , JSTOR : 2323624 ( math.fau.edu, FIG.1. Construction of a regular heptagon [PDF; 303 kB ; accessed on May 21, 2019]).
2. ^ Weisstein, Eric W. "Heptagon." From MathWorld, A Wolfram Web Resource.
3. ^ H. August: Drawing construction of an elf. In: Drawing constructions: polygons. Retrieved January 3, 2018 . 
4. ^ Peter Eckardt: Siebeneck. In: Stars and Polygons. Retrieved January 3, 2018 .