Transfer (materials science)
The dislocation is a disturbance of the periodicity of a crystal lattice that continues along a line in the crystal . It can arise, for example
- during crystal growth (e.g. from the melt or in vapor deposition),
- in the existing crystal as a result of internal stresses
- or with plastic deformation . The plastic deformation of crystals takes place with the creation and movement of dislocations (Fig. 1) or through the formation of twins .
The dislocation cannot end inside a crystal. A distinction is traditionally made between two idealized models of dislocations: the step dislocation and the screw dislocation. An important feature for characterizing a dislocation is the Burgers vector.
Idealized basic models
There are two basic models of dislocations that allow the theoretical determination of the dislocation energy from the distortion of the crystal lattice. The idealization consists primarily in the assumption that the dislocation line is straight.
Step shift
Burgers vector and dislocation line are perpendicular to each other. However, this only applies to crystal lattices in which right angles occur due to higher symmetry, not in the triclinic crystal lattice. A step dislocation can be imagined as an additional half-plane of particles ( atoms , ions ) which has no continuation in a perfect crystal. The English name for the step dislocation is edge dislocation . The Russian name is краевая дислокация , German: Randversetzung. The place where this half-plane ends is called the dislocation kernel or dislocation line. There, the dislocation causes the strongest distortion of the grating, which results in a distortion field around the dislocation line (Fig. 2).
The energy of a step displacement per unit length is
with G = shear modulus .
Screw dislocation
Burgers vector and dislocation line are parallel. A screw dislocation creates a helical relationship between all the grid planes it cuts.
The energy of a screw dislocation per unit length is lower than with a step dislocation:
The typical dislocation ring therefore has more areas with a screw character than with a step character and has an elliptical shape .
properties
Each dislocation has two important parameters: the dislocation line and the Burgers vector.
Burgers vector
The Burgers vector (named after Johannes Martinus Burgers ) describes the direction in which the dislocation movement necessarily occurs. Its magnitude corresponds to the distance between two neighboring atoms in this direction. Its direction is dictated by the crystal structure of the material.
The Burgers vector can be generated using the following procedure (Figure 5):
- With a little distance to the dislocation, a connection is drawn between the atoms, so that a closed circuit is created. This is the Burgers circulation , represented by the dashed line in the left picture.
- Now the circulation of the left image is transferred 1: 1 to the right image of an undisturbed crystal.
- At one point the circulation cannot be closed.
- The connection needed to close the circuit is the Burgers vector .
It leads to an analogous result if the closed circuit is constructed in the perfect lattice and is transferred to the vicinity of the dislocation line. The Burgers vector with the lowest energy (grows with the square of its amount) lies in densely packed planes:
- In a face-centered cubic lattice, these are the <110> directions in the {111} planes.
- In a body-centered cubic lattice these are the <111> directions in the {110} planes. However, the {112} and {123} planes are only slightly less densely packed and can therefore also appear as slip planes.
The energy of a crystal can drop if the dislocation splits into two partial dislocations, each with only half the size of the Burgers vector. In the automotive grid , the Shockley dislocations are particularly interesting. By combining two Shockley dislocations, a so-called Lomer-Cotrell dislocation can then be formed, which brings about a further reduction in energy. Lomer-Cotrell dislocations are often "sedentary", so they cannot move any further because they lie in a different plane than the initial dislocation.
Visualization
The grid distortions around a dislocation line can be visualized using a number of methods. In principle, these are also suitable for determining the dislocation density ρ (see there).
- etching
- IR microscopy
- Transmission Electron Microscopy (TEM)
- Field Ion Microscopy (FIM)
- X-ray topography
Furthermore, using high-resolution transmission electron microscopy (HR-TEM) in steel (Fig. 6) and some semiconductors, it has recently been possible to make dislocation nuclei visible in almost atomic resolution.
Dislocation movement as an explanation of plasticity
Formerly used concept of theoretical strength
Until the 1930s, explaining the plasticity and strength of metals on a microscopic level was a major challenge . In a “defect-free” crystal, the theoretical strength is described by the expression
= Shear modulus .
This estimate results from considerations of the displacement resistance of two superimposed atomic layers. However, the values actually observed are many times below this estimate for practically all metals . With body-centered cubic crystal lattices, the theoretical strength is missed by a factor of 100, with face-centered and hexagonal lattices by a factor of 1,000 to 10,000.
Discovery and description of dislocations
In 1934 Egon Orowan , Michael Polanyi and GI Taylor described independently and at about the same time how this contradiction can be resolved by the relocation concept. In the 1950s, their concept was first experimentally confirmed with the help of newly developed electron microscopes that could make dislocations visible.
When determining the theoretical strength, it was assumed that all atoms in an atomic layer would have to overcome the displacement resistance at the same time. According to the dislocation concept, however, this is not the case: under the effect of a very small shear stress compared to the theoretical strength , dislocations can "move", i.e. That is, the atoms of the neighboring half-plane break their bonds briefly and attach to those of the next half-plane. The dislocation line “wanders”. This is the elementary mechanism of plastic deformation. It only ever happens in those slip planes in which the Burgers vector also lies. Except in the case of pure screw dislocations , which can also slide transversely , the slip plane is already fixed by the position of the dislocation.
Dislocation multiplication
The movement of a dislocation line can be perturbed by interaction with voids , other dislocations, or precipitates such as carbides . This hinders the sliding process. If several dislocations run into each other, the stress fields overlap and cause the dislocation to bulge between two obstacles. The subsequent transfers now take the places of the previous transfers. A so-called Frank-Read source is created (Fig. 7), which can emit over one hundred new dislocations in real crystals. The dislocation multiplication leads to work hardening .
The work hardening is irreversible as long as the temperature remains below approx. 30% of the absolute melting temperature T m (in Kelvin ). In addition, the dislocations can heal ( crystal recovery ) through recombination or arrangement of the dislocations with one another, whereby the strength drops again significantly and the deformability increases. At even higher temperatures, the dislocations are eliminated by the formation of new structures during recrystallization annealing .
Dislocations in semiconductors
In the semiconductor industry , single crystals with as little dislocation as possible are required, since otherwise the electronic properties of the crystals would be disturbed.
Electronic silicon is normally dislocation-free, regardless of the zone-floating and Czochalski growth processes. In the case of germanium, the dislocation density depends very much on the process, especially in the case of optical components for infrared optics, it can be high, but low-dislocation to dislocation-free germanium is also grown. In the case of gallium arsenide (GaAs), the crosslinking density depends heavily on the growth process and doping. Growing by means of "Vertical Gradient Freezing" (VGF) enables dislocation densities between 10 cm −2 (silicon doping) and 10 3 cm −2 (undoped). Undoped crystals with a diameter of 150 mm grown using liquid encapsulated Czochalski (LEC) show dislocation densities in the range of 10 5 cm −2 . Indium-doped GaAs: In (approx. 0.1–1% indium) is almost free of dislocations. Other compound semiconductors (InP, GaP, InAs) are in a similar range. At dislocation densities above 10 6 cm −2 , the transition to polycrystalline growth is mostly observed.
In the initial phases of heteroepitaxy on sapphire or silicon, GaN and AlN show dislocation densities of up to 10 9 cm −2 . Then, however, a sequence of layers is selected that drastically lowers it so that values below 10 7 cm −2 are achieved in the active area of the component . Freely growing crystals again have a lower dislocation density (AlN growth by means of gas phase transport approx. 10 3 cm −2 ,).
In single crystals, the dislocations occur primarily through thermal stresses in the material during the cooling process , in semiconductor heterolayer systems mostly through a lattice mismatch . Single crystals with as few dislocations as possible are therefore obtained by gentle cooling.
Example in 2 dimensions
In two dimensions, only the step dislocation exists, which has a central role in melting in 2D, but not the screw dislocation.
These dislocations form topological point defects ; H. they cannot be generated individually locally by an affine transformation . They can only arise in pairs with an antiparallel burger vector . Are many dislocations e.g. B. exists due to thermal fluctuations, the discrete translation order of the crystal is destroyed, at the same time the shear and elasticity module disappear , i.e. H. the crystal has melted into a liquid phase . The orientation order has not yet been destroyed, however, and one finds - similar to liquid crystals - an anisotropic phase with typically six-fold directional symmetry , known as the hexatic phase . Only the presence of disclinations (Fig. 8) creates an isotropic liquid. This two-stage melting process using dislocations and disclinations is described in the KTHNY theory .
Dislocations in triclinic crystals
The consideration of dislocations in a crystal lattice without right angles leads to a new perspective and offers the possibility to recognize more precisely which statements about dislocations are generally valid. This is done on the basis of a comprehensive investigation of the plastic deformation of crystals of two isomorphic triclinic TCNQ complex salts as model bodies. These are molecular crystals of the substances triphenylmethylphosphonim-bis-7.7.8.8. Tetracyanoquinodimethanide (short: [Ph]) and triphenylmethylarsonime-Bis-7.7.8.8. Tetracyanoquinodimethanide (short: [As]), which are interesting because of the extraordinarily strong anisotropy of their electrical conductivity.
By chance it was found that the impressions of bronze wires, which served as measuring electrodes, were surrounded by sliding steps - a sure sign of plastic deformation (Fig. 9).
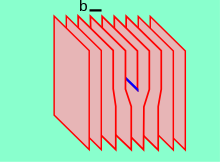
How the creation of a sliding step is related to the movement of a dislocation can be shown using the schematic representation of the local deformation of a two-dimensional lattice (Fig. 10). When the upper part of the grid is pressed from left to right, it is shifted from the surface by g , forming a sliding step , with a disturbance of the periodicity occurring inside at X - a dislocation (b). If one transfers the orbit ABCD from the undisturbed grid (a) into the disturbed grid, then the grid vector b = g is required to completely close the circulation . Where b is the Burgers vector.
If the dislocation moves to the right edge (c), the slip is complete and a slip step occurs there too. The movement of the dislocation takes place in the slip plane to which it is bound. After a complete slip, the crystal is free of dislocations.
If pressure is exerted on the upper part from right to left, a sliding step first occurs on the right side and a dislocation (d) inside. After complete slipping, the dislocation left the crystal and a sliding step (e) is also created there.
Because there are no right angles in the grid , the term step dislocation only makes sense here as the edge of an additional grid plane, and the steps in (c) and (e) have a different profile: in (c) they are obtuse-angled, in ( e) acute-angled.
In the schematic representation of an elementary slip step (Figure 11), which was created by the local displacement of a part of the crystal around the lattice vector g (slip vector), a Burgers cycle around the endpoints of the slip step shows that there is a displacement with the Burgers vector b = + g intersects the surface and at the same time that the center of a helical relationship between neighboring lattice planes is located there. This lattice disruption must continue into the interior of the crystal along a line. How this line runs cannot be said. In the case of curve L, the displacement began on the surface and the dislocation migrated into the crystal. In the course of L ', a dislocation coming from inside the crystal has reached the surface. The creation of a helical connection of lattice planes does not require that the dislocation line is oriented parallel to the Burgers vector, as suggested by the traditional definition of screw dislocation.

The TEM photo of the slip step field next to a needle prick on the (100) surface of an [As] crystal (Fig. 12) shows that plastic deformation results in multiple slip steps, in which slip is repeatedly activated in the same slip plane. This speaks in favor of the dislocation course along L in Figure 11, because it can be considered unlikely that random dislocations that occur during crystal growth are in an orderly manner in the same slip plane.
Step dislocations also create a helical relationship between neighboring lattice planes if these are intersected by the dislocation line and are not oriented parallel to the Burgersvekror. Figure 13 shows this situation as a projection of a step offset onto the slip plane in the drawing technique practiced by Read. The filled points are in front of the slip plane, the open circles behind it. On the right edge of the graphic you can see the flank of a sliding step. Two burger circuits with different orientations are drawn in, both of which lead to the same burger vector b . The circuit, which runs obliquely to the Burgers vector from bottom left to top right, leads to an adjacent lattice plane and indicates a helical relationship created by the displacement.
The following applies in general: Every dislocation creates a helical relationship between lattice planes that are intersected by it and are not oriented parallel to the Burgers vector.
Proof of dislocation by etching
At the intersection of a dislocation with the crystal surface, attack by a solvent is favored. The dissolving process is carried out along the dislocation line in depth, with an etching pit being created with a point marking the location of the dislocation. This can be used to detect dislocations and their distribution on the crystal surface.
Figure 14 shows an example. Here the surface of the [As] crystal was partially dissolved / etched by the solvent acetonitrile. In order to avoid a coarse dissolving process, it was ensured that the solvent could only act for a very short time (about 0.1 s). Shown is the environment of a microhardness -Eindrucks, in the image 14 a and the Gleitstufenfeld in Figure 14 b the corresponding etched pattern. The etching pits mark the beginning of each sliding step and each point at which the sliding step height increases or decreases by one grid plane distance (here 1.5 nm) through repeated use of the same sliding plane. The beginning of the visibility of a sliding step is marked by an arrow in (a), the third etching pit at the same point in (b). The visibility of the sliding step in the incident light microscope with oblique illumination therefore began at a step height of 4.5 nm. The row of numerous etching pits along the sliding steps shows that the multiple use of identical sliding planes due to dislocations produced one after the other with continuous loading is the rule.
The schematic representation of a quadruple sliding step (Fig. 15) shows how the dislocations create a helical relationship between the lattice planes they cut. In the section furthest from the surface, the dislocations run parallel to the lattice planes and do not create a helical relationship there. There you have the orientation of a step shift.
See also
literature
- JM Burgers: Some considerations on the fields of stress connected with dislocations in a regular crystal lattice. In: Proceedings of the Section of Sciences. Koninklijke Nederlandse Akademie van Wetenschappen. Vol. 42, 1939, ISSN 0370-0348 , pp. 293-325, 378-399.
- Jacques Friedel: Les dislocations (Paris, Gauthier Villars, 1956, 2nd ed.Dislocations, Pergamon, 1964)
- Derek Hull, David J. Bacon: Introduction to Dislocations (= International Series on Materials Science and Technology. Vol. 37). 3rd edition. Pergamon Press, Oxford et al. 1984, ISBN 0-08-028720-4 .
- John P. Hirth, Jens Lothe: Theory of dislocations. 2nd edition. Wiley, New York NY et al. 1982, ISBN 0-471-09125-1 .
- Hagen Kleinert : Gauge Fields in Condensed Matter. Disorder Fields and Applications to Superfluid Phase Transition and Crystal Melting. Volume 2: Stresses and Defects. Differential Geometry, Crystal Melting. World Scientific, Singapore et al. 1989, ISBN 9971-5-0210-0 , pp. 743-1456 ( readable online on the author's website ).
R. Fornari (Editor) Single crystals in electronic materials, Elsevier Ltd, ISBN 978-0-08-102096-8 (2019)
Individual evidence
- ↑ a b c d e Christoph Broeckmann, Paul Beiss: Materials Science I . Institute for Material Applications in Mechanical Engineering at RWTH Aachen University , Aachen 2014, pp. 162–182.
- ^ E. Orowan, Zeitschrift für Physik Vol. 89 (1934) pp. 605, 614, 634.
- ↑ M. Polanyi, Zeitschrift für Physik Vol. 89 (1934) p. 660.
- ^ GI Taylor, Proc. Royal Society London 1934 pp. 145, 362, 388.
- ↑ U. Gasser, C. Eisenmann, G. Maret, P. Keim, ChemPhysChem Vol. 11 (2010) pp. 963-970.
- ↑ U. Gasser, G. Maret, P. Keim, Physik in our Zeit Vol. 39 (2008) p. 36.
- ↑ Heinz HW Preuß :, Tricline TCNQ complex salts as a model body for the investigation of crystal plasticity with low symmetry, Dissertation B (habilitation thesis), Leipzig 1977 . In: The Rector of the Bergakademie Freiberg (Hrsg.): Freiberger research booklet . B 204. VEB Deutscher Verlag für Grundstofftindustrie, Leipzig 1978.
- ^ Müller E., Ritschel H., Hansel H., 33 (): Relocation . In: Physica status solidi . tape 33 , 1969, p. K 55 .
- ↑ Read, WT Jr .: Dislocations in Crystals . London 1953.