Well-tempered mood

from Wikipedia, the free encyclopedia

As well temperament is called a tempered mood system for instruments with fixed pitch ( piano , organ , harp , which in contrast to u. A.) Pure or mean tone that only a limited number of keys make available, unrestricted use of all keys of the circle of fifths allows . The most widespread variant of the well-tempered tunings today is the equal tuning , in which, however, the specific character of the keys is lost. Therefore, in today's linguistic usage, the term well-tempered is often only related to (non-equal) historical tuning systems ( Werckmeister , Kirnberger , Vallotti and others) that preserve the key character .

Derivation of the term

The verb tempering comes from the Latin temperare , which means something like "correctly measured"; In music it means that intervals are deliberately tuned a little impure, so that small pitch differences such as the syntonic and Pythagorean commas are distributed and thus no longer appear disturbing. These pitch differences, as a result of the fundamental mismatch of stacked fifths and thirds in the octave space, prevent transposing into any key with the usual twelve keys when the tuning is pure and require many additional keys, such as the 31-step archicembalo by Nicola Vicentino , the Mean- tone tuning usual at the time, extended to be transposable. Werckmeister condemned such instruments as "patchwork of the subsemitonies".

history

Under the collective name of well-tempered moods , Andreas Werckmeister introduced a series of moods on keyboard instruments from 1681 , which expanded the mean-tone moods so that the keys of the entire circle of fifths could be played. Previously impossible transpositions and enharmonic mix-ups were made possible. In individual cases, the organ builder Christian Förner had already developed a mood that made it possible to play in all keys. This tuning is documented for the first time for the Förner organ of the castle church in Weißenfels , built between 1668 and 1673 . Zacharias Thayßner , a student of Christian Förner, built the organ of the collegiate church of St. Servatii in Quedlinburg from 1677 to 1682 , where Werckmeister had held office since 1675, and in the 1677 contract he promised a temperature that could be used in all keys.

In the decades that followed, Förner's students Zacharias Thayßner , Christoph Junge and Tobias Gottfried Trost, as well as Förner's grandchildren, including Tobias Heinrich Gottfried Trost and Johann Friedrich Wender , created further organs with modified mean-tone (without wolf fifth ) or well-tempered organs in Central Germany . Johann Friedrich Wender built the organ of Divi Blasii in Mühlhausen from 1687 to 1691 and the organ of the Bonifatius Church (today Bach Church ) in Arnstadt from 1699 to 1703 . The young Johann Sebastian Bach officiated on these two organs from 1703 to 1708. They made it possible to compose organ works that go beyond the keys that are permitted by the ¼ point mean pitch. Some organ works by Dietrich Buxtehude , which require a well-tempered tuning, are likely to be related to the introduction of a well-tempered tuning by the Förner School: Buxtehude was friends with Andreas Werckmeister and can be shown to have sent him a large number of his organ works. He could have written some of these works especially for Werckmeister and his well-tempered Thayßner organ. According to another opinion, Buxtehude never had a well-tempered organ at his disposal. The first documented cases of changes to “new temperatures” in northern Germany did not occur until around 1740.

Many other theorists and practitioners, such as Johann Georg Neidhardt , Johann Philipp Kirnberger , Francesco Antonio Vallotti , kept making new suggestions for temperature control , which shows that there was no clear solution at the time. On the one hand, one wanted to approximate or actually obtain pure thirds for the most frequently used keys; on the other hand, the circle of fifths had to be closed without a wolf fifth . Each system had its advantages and disadvantages in terms of sound and was more or less complex in the (then) craftsmanship.

Characteristic

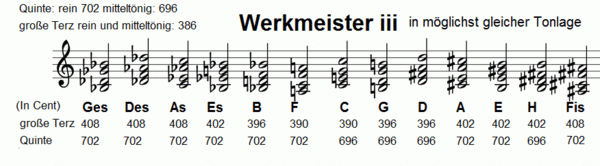
It is characteristic of well-tempered tunings that all important intervals deviate from the pure tuning in such a way that the keys that are frequently played contain purer thirds and the distant keys are sharper. Werckmeister's famous mood Werckmeister III has found widespread use. It contains different sounding keys from a mid-tone sounding C major ( listening ? / I ) to a Pythagorean sounding F sharp major ( listening ? / I ) - a property that must be given in a Werckmeister “good mood”. This means that this tuning creates a distinct key characteristic that is strived for, which is created by deliberately uneven tuning of the twelve fifths of the circle of fifths. Audio file / audio sample Audio file / audio sample

Fifths and thirds in the Werckmeister III tuning (for comparison: pure major third = 386 cents , Pythagorean third = 408 cents, pure fifth = 702 cents).

Triad ceg des-f-as
c sharp-f-g sharp 		d-f sharp-a es-gb e-gis-h fac fis-ais-cis
ges-b-des 		ghd as-c-es
g sharp-c-dis 		a-cis-e bdf h-dis-f sharp ceg
Major third (ce etc.) in cents 390 408 396 402 402 390 408 396 408 402 396 402 390
Fifth (cg, etc.) in cents 696 702 696 702 702 702 702 696 702 702 702 696 696

In the Werckmeister III tuning, all fifths of the circle of fifths are pure except for the 4 fifths cg, gd, da and h-f sharp, which are reduced by ¼ point. The maximum deviation from the perfect fifth is 6 cents, while a wolf fifth (deviation 37 cents) protrudes in the mid-tone tuning .

The thirds in the chords can be divided into three classes:

Thirds quality
ce, fa, d-fis, gh, bd almost pure (pure: 386 cents)
es-g, e-gis, a-cis, b-dis comparable to the thirds of the equal tuning (400 cents)
des-f, ges-b, as-c Pythagorean third (Pythagorean: 408 cents)
026bachstimmung.gif Listen:
Cadence in F major, E flat major and G major Werckmeister III ? / i equal ? / i
Audio file / audio sample
Audio file / audio sample
Chords in the same pitch as possible

Audio file / audio sample Listen ? / i

The four fifths c – g – d – a and h – f sharp are narrowed by ¼ of a comma, all others are pure.

Here you can hear the restless character of the first chords, which gradually change into a clear B flat major, F major, C major, G major and D major chord, only to get rougher again. In C major you can hear the typical beat of the mean-tone fifth.

Relationship between well-tempered and equal

Our pianos today are usually tempered at the same level (historical terms for this: evenly, equally suspended). The key characteristic is lost here, since every small second is tuned as evenly as possible as louder equal semitone steps. Werckmeister was familiar with this uniform twelve-step temperature from Gioseffo Zarlino , whose geometric monochord construction from 1588 he cited. Zarlino described it as a lute tuning that was well known long before him in the early 16th century. In Werckmeister's thoroughbass school of 1698, it appears expressly as a borderline case. In his work Musicalische Paradoxal-Discourse , published posthumously in 1707 , Werckmeister even emphatically recommends the evenly floating mood, since it "can be an example of how all pious and well-tempered people will live and jubilate with God in constant, equal and eternal harmony." (P. 110) Other temperature theorists such as Georg Andreas Sorge (1744) later took on the equation. Equal tuning was rejected by various theorists, however, because of the leveling of the key characteristics and because of vocal technical problems that had not yet been satisfactorily solved, for example by Johann Philipp Kirnberger , who from 1766 designed his own, uneven, well-tempered tunings that could be applied to keyboard instruments much more quickly.

The widespread assertion (e.g. in Brockhaus ) that the term well-tempered tuning is identical with equal tuning is incorrect. It is equally inaccurate to exclude the equal temperament from the canon of well-tempered moods, since Werckmeister expressly recommends it in his last work at least for “well-tempered people”. The well-known work The Well-Tempered Clavier by Johann Sebastian Bach (1685–1750) did not serve to demonstrate equal tuning, but rather a system that aimed to compose in all keys of the circle of fifths. Since Bach uses the term “well-tempered”, the reference to Werckmeister is established. It is clear that Bach did not mean an equal tuning; How exactly his mood looked, however, remains controversial.

Hypotheses on the well-tempered mood in JS Bach

With the development of well-tempered tunings, Bach was able to compose for stringed keyboard instruments in all keys of the entire circle of fifths, which was previously impossible with the mid-tone tunings. Bach's Leipzig church music, on the other hand, seems to have continued to be accompanied by organs tuned to the middle note. Johann Nikolaus Forkel reports that Bach tuned his clavichord in less than 15 minutes. How Bach voted exactly can not be deduced from the controversial dispute between Kirnberger and Friedrich Wilhelm Marpurg (1718–1795).

Interpretation of the garland on the title page: The more loops, the closer the fifths in the circle of fifths

The interpretation of the garland on the title page of Bach's Well-Tempered Clavier , Part I, 1722 as a regulation for tuning the circle of fifths by Andreas Sparschuh is controversial - for some it is impressively plausible . For this purpose, the loops in the circles should give hints to make the fifths tighter, as is typical for well-tempered moods. For Bradley Lehman this is the Rosette stone for Bach's mood problem. However, there are many controversial interpretations on this subject.

literature

Historical treatises

  • Johann Philipp Kirnberger : The art of the pure sentence in music . Koenigsberg 1774
  • Johann Georg Neidhardt : Sectio Canonis harmonici. Koenigsberg 1724.
  • Andreas Werckmeister : Organ rehearsal or a brief description ... how to temper and tune a piano with the help of the monochord ... Frankfurt / Leipzig 1681
    • Ders .: Musical temperature . Quedlinburg 1691.
    • Ibid .: The most necessary comments and rules as the Bassus continuus or basso wol could be tractieret . Aschersleben 1698.
    • Ders .: Musical Paradoxal Discourse. Calvisius, Quedlinburg 1707. (digitized version)
  • Giuseppe Zarlino: Sopplimenti musicali . Venice 1588.
  • Johann Philipp Kirnberger: Construction of the equilibrium temperature. Berlin 1760.
  • Johann Georg Neidhardt: Best and lightest temperature of the Monochordi, by means of which the today's Genius Diatonico-Chromaticum is established / that all intervals, according to the proper proportion, overtake a single beat / and therefore the modes regularis in all and every claves, in a pleasant equality / let transpose. Jena 1706.

Newer specialist literature

  • Alfred Dürr : Johann Sebastian Bach - The Well-Tempered Clavier. Bärenreiter, Kassel 1998, ISBN 3-7618-1229-9 .
  • Herbert Kelletat : On the musical temperature. Part I: Johann Sebastian Bach and his time ( ISBN 3-87537-156-9 ); Part II: Wiener Klassik ( ISBN 3-87537-187-9 ); Part III: Franz Schubert ( ISBN 3-87537-239-5 ). Edition Merseburger, Kassel 1981–1994.
  • Mark Lindley : Mood and Temperature. In: Frieder Zaminer (ed.): History of music theory. Volume 6: Hearing, Measuring and Arithmetic in the Early Modern Era. Wissenschaftliche Buchgesellschaft, Darmstadt 1987, ISBN 3-534-01206-2 , pp. 109-332.
  • Mark Lindley, Ibo Ortgies: Bach-Style Keyboard Tuning. In: Early Music. Vol. 34, No. 4, November 2006, ISSN  0306-1078 , pp. 613-623.
  • Wilfried Neumaier: What is a sound system? A historical-systematic theory of the occidental sound systems, based on the ancient theorists Aristoxenus, Eucleides and Ptolemaios, represented by means of modern algebra. Lang, Frankfurt am Main a. a. 1986, ISBN 3-8204-9492-8 ( Sources and studies on music history from antiquity to the present 9), (At the same time: Tübingen, Univ., Diss., 1985).
  • Ibo Ortgies : temperature. In: Siegbert Rampe : Bach's piano and organ works. The manual. Part 2 = Volume 4, 2nd Laaber-Verlag, Laaber 2008, ISBN 978-3-89007-459-7 , pp. 623-640 ( Bach Handbook. Vol. 4, 2).
  • Jürgen Grönewald: Did Johann Sebastian Bach vote level? In: Ars Organi . 57, 2009, H. 1, pp. 38-41.

Web links

Individual evidence

  1. ^ Johann Caspar Trost: Detailed description of the new organ work on the Augustus Castle in Weissenfels . Nuremberg 1677, p. 37 ( online ); Facsimile in: Acta Organologica . 27, 2001, pp. 36-108.
  2. ↑ Contract text printed in: Klaus Beckmann: Die Norddeutsche Schule. Organ music in Protestant Northern Germany between 1517 and 1755. Part II: Heyday and decline 1620–1755. Schott, Mainz 2009, pp. 104-105.
  3. ↑ Klaus Beckmann : The North German School. Organ music in Protestant Northern Germany between 1517 and 1755. Part II: Heyday and decline 1620–1755. Schott, Mainz 2009, pp. 114–115.
  4. ↑ Roland Eberlein : Tunder, Buxtehude, Bruhns, Lübeck: For which instruments did you write and how were they tuned? (PDF) walcker-stiftung.de, pp. 5–7; Retrieved March 19, 2016.
  5. ^ Ibo Ortgies: The practice of organ tuning in northern Germany in the 17th and 18th centuries and its relationship to contemporary music practice . Göteborgs universitet, Göteborg 2007 ( gbv.de [PDF; 5.4 MB ] First edition: 2004).
  6. ↑ The equal tuning could only be realized precisely with physical methods since 1917.
  7. ^ Herbert Kelletat : On the musical temperature: I. Johann Sebastian Bach and his time . 2nd Edition. Merseburger, Berlin / Kassel 1981, ISBN 3-87537-156-9 , p. 24.
  8. ↑ "He also tuned the piano as well as his clavichord himself, and was so skilled in this work that it never cost him more than a quarter of an hour." In: Johann Nicolaus Forkel: About Johann Sebastian Bach's life, art and works of art . Leipzig 1802. Reprint Kassel / Basel 1974, p. 17 ( online ).
  9. ^ Lecture at the 1999 annual conference of the German Mathematicians Association in Mainz. Source: Andreas Sparschuh: Voice arithmetic of the well-tempered piano by JS Bach. In: German Mathematicians Association Annual Conference 1999, pp. 154–155.
  10. ↑ Weblinks on this controversial topic: