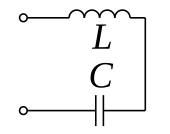
Resonant circuit
An electrical resonant circuit , as the resonant circuit is designated, a resonance capable electric circuit of a coil (L component) and a capacitor (component C), the electric vibrations can perform. The electrical oscillating circuit is often compared to the harmonic oscillator of mechanics such as the spring pendulum or the tuning fork . In this LC resonant circuit , energy is periodically exchanged between the magnetic field of the coil and the electric field of the capacitor, which means that high currents and high voltages are alternating . The resonance frequency is calculated as follows:
where stand for the inductance of the coil and for the capacitance of the capacitor. This equation is called Thomson's oscillation equation .
If a resonant circuit is triggered once by a switching process or an impulse , then it carries out free oscillations (natural oscillations), which in reality subside after a certain time due to losses. However, if it is periodically excited in the range of its resonance frequency, then it carries out forced vibrations . The resulting resonance phenomena are of paramount importance for practical use.
In the case of an oscillating circuit with external excitation, a distinction is made between parallel oscillating circuit (L parallel to C) and series oscillating circuit (L in series with C) , depending on the arrangement in relation to the excitation source . The series resonant circuit is sometimes also referred to imprecisely as a series resonant circuit .
Similar circuits consisting of coil and capacitor are also referred to as LC elements , but they are not necessarily in resonance (see low pass , high pass ).
Creation of free oscillations in the ideal oscillating circuit
A periodic process results for an externally closed circuit made up of ideal (lossless) components that contain a certain amount of energy. For the purpose of description, the state at an arbitrarily selected point in time is set as the initial state.
- First, let the coil be without magnetic flux. The capacitor is charged and the entire energy of the oscillating circuit is stored in its electric field. No current is flowing through the coil yet. (Image 1)
- Due to the voltage across the capacitor, which also drops across the coil, the current begins to flow, but it does not increase suddenly. According to Lenz's rule , a change in the current flow induces a voltage that counteracts its change. This means that the current strength and the magnetic flux only increase slowly (initially linearly with time). As the current increases, the charge in the capacitor is reduced over time, which at the same time reduces its voltage. As the voltage decreases, the increase in current flow decreases.
- When the voltage has dropped to zero, the current no longer increases and thus reaches its maximum. At this point in time, the magnetic field strength of the coil is greatest and the capacitor is completely discharged. All of the energy is now stored in the coil's magnetic field. (Picture 2)
- When the coil is de-energized, the current continues to flow steadily because - just like the magnetic flux - it cannot change abruptly. The current begins to charge the capacitor in the opposite direction. A counter-tension builds up in it (initially linear with time). This voltage, which increases with a negative sign, is equal to a voltage in the coil which, according to the rules of induction, reduces the magnetic flux over time, which at the same time reduces the current strength. As the current flow decreases, the charging of the capacitor and the increase in its negative voltage slows down.
- When the current has decreased to zero, the amount of voltage no longer increases and thus reaches its maximum. The capacitor regains its original charge, but with the opposite polarity. The entire magnetic field energy has been converted back into electrical field energy. (Picture 3)
- These processes continue in the opposite direction. (Picture 4, then again picture 1)
With continuous repetition, the voltage curve is set according to the cosine function ; the current curve follows the sine function. The transition from Figure 1 to Figure 2 corresponds in the functions to the range x = 0… π / 2; the transition from image 2 to image 3 runs as in the area x = π / 2… π, from image 3 via image 4 to image 1 as in x = π… 2π.
Free oscillations in the real series oscillating circuit
As a first approximation, the losses occurring in the real resonant circuit can be represented by an ohmic resistance R that is in series with the inductance L. Starting from the stitches set and the behavior of the three components (and assuming that the current and voltage arrows are all the same direction of rotation), such a RLC series resonant circuit by the following (linear) differential equation system (in shape state with the capacitor voltage u C i and the coil current as State variables):
If you are only interested in the current in the resonant circuit, then you can (by eliminating u C ) convert this DGL system into a single linear differential equation of the second order:
If one uses the "abbreviations" for the (ideal) resonance angular frequency for simplification and generalization
and the decay constant
introduces the differential equation
The differential equation for capacitor voltage has the same form. For the two initial conditions required for a clear solution, it is usually assumed that at time t = 0 the capacitor is charged with a voltage U C0 and the current through the inductance is 0.
Real oscillating circuit
In general, a real resonant circuit can be described with the model of the damped, harmonic oscillator . If one assumes that the losses in the resonant circuit are low, specifically that is, and the natural angular frequency still leads
one, then one obtains the solution functions for the two state variables with the classical methods for the solution of a linear homogeneous differential equation , with the help of the Laplace transformation or with the help of another operator calculation
with . The minus sign in front of the current is caused by the direction of the current during discharge. The correctness of the solutions can be checked by inserting them into the differential equations and checking the initial state.
In this "normal case of practice", the current and capacitor voltage are slightly damped by the factor and are not exactly 90 ° shifted in phase from one another. The natural angular frequency ω e lies below the ideal resonant angular frequency ω 0 due to the damping . As the losses increase, it gets smaller and smaller.
Ideal oscillating circuit
For the ideal case of an oscillating circuit without losses, one obtains the solution of the undamped harmonic (90 ° phase shifted) oscillations described above.
Aperiodic borderline case
If the losses are greater, then in the special case "without overshoot" the idle state is reached again the fastest. This behavior is called the aperiodic limit case . Then you get
Creep fall
If finally applies, then there is no longer any vibration either. The greater the damping, the slower the current and voltage creep towards 0. This behavior is called the (aperiodic) creep case . If you use the "creep constant"
one, then applies to the current
Forced oscillations in the parallel oscillating circuit
For the following description of the forced oscillations , a sinusoidal alternating voltage is assumed as the excitation of the oscillating circuits , which is already present until the natural oscillations have subsided due to the loss damping due to the switch- on process . One speaks of the stationary process and can use vector diagrams and / or the complex alternating current calculation for analysis .
Ideal parallel resonant circuit
A coil and a capacitor are connected to the same voltage in parallel. With this ideal resonant circuit made up of lossless components, the resistance that can be observed at the terminals is infinitely great when the parallel resonance occurs.

With a capacitance C of the approaches phase angle φ of the current vs. the applied voltage at 90 ° advance , d. H. the voltage is 90 ° behind the current in the phase; see phasor diagram .
- Key point: The condensate or the current lags v or .
When an inductance L , the current phase runs over the voltage phase by 90 ° after .
- Key point: In the inductance ät comes the power to sp ät .
If the arrow for I C is longer than the arrow for I L , then the capacitive resistance in the parallel connection is smaller than the inductive resistance; In the case under consideration, the frequency is higher than the resonance frequency. (At resonance, the arrows are for I C and I L equal length.) The resulting current I tot in the supply lines to the oscillation circuit is by graphic addition of I L and I C added.
The amounts of the total current is always smaller than the larger single stream through C or L . The closer you get to the resonance frequency, the more I tot approaches zero. In other words: close to the resonance frequency, the current flowing within the resonant circuit is significantly greater than the current in the supply lines ( excessive current ).
The sum current arrow points upwards in the present drawing. This means that the resonant circuit behaves like a capacitor with low capacitance at the present frequency; the frequency is above the resonance frequency. Precisely at the resonance frequency, I tot = 0, and the parallel resonant circuit does not let any current through. Below the resonance frequency, I tot points down, and the resonant circuit acts like an inductance.
The currents are limited by the capacitive and inductive alternating current or reactance . For a coil with inductance L, the following applies for the frequency or the circular frequency :
correspondingly for a capacitor with the capacitance C :
The negative sign stands for the opposite direction of the current arrow. (For the sign convention used, see note under reactance , for derivation see under Complex AC Calculation ).
To calculate the resonance frequency of the ideal oscillating circuit, it is assumed that the impedance at the terminals is infinitely large, i.e. the conductance of the parallel circuit is zero.
or
Real parallel resonant circuit
A real resonant circuit always contains losses in the coil and the capacitor; the ohmic resistance of the lines and the coil winding, dielectric losses in the capacitor and radiated electromagnetic waves. A residual current then remains at the terminals, which is in phase with and which does not go to zero even in the case of resonance. Therefore the resonance resistance does not become infinitely large in a real parallel resonant circuit. The impedance only reaches a maximum.
The losses of the capacitor can usually be neglected compared to the coil losses. For the lossy coil, its series equivalent circuit with and is preferably used . After transformation into its parallel equivalent circuit with and one obtains the circuit on the right in the picture. The conductance of the parallel connection is off and is zero in the case of resonance. In this case, the impedance in the parallel resonant circuit is limited to the (by definition, purely ohmic) resonance resistance ; this results in:
The above-mentioned resonance frequency of the ideal resonant circuit applies to . The real resonant circuit dealt with here results from the parallel equivalent circuit diagram
It is typically (see following example) somewhat smaller than and can be converted to
This resonance frequency for forced vibrations has a different value than the natural frequency given above for free vibrations.
The locus curve shown illustrates the properties of a parallel resonant circuit using a specific example:
- In the case of resonance, the oscillating circuit has a finitely high, purely ohmic resistance ; The length of the pointer in a horizontal position is clear ; in the example is twenty times the DC resistance .
- The resonance resistance is not at the same time the maximum of the impedance ; clearly occurs at the maximum distance of the locus from the zero point somewhat below the real axis; in the example is about 2.5% smaller than .
- The actual resonance frequency is lower than the frequency calculated using Thomson's oscillation equation ; this can be seen in the frequency values along the locus; in the example is about 2.5% smaller than .
-
occurs at a frequency close to. When the active component of the impedance is exactly the same . But there is also a clear capacitive reactive component; clearly has a blind component due to the vertical component of the pointer; in the example the value of the reactance is greater than 22% of .
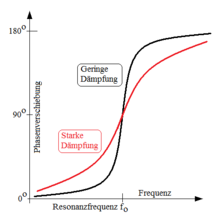
Phase shift
If an oscillating circuit is excited to forced oscillations by an external oscillator and weak inductive coupling (see measuring circuit), it reacts with a phase shift between 0 ° at extremely low frequencies and 180 ° at very high frequencies. At the resonance frequency f 0 , the phase shift is exactly 90 °.
In the vicinity of the resonance frequency, the deviation of the phase shift φ from 90 ° is almost proportional to the deviation of the frequency f . This is used in demodulation circuits of frequency modulation .
The proportionality factor k is greater, the smaller the damping of the resonant circuit. This can be changed through the series resistance to the inductance. With vanishing damping, the curve would have the form of a Heaviside function .
Forced oscillations in the series oscillating circuit
Ideal series resonance circuit
In the case of the LC series resonant circuit, the coil and capacitor are connected in series. The same alternating current flows through both, causing an oscillation that is forced at its frequency . In the case of sinusoidal excitation, a voltage that leads the current by 90 ° forms on the coil, and a voltage that leads by 90 ° on the capacitor. The voltages are directed against each other, so that their sum is always smaller in magnitude than the respective larger individual voltage. In special cases, they cancel each other out, which corresponds to a short circuit. This case is called series resonance or series resonance of an LC series resonant circuit. It is reached at the resonance frequency of the oscillating circuit. The (reactive) resistance of the series connection is
At the resonance frequency , the capacitive and inductive reactance cancel each other out, which causes the short circuit; . (The same applies to the sign convention for as above for the parallel resonant circuit.) So for resonance, the following applies
If the frequency is above the resonance frequency, the amount of the inductive reactance (coil) is greater than the capacitive one, so that the reactive component of the complex total resistance is positive. As the frequency increases, the capacitor supplies an ever smaller proportion of the total reactance, the coil an ever increasing proportion. If the frequency is below the resonance frequency, the capacitive reactance of the capacitor is larger in magnitude than the inductive reactance of the coil, and the reactive component of the total resistance has a negative sign. Here, the coil resistance becomes increasingly smaller with decreasing frequency and the increasing amount of the reactance of the capacitor is compensated less and less.
In a series resonant circuit, there is an excessive voltage increase, because individually higher voltages occur across L and C than on the connection terminals (see resonance transformer ).
Real series resonant circuit
In the real case there is an ohmic resistor in series in addition to the capacitor and coil. This can be another component or just the wire of the coil.
The locus curve shown illustrates the properties of a series resonant circuit using a specific example:
- In the case of resonance, the oscillating circuit has a small, purely ohmic resistance Z 0 . This is as great as the resistance R alone.
- The resonance resistance is also the minimum possible impedance across all frequencies.
- The resonance frequency is the same as for the ideal resonant circuit.
Circular quality
In real resonant circuits, losses also occur in the coils and capacitors (ohmic losses, dielectric losses, radiation). These lead to the oscillation of a resonant circuit being dampened. On the other hand, without any damping, the amplitude would increase beyond all limits in the case of resonance. The quality factor is a measure of the losses.
The resonance curve shows in a diagram how far there is an amplitude increase depending on the excitation frequency for a given quality factor.
oscillator
Once triggered and then left to its own devices, an oscillating circuit oscillates in the vicinity of its resonance frequency f 0 . As a result of the damping through losses, the amplitude of the oscillation decreases over time (“damped oscillation”) unless energy is regularly supplied again by an active amplifier circuit (for example with a transistor ) or a negative differential resistor . One then speaks of positive feedback or undamping of the resonant circuit. Such a circuit forms an oscillator (vibration generator), an example is the Meißner circuit .
poll
The resonant frequency depends on L and C and can therefore by changing L or C are affected. The resonant circuit is thereby tuned to a specific frequency .
The inductance L can be increased by inserting a ferromagnetic core ( iron or ferrite ) more or less far into the coil . The field can also be displaced by inserting a highly conductive core - then the inductance is reduced.
The capacitance C can be changed by changing the plate size or the plate spacing of the capacitor . With the rotary condenser and with many trimmers this is done by twisting the plates laterally against each other, so that the proportion of the opposing surfaces is changed. Other circuits use a capacitance diode instead, for example .
application
filter
The impedance is frequency-dependent; in the vicinity of the resonance frequency it is minimal in the series resonant circuit and maximum in the parallel resonant circuit. This frequency dependency makes it possible to filter out a certain frequency from a mixture of signals of different frequencies - either to let it through alone or to suppress it in a targeted manner. The parallel resonant circuit also has the advantage of allowing direct current such as the operating current of the transistor to pass unhindered. This is why a parallel resonant circuit is always used when used in a selective amplifier .
- In older telephone systems, both voice and - at a higher frequency - the charge pulses were sent over the two-wire line. A blocking circuit (parallel resonant circuit as two-pole) was built into the telephone set in order to suppress the frequency of the impulse for the listener. Only this was sent via a series oscillating circuit to the charge meter, before which the voice frequencies were blocked.
- With parallel resonant circuits, radio receivers are tuned to the desired transmitter . An oscillating circuit is connected between the input poles - in the simplest case of the detector receiver, directly between the antenna and earth. The output signal is picked up at these connections and sent for further processing (mixing in a heterodyne receiver, demodulation).
- The output stages of transmission systems often generate unwanted harmonics that must not be emitted via the antenna and must be suppressed by some resonant circuits after the output stage. If the resonant circuit is replaced by a resonance transformer , the line can also be matched to the impedance of the antenna cable.
- With suction circuits , interfering frequencies can be filtered out (short-circuited) from a composite signal. To do this, it is connected in front of the actual receiver between antenna and earth. In the case of simple radio receivers, a very strong local transmitter can be filtered out in order to then tune the actual frequency selection stages to the desired frequency of a more distant and therefore weaker transmitter that would otherwise be superimposed by the local transmitter. A blocking circuit in the antenna feed line is also well suited and often used.
Parallel and series resonant circuits can also take on the other task depending on the wiring. A loosely coupled parallel resonant circuit can only absorb energy at its natural frequency ( suction circuit ); a series resonant circuit in a signal line allows only frequencies of its natural resonance to pass. On the other hand, a parallel resonant circuit connected in series in a signal line does not allow its natural frequency to pass - provided that it is not significantly attenuated by it.
Compensation of reactive current
Consumers in the electrical power supply network draw electrical energy and give it to z. B. as thermal, mechanical, chemical energy. In many cases, they also store energy, e.g. B. in motors as magnetic field energy. The field is built up and reduced again in the rhythm of the alternating mains voltage, and the energy is drawn in and returned. This energy pendulum generates reactive current , which loads the source and the grid and should be avoided. An oscillating circuit is set up for this purpose: a capacitance is connected in parallel to an inductance - or vice versa. The additional component is dimensioned in such a way that the resonance frequency is the same as the mains frequency, which results in the highest possible impedance. This circuit measure is called reactive current compensation .
Resonant circuits as equivalent circuit diagrams
In addition to resonant circuits, there are many other electronic constructions that are used in applications instead of resonant circuits (especially at very high frequencies). See Lecher line , pot circle , cavity resonator , but also antenna dipole . The physical function of these constructions is mostly based on the use of standing waves and thus differs fundamentally from the physical function of an oscillating circuit. For such constructions, equivalent circuit diagrams are often given in the form of electrical oscillating circuits, which allow a simplified, approximate calculation of their behavior.
Equivalent circuit diagrams with their ideal electronic components reproduce the behavior of the "replaced" construction, but not their physical mode of operation.
Measuring device
The resonance frequency of oscillating circuits in the MHz range can be measured with a dipmeter .
literature
- Wilfried Weißgerber: Electrical engineering for engineers 2 . Vieweg / Teubner, Wiesbaden 2009, ISBN 978-3-8348-0474-7 .
- Martin Gerhard Wegener: Modern radio reception technology . Franzis-Verlag, Munich 1985, ISBN 3-7723-7911-7 .
- Wolfgang Demtröder: Experimental Physics 2 . Springer-Verlag, Berlin 2006, ISBN 3-540-33794-6 .
- Klaus Lunze : Theory of AC circuits . Verlag Technik, Berlin 1991, ISBN 3-341-00984-1 .
- Ralf Kories and Heinz Schmidt-Walter: Taschenbuch der Elektrotechnik . Harri Deutsch publishing house, Frankfurt a. M. and Thun.
Web links
- HTML5 app for demonstrating an oscillating circuit