Covalent bond

Covalent bond (older terms: atom bond , electron pair bond or homeopolar bond ) is a form of chemical bond and as such is responsible for the firm hold of atoms in molecularly structured chemical compounds . Covalent bonds are especially formed between the atoms of non-metals . In ion crystals , on the other hand, mainly ionic bonds act, and in metals metallic bonds .

In the case of covalent bonds, the interaction of the external electrons ( valence electrons ) with the atomic nuclei of the atoms involved plays a key role. The atoms form at least one electron pair between them. This pair of electrons holds two ( two-center bond ) or more ( multi-center bond ) atoms together, so it is binding and is therefore called a bonding electron pair . In addition to a bonding pair of electrons (single bond, a σ bond ), two ( double bond , σ bond plus a π bond ), three ( triple bond , plus another π bond) and even more electron pairs ( δ bond for bonds between Elements of the subgroups ). A covalent bond has a specific direction of action, i.e. it is a directional bond and thus determines the geometric structure of a connection. The strength of a bond is described by the bond energy . During the chemical reaction of corresponding substances with one another, one or more covalent bonds are made or broken.

Basics

It is known from experience that molecules do not form in any combination of atom types and numbers during chemical reactions. The electron shells of atoms of the same or different elements must be suitable for forming bonds with one another. However, a more precise description of the electron shells is only possible with more complex mathematical methods (see molecular orbital theory , valence structure theory ). The less complicated noble gas rule is an important and powerful tool in understanding bonding relationships . It allows the graphical representation of many chemical compounds as valence line formulas , in which bonding electron pairs are shown by lines between the element symbols.

Noble gas rule

According to Lewis and Kossel (1916), chemical compounds are particularly stable when the atoms involved reach the closest noble gas configuration in the periodic table ( noble gas rule ). The closest noble gas to hydrogen is helium with only two electrons. For hydrogen, the rule is therefore fulfilled with only two electrons and is accordingly present as a molecule that is held together by a covalent bond (H − H).

In many cases, atoms in compounds reach a valence shell with four electron pairs, i.e. they have an electron octet and fulfill the so-called octet rule . The octet rule applies to most connections of main group elements . This rule of thumb works well for the elements of the second period , such as carbon , nitrogen and oxygen , which are the important elements of innumerable organic compounds .

Limits of the noble gas rule

Physics of the covalent bond

For a bond of 2 atoms (or 2 bodies in general) at a fixed distance, there is a balance between an attractive and a repulsive force. If we consider the simple case of the hydrogen molecule, the following results: The repulsive force is the electromagnetic force between the positive nuclei, which becomes stronger the closer the nuclei move together. The attractive force is caused by the exchange interaction of the 2 electrons: the wave function of the 2 electrons in the symmetrical case results in a probability of the electrons being between the nuclei. The electrons shield the nuclear charges from one another and are themselves largely enclosed between the nuclei. A binding electron pair is created. A detailed description can be found in the article Molecular Orbital Theory, section Hydrogen .

Bonding electron pairs

Examples of electron formulas
Single bond
${\ displaystyle \ mathrm {\ vert {\ overline {\ underline {Cl}}} \ cdot \ + \ \ cdot {\ overline {\ underline {Cl}}} \ vert \ longrightarrow \ vert {\ overline {\ underline { Cl}}} {-} {\ overline {\ underline {Cl}}} \ vert}}$ Chlorine (Cl ₂ )
${\ displaystyle \ mathrm {4 \ H \ cdot \ + \ _ {\ cdot} ^ {\ cdot} C _ {\ cdot} ^ {\ cdot} \ longrightarrow}}$ ${\ displaystyle \ mathrm {H {-} {\ overset {\ displaystyle H \ atop \|} {\ underset {\| \ atop \ displaystyle H} {C}}} {-} H}}$ Methane (CH ₄ )
Double bond
${\ displaystyle \ mathrm {^ {\ cdot} _ {\ cdot} C _ {\ cdot} ^ {\ cdot} \ +2 \ _ {\ cdot} ^ {\ cdot} \ O _ {\ diagup} ^ {\ diagdown } \ \ longrightarrow \ \ _ {\ diagdown} ^ {\ diagup} O {=} C {=} O _ {\ diagup} ^ {\ diagdown}}}$ Carbon dioxide (CO ₂ )
Triple bond
${\ displaystyle \ mathrm {{\ overline {_ {\ cdot} N _ {\ cdot} ^ {\ cdot}}} \ \ + \ {\ overline {_ {\ cdot} ^ {\ cdot} N ^ {\ cdot }}} \ \ longrightarrow \ vert N {\ equiv} N \ vert}}$ Nitrogen (N ₂ )
Formal charge
${\ displaystyle \ mathrm {^ {\ cdot} _ {\ cdot} C _ {\ cdot} ^ {\ cdot} \ \ + \ _ {\ cdot} ^ {\ cdot} O _ {\ diagup} ^ {\ diagdown} \ \ longrightarrow \ ^ {\ ominus} \ vert C {\ equiv} O \ vert ^ {\ oplus}}}$ Carbon monoxide (CO)

Molecular substances exist through covalent bonds , such as oxygen (O ₂ ) or carbon dioxide (CO ₂ ), but also substances such as diamond (C _diamond ) or silicon dioxide (SiO ₂ ), which do not form molecules but atomic lattices . Complex ions , i.e. molecules that carry electrical charges, are held together by covalent bonds. Although these ions form salts through ionic bonds , the atoms of the complex ions such as ammonium (NH ₄⁺ ) or sulfate (SO ₄²⁻ ) are held together by covalent bonds.

The valence electrons of non-metal atoms can be represented graphically in an electron formula, with electrons being distributed in four positions around the element symbol. Dots represent individual electrons, while lines symbolize lone electron pairs (also: free electron pair , non-binding electron pair ). The electron formulas of the atoms can be combined to form molecules of many known chemical compounds and, if the atomic composition of small molecules is known, the molecular structure of a compound can be predicted. In order to arrive at a valence line formula for a molecule, solitary electrons (dots) are combined to bond electron pairs (bars) or electron pairs (bars) are shifted between atoms in such a way that the octet rule is fulfilled. Double bonds and triple bonds between two atoms are also possible here (see also: Lewis notation ).

The formal assignment of binding and non-binding electron pairs to represent a chemical compound occasionally leads to a so-called formal charge . It is the difference between the positive nuclear charge and the negative electrons assigned to the atom and is often given as a superscript plus or minus sign in a circle symbol. Formal charges exist , for example, in the case of carbon monoxide .

The coordination number of an atom indicates the number of the closest neighboring atoms and is, for example, with respect to the C atom in carbon monoxide 1, in carbon dioxide 2 and in methane 4.

Polarity of covalent bonds

→ Main article: Polar atomic bond

The electron- attracting forces ( electronegativity , En) are a measure of the ability of an atom in a chemical bond to attract the binding electrons. The electronegativity of binding partners is only exactly the same for element molecules and only here are ideal covalent bonds. Strictly speaking, only these bonds can be called non-polar or also homeopolar .

If the binding partners differ in their electronegativity, there are polar or heteropolar covalent bonds. The binding electrons are more or less unevenly distributed between the binding partners. Your focus has shifted towards the more electronegative partner. The atom with the greater electronegativity draws the bonding electrons closer to itself. This gives this binding partner a negative partial charge, which is symbolized by δ ^- . The electron shell of the atom at the other end of the bond is depleted accordingly of negative charge density and the atom receives a positive partial charge (δ ⁺ ). Such covalent bonds are called polar bonds , since poles with different partial charges arise.

In the case of very polar covalent bonds, binding electrons can largely be assigned to a binding partner. It is the borderline case of ionic bonds and in some cases it makes sense to describe the compound as ionic.

Hydrogen fluoride (HF)	Carbon dioxide (CO ₂ )	Water (H ₂ O)

${\ displaystyle \ Delta E_ {N} = 1 {,} 9}$	${\ displaystyle \ Delta E_ {N} = 1 {,} 0}$	${\ displaystyle \ Delta E_ {N} = 1 {,} 4}$

Dipole moment

Polar bonds can lead to the entire molecule being polar: the molecule then carries a dipole moment and is present as a dipole molecule . Whether a molecule has a (measurable) dipole moment depends not only on the polarity of the bonds, but also on the structure of the molecule. The dipole moments of different bonds in the molecule add up depending on the direction ( vectorial ) and can therefore cancel each other out. As a diatomic, heteronuclear compound, hydrogen fluoride has a dipole moment. Carbon dioxide has a total dipole moment of zero because the bond dipoles are oppositely oriented and cancel each other out. Water has a larger total dipole moment than hydrogen fluoride, although the polarity of the O – H bond is smaller than that of the H – F bond. The cause lies in the addition of the two OH bond dipoles, which are at a bond angle of about 105 ° (see below) to one another.

Bonding electron pairs from lone electron pairs

Lone pairs of electrons in a compound can take on the role of bonding pairs of electrons in a reaction. This type of bond is called a coordinative bond (also: dative covalent bond ) and occurs in compounds such as the ammonium cation and in complex compounds . Coordinative bonds are similar to the weak bonds that occur, for example, in hydrogen bonds .

Examples

Coordinative bond in the ammonium cation	Coordinative bond in the diammine-silver-I complex	Hydrogen bonds between two water molecules

geometry

Spatial orientation

→ Main article VSEPR model

Three interconnected atoms in an atomic lattice, molecule or complex are at a certain bond angle to each other. Knowing about bond angles allows the structural formula of a compound to be established. From knowledge of bonding and non-bonding electron pairs in a compound, bond angles can be estimated with the aid of the electron pair repulsion model. The bond angles result from an arrangement of the electron clouds at the greatest possible distance from one another. An electron cloud can consist of a single electron (for radicals ), a non-bonding pair of electrons or single bonds. For a simple estimate, double and triple bonds can be thought of as a single cloud.

Examples	Hydrocyanic acid (HCN)	Carbonic acid (H ₂ CO ₃ )	Water (H ₂ O)	Ammonia (NH ₃ )	Methane (CH ₄ )
Illustration
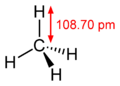
Number of spherical clouds	2	3	4th	4th	4th
appraisal	linear (180 °)	trigonal planar (120 °)	tetrahedral (109.47 °)	tetrahedral (109.47 °)	tetrahedral (109.47 °)
actual bond angle	180 °	about 120 °	104.5 °	107.8 °	109.47 °

Considerable deviations can occur between an estimate of a bond angle using the electron cloud model and real molecules. The actual bond angle in the water molecule is not 109.47 °, but 104.45 ° due to the lower repulsive effect of the non-bonding electron pairs on the bonding pairs, or the smaller size of the sq-bond orbitals that contain the proton.

Bond length

H-H	H-F	H-Cl	H-Br	HI
74 pm	92 pm	128 pm	141 pm	160 pm

C-C	${\ displaystyle \ mathrm {C {\ overline {\ ldots}} C}}$	C = C	C≡C	N-N	N = N	N≡N
154 pm	139 pm	134 pm	120 pm	146 pm	125 pm	110 pm

The atomic distances in molecules and complexes with covalent bonds can be determined experimentally by analyzing the rotational spectra. The bond lengths depend on the size of the atoms . The larger their radius, the greater their distance.

In the case of bonds between atoms of the same type, the distance between them also depends on the number of bonding electron pairs: the more bonding electron pairs that are active, the shorter the bond length. The shape of the bond potential can be described by the Morse potential .

Geometry of multiple bonds

geometry
ecliptic & staggered butane
2-butene

Single bonds determine the bond angles between atoms, but they can rotate in themselves. A molecule like n -butane can rotate easily and therefore exists in different conformations . All conformations describe the same connection. Multiple bonds, on the other hand, cannot be twisted. The double bond is particularly important in organic compounds . Hydrocarbons such as 2-butene exist as two different chemical compounds, namely cis - and trans -2-butene. The rigidity of the double bonds generally leads to the so-called cis - trans isomerism . Further distortions of multiple bonds can be described by the Carter-Goddard-Malrieu-Trinquier model .

Conjugated double bonds and aromatic bonds

If double and single bonds alternate in a molecule, the atomic distances of the single bond are shorter (tighter) than in the case of single bonds without double bonds in the vicinity. On the other hand, this has a lengthening effect on the multiple bonds. This phenomenon is called conjugation and can hardly be explained with the simple binding models described here.

A special case is the aromaticity: Here there are only formal sequences of double and single bonds, but the atomic distances are all equally short. A simple aromatic compound is the ring-shaped molecule benzene (C ₆ H ₆ ). Valence line formulas of this compound lead to two possible representations, which are referred to in the figure as mesomeric boundary structures. Both valence formulas lead to the correct assumption that benzene is a flat (planar) molecule, since the geometry requires trigonal planar orientations. Each CC bond can be represented as a double or single bond. In reality, the double bonds are not in fixed locations, but are distributed ( delocalized ) over the entire ring . All aromatic compounds, i.e. compounds with delocalized double bonds, must meet the so-called Hückel rule , which is based on quantum mechanics .

Benzene (C ₆ H ₆ )

Benzene with six electrons (more precisely six π electrons) in delocalized double bonds , one of the simplest aromatic compounds, represented here by mesomeric boundary structures . (Note: the presentations above and below are equivalent.)	The delocalized electrons and the equality of bonds is represented by the ring in both drawings of the benzene molecule. (The presentation on the right can sometimes be found in school books.)	Historic Kekulé benzene formula from the original publication.

Structure of amides

The creation of mesomeric boundary structures with the simple bond models described here also allows estimations of rather complicated bond relationships. The figure on the right shows the peptide bond in two boundary structures. Boundary structure 1 suggests a CNC bond angle of 109 ° (tetrahedral), while boundary structure 2 indicates an angle of 120 ° (trigonal planar). In reality there is a bond angle of 122 °, as it results from boundary structure 2 with formal charges . The CN distance of the possible double bond is 133 pm between a C – N single bond (147 pm) and a C = N double bond (130 pm).

Binding energy

Enthalpy of bond dissociation
binding	Bond length in pm	Enthalpy of binding in kJ / mol
H-H	74	436
F-F	142	159
Cl-Cl	199	242
Br – Br	228	193
I-I	267	151

C-H	108	413
C-F	138	489
C-Cl	177	339
C-Br	228	285

C-C	154	348
C = C	134	614
C≡C	120	839

The bond energy (also: dissociation energy, bond cleavage energy, bond enthalpy, bond dissociation enthalpy or valence energy) is the same as the energy required to split a covalent bond and convert a compound (A − B) into two radicals :

A − B → A + B

This dissociation is called homolytic fission . The bond dissociation enthalpy can be measured for simple molecules and estimated for more complex molecules by measurements and calculations. Like the bond length (see above ), it depends on the size of the bonded atoms. The larger the radius of the binding partners, the greater their distance and the smaller their binding energy. In the case of bonds between atoms of the same type, it can also be seen that their distance becomes smaller with an increasing number of bonding electron pairs, while their binding energy increases.

Individual evidence

^ Brockhaus ABC Chemie , VEB FA Brockhaus Verlag Leipzig 1965, pp. 226–229.
↑ Otto-Albrecht Neumüller (Ed.): Römpps Chemie-Lexikon. Volume 1: A-Cl. 8th revised and expanded edition. Franckh'sche Verlagshandlung, Stuttgart 1979, ISBN 3-440-04511-0 , pp. 662-668.
↑ August Kekulé: About some condensation products of the aldehyde , Liebigs Ann. Chem. 1872 , 162 (1), pp. 77-124; doi : 10.1002 / jlac.18721620110 .

Web links

Wikibooks: Inorganic Chemistry for Students / The Atom Binding - Learning and Teaching Materials

Wiktionary: atomic bond - explanations of meanings, word origins, synonyms, translations

Wiktionary: covalent - explanations of meanings, word origins, synonyms, translations

[ABC_Chemie-1] Brockhaus ABC Chemie , VEB FA Brockhaus Verlag Leipzig 1965, pp. 226–229.

[RÖMPP-2] Otto-Albrecht Neumüller (Ed.): Römpps Chemie-Lexikon. Volume 1: A-Cl. 8th revised and expanded edition. Franckh'sche Verlagshandlung, Stuttgart 1979, ISBN 3-440-04511-0 , pp. 662-668.

[August_Kekulé-3] August Kekulé: About some condensation products of the aldehyde , Liebigs Ann. Chem. 1872 , 162 (1), pp. 77-124; doi : 10.1002 / jlac.18721620110 .