Distance measurement

Under distance measurement , distance measurement or length measurement refers to the measurement of the distance between two points in space by direct or indirect comparison with a unit of length such as the meter . Optical rangefinders are also called telemeters .

The range of possible lengths begins with the so-called Planck length of around 10 ⁻³⁵ meters. This is the smallest length into which the room can be divided. The physically relevant range begins at 10 ⁻¹⁸ meters, the size of the elementary particles , and extends to 10 ²⁶ meters. It spans 44 decimal orders of magnitude - from atomic physics to biology and technology to the most distant galaxies . This means that a very large number of different methods are necessary to measure distances.

If you combine distance and direction measurement , the position of points can be determined in a plane or spatial coordinate system - see geometry , surveying , positioning , navigation and astrometry .

The following diagram gives an overview of the unit attachments for length and distance measurements, contains examples for the size ranges and assigns the respective measurement principles.

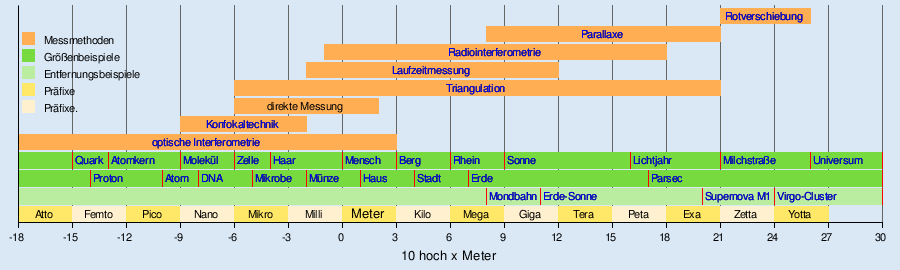
Measurement principles

Distance measurement in the military (1903)

Direct measurement

The most direct form of distance measurement is the so-called direct measurement . This means the direct comparison of the distance to be determined with a yardstick. This type of measurement is only possible in a limited range of lengths, as comparison scales cannot be produced in any size. The smallest scales are produced using lithographic methods and are only a few micrometers in size. They can be used like a normal measuring tape under a microscope or can be read off automatically with optical devices (see glass ruler ). The longest rules are made from flexible steel strip in lengths of over 100 meters.

All these scales are reduced to a normal length (earlier the original meter , today the definition of the meter using the light transit time). This process is known as calibration . The definition of the meter enables the worldwide comparability of length measurements.

From a computational point of view, the direct distance measurement is to be treated as an inclined section, which requires the measurement of its inclination or the elevation angle for its conversion into a horizontal section .

Some methods of direct distance measurement are listed and briefly explained below.

Interferometry

The interferometry with coherent waves is very precise for the measurement of changes in length. The accuracy essentially depends on the wavelength used. In practice, light and radio waves are used. In order to be able to measure distances with an interferometer , the phase shifting method , white light interferometry or conoscopic holography are used , among other things . Interferometry is a direct measurement technique, as the distance to be determined is compared with the wavelength of the light used. The wavelength is linked to the international system of units .

Confocal distance measurement

The confocal technology is used in various technical versions to determine very small distances in the nanometer to millimeter range. It is based on the effect of depth discrimination: a confocal sensor delivers a signal that is larger the closer the measurement object is to the focal plane of the optics. The confocal technique is a direct measurement because it shifts the object or optics by the measuring length and compares the shift with a reference scale.

Indirect measurement

In many cases, direct measurement cannot be used. Already when determining the distance between two islands from the mainland it fails because the two points are not accessible at the same time. Indirect methods are more universal and usually more convenient to use .

All indirect methods have in common that they do not measure the distance itself, but a quantity that depends on it - for example, measuring the transit time of a signal or echo (laser, radar ), the direction of a bearing or the brightness of a star . All changes in distance are also measured indirectly , for example using the Doppler effect (see also approximation ). Indirect measurements have to be calibrated by comparing them with known standards so that they can be compared with other measurements.

Hodometry

Measuring wheel

The Hodometrie , often after English as odometry called, is a very simple and old method of indirect displacement measurement, which can be restricted also be used for distance measurement. The revolutions of a wheel with a known circumference that is rolled on the measuring section are counted. The number of revolutions multiplied by the circumference gives the measured distance. In everyday life, this method is used, for example, for odometers in cars or measuring wheels in surveying.
( See also: Curvimeter , device for measuring curvy routes in maps)

Inertial navigation

Inertial navigation is based on the fact that a movement that is not uniform is always associated with an acceleration of the moving object. If you integrate all accelerations that an object has experienced, depending on the direction over time, you can use the simple formula speed equals acceleration times time and distance equals speed times time to calculate the distance covered (double numerical integration). This method is used in all types of vehicles and aircraft to enable distance measurement that is independent of the environment. However, the measurement errors also add up over time, which is why the position must be compared at regular intervals with information from another source.

Triangulation

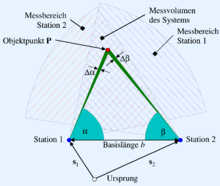
Principle of triangulation

Triangulation was already used for land surveying in ancient times and is still used today, with the exception of technical innovations. The measuring process itself uses direct or indirect angle measurements to the measuring point if the length of the measuring base is known. The distance to the base can be calculated from the spatial position of two angles.

With triple angulation or triangulation , you aim for the desired measuring point from at least two different locations with a known distance using a theodolite or another protractor. The object point P and the two positions (1 and 2) form a triangle whose base length , and the base angle , and is known. With this one can calculate all other quantities in the triangle. The base length is the measure of the triangulation. The three angles of the triangle give the method the name 'Triangulation'. ${\ displaystyle b}$ ${\ displaystyle \ alpha}$ ${\ displaystyle \ beta}$

{\ displaystyle {\ tfrac {b} {\ sin (180 ^ {\ circ} - \ alpha - \ beta)}} = {\ tfrac {\ overline {BP}} {\ sin \ alpha}} = {\ tfrac {\ overline {AP}} {\ sin \ beta}}}

Optical rangefinders that work according to this principle are the coincidence and spatial image rangefinders . In reverse of this procedure, the end points of a highly precise reference route, a so-called base staff, can also be targeted from one point. The base bar thus forms the base length of an isosceles triangle.

Optical measuring techniques such as fringe projection and photogrammetry , which have opened up other areas of application, have been added.

Also distance sensors use the principle of triangulation (see laser triangulation ).

A simple way of estimating distances without technical aids is the thumb jump . It only assumes that you know the approximate size of the target object.

Trilateration

The trilateration is a similar process of triangulation, which is also used three sizes of a triangle to the description of which, namely the sides. From this, in turn, all other quantities that define the triangle can be calculated.

The measurement process itself uses direct or indirect (radio-technical) distance measurements, in each case realized in terms of measurement technology as time-of- flight measurements (unambiguous) or additionally through phase comparisons (ambiguous). From the spatial position of two points, their distance can be calculated on the basis of geometric relationships in the triangle. The method is primarily used to determine the spatial position of measurement points to one another on the basis of simple distance measurements quickly and with sufficient accuracy.

To determine the position of an unknown point in three-dimensional space, four known points are required, three known points are sufficient on a surface and two known points are sufficient along a trajectory . If you can choose between several mathematically correct solutions on the basis of constraints, the number of necessary known points can be reduced by the number of usable constraints.

Trilateration is the basis of distance measurement, for example in satellite navigation systems or in global navigation satellite systems . In the sense of classical geodesy , trilateration is not an independent measurement method, but it is in satellite geodesy (see also Satellite Laser Ranging and SECOR ).

Runtime measurement

Principle of the transit time measurement

The transit time measurement is based on the fact that electromagnetic and acoustic waves propagate at a finite, known speed. If you send a signal to an object to be measured, from which it is reflected, and measure the time it takes to travel there and back, you can determine the distance to the object from the transit time and the speed of propagation of the signal, i.e. the group speed of the wave to calculate: ${\ displaystyle \ Delta t}$ ${\ displaystyle c}$ ${\ displaystyle r}$

{\ displaystyle r = {\ frac {c \ cdot \ Delta t} {2}}}

The measurements are influenced by the environment. If a medium is penetrated, the speed of light is reduced compared to the speed of light in a vacuum. If the material properties are temperature-dependent or anisotropic tensors, measurements of the transit time are disturbed by changes in the parameters or the orientation. For example, the speed of sound is highly dependent on temperature, electromagnetic waves are deflected by electrically conductive layers of the atmosphere .

Determining the route is particularly problematic: only direct routes provide the direct distance. All detours via secondary reflectors lead to longer transit times and thus incorrect measurement data.

Examples:

Bats and ultrasonic distance sensors or measuring devices use ultrasonic signals to determine the distance from obstacles and prey.
The echo sounder and sonar use sound signals in the water to measure depth under ships, measure distances under water ( submarines ) and locate schools of fish.
Radar systems use electromagnetic waves in the radio wavelength range to measure distances. A distinction is made between pulse devices for large and continuous wave radar devices for short distances. See e.g. B. Distance measuring equipment , satellite navigation (e.g. GPS ), time domain reflectometry
Also, light is suitable for this method, see Satellite Laser Ranging , LIDAR , laser gun , electro-optical distance measurement .
A similar principle is used in radio navigation (in particular hyperbola navigation ) when the differences in transit time of signals sent out in a time-coordinated manner from different fixed transmitters to a receiver whose position is to be determined are converted into distance differences.

Chromatic-confocal distance measurement

The chromatic confocal distance measurement uses the dispersion of white, ie, spectrally broadband light in an optical system for determining the distance between the measuring object and the sensor. The different focal lengths for different spectral components of the sensor light due to the dispersion in the focus lens are used for the measurement.

Capacitive distance measurement

The distance between two conductive parts can be determined based on the capacitance between them . To do this, the parts must be isolated from each other; To measure capacitance, they are included in an electrical oscillating circuit or a ring oscillator , the frequency of which is sensitive to the capacitance. They are used for focus position control on laser cutting machines , for position control in nanopositioning systems and for force measurement .

parallax

Various parallax methods are used to determine distances beyond the scale of the solar system . The word "parallax" is used here in the sense of "distance". A distinction is made between the following:

Trigonometric parallax

Formation of parallax when the earth revolves around the sun

Trigonometric parallax is the change in the direction of view to an object in relation to the sky background, which is caused by the annual movement of the earth around the sun. The trigonometric parallax is based on triangulation, its base length is the diameter of the earth's orbit. In addition, one also speaks of the daily parallax , which is caused by the rotation of the earth. The further away the object, the smaller the parallax. The distance can be calculated directly from it:

{\ displaystyle r = 1 / \ pi}

where the distance r is given in parsec (pc) and the parallax in arc seconds . ${\ displaystyle \ pi}$

The first parallax measurement of a star was carried out in 1838 by Friedrich Wilhelm Bessel for the star 61 Cygni . He determined a value of about 0.3 arc seconds and thus a distance of about 3.3 pc. Proxima Centauri , the closest star to Earth, has a parallax of 0.762 arc seconds, which is a distance of about 1.31 pc. Normally the trigonometric parallax can be determined up to a distance of about 100 pc. With modern methods, however, parallaxes of a few milli-arcseconds have already been measured. Between 1989 and 1993 the Hipparcos satellite measured around 100,000 stars up to a brightness of 9 mag and determined their parallaxes. He achieved an error of only 0.001 arc seconds. The smallest parallax so far (2005) could be determined for the pulsar PSR B1508 + 55 with a radio telescope: it was 0.415 milli-arcseconds (= 0.000415 arcseconds) - this corresponds to a distance of around 2,400 pc or around 7,800 light years .

Spectroscopic parallax

In the case of spectroscopic or photometric parallax, the direction of the light is not examined as is the case with trigonometric parallax, but the quality. In addition to the temperature of a star, the intensity of the light arriving on Earth naturally also depends on the distance, which makes it possible to measure the distance. Of course, the brightness of a star that can be directly observed is only the so-called apparent brightness m . A very bright star that is far away and a very close star that shines only weakly can both appear equally bright to an earthly observer. Therefore it is necessary to define the absolute brightness M : it corresponds to the apparent brightness that an object would have if it were exactly 10 pc away from the earth. The following relationship exists between apparent and absolute brightness:

{\ displaystyle mM = -5 + 5 \ cdot \ log r}

where the distance r must be given in parsecs . If the absolute brightness of an object is known, the distance can be calculated immediately from the measured apparent brightness. The absolute brightness can be determined by comparing spectra . The spectrum of an object with a known distance is used as a yardstick - i.e. H. the spectroscopic parallax builds directly on the trigonometric parallax.

Dynamic parallax

The dynamic parallax is used to determine the distance of visual binary stars . To do this, the path speed , which can be determined spectroscopically, must be known; The distance can now be calculated from the apparent distance between the two stars and the period of rotation of the stars around their center of mass . ${\ displaystyle v}$ ${\ displaystyle a}$ ${\ displaystyle T}$ ${\ displaystyle r}$

Redshift

The determination of distance with the help of the redshift of the light is used for very distant objects such as galaxies or quasars . No alternative measurement methods are available for these distances. For the redshift, known spectral lines in the spectrum of a galaxy must be identified and their exact wavelengths measured. The distance can be calculated using the Hubble constant with the following formula: ${\ displaystyle r}$ ${\ displaystyle H_ {0}}$

{\ displaystyle r = {\ frac {z \ cdot c} {H_ {0}}}}

where are the redshift and the vacuum speed of light. ${\ displaystyle z = {\ frac {\ lambda _ {\ text {observed}} - \ lambda _ {0}} {\ lambda _ {0}}}}$ ${\ displaystyle c}$

It should be noted that the value of the Hubble constant cannot yet be precisely determined and was previously subject to strong fluctuations. The last measurements give values between 68 and 74, depending on the measuring method . ${\ displaystyle {\ frac {\ mathrm {km}} {\ mathrm {s \ cdot Mpc}}}}$

Applications

Suitable measuring equipment is required for each distance range . The following is an overview of the various distance measurement applications.

Distance measurement in everyday life

International meter prototype , standard bars made of platinum-iridium. These were the length standards ( original meters ) until 1960. ( NIST )

Direct measurement is the most common method of distance measurement in everyday life. The distance to be measured is compared with a multiple of the length standard. This is usually not done with a replica of the original meter shown on the right , but with an inexpensive and handy folding rule , tape measure or ruler . With these measuring devices, longer distances can also be determined by setting the standards several times in a row. This of course also increases the measurement error. Mechanical precision measuring instruments such as calipers or micrometer screws are used for very small everyday lengths of 0.1 millimeters to 200 millimeters . In inaccessible places z. B. between machine parts, deformable wax strips, so-called plastigauges , can also be used.

Distance covered

The distance measurement on traveled distances is usually done with an odometer , which determines the distance by counting the wheel revolutions. Such odometers are common on motor vehicles and bicycles . The procedure does imply inaccuracies of up to a few percent of the distance (e.g. a worn car tire has a slightly smaller diameter than a new one); however, it is sufficient for simple calculations for navigation or fuel consumption. A so-called bucket wheel enables more precise measurements . For simple geodetic measurements, hodometry is practiced with a measuring wheel . There is a pedometer for hikers .

Recently , GPS -based navigation systems that can measure and record distances have spread in motor vehicles, hikers and cyclists . Special systems can also measure and record height profiles , which can be of interest to mountain hikers, cyclists and mountain bikers. Hang gliders , glider pilots and paragliders also use such systems.

Designations on the right triangle

The steeper the distance traveled, the greater the difference between the actual route and the route indicated by the GPS system (the term pseudo distance is used for the latter ; a German synonym has probably not yet been established).

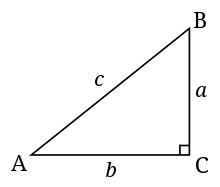
Here is a numerical example with the Pythagorean theorem

If , and are the lengths of the sides of a right triangle, where and are the lengths of the cathetus and the length of the hypotenuse, then applies . ${\ displaystyle a}$ ${\ displaystyle b}$ ${\ displaystyle c}$ ${\ displaystyle a}$ ${\ displaystyle b}$ ${\ displaystyle c}$ ${\ displaystyle a ^ {2} + b ^ {2} = c ^ {2}}$

The distance b is 4 units of length, the distance a is 3 LE. Then c is 5 LE long. Route c is a steep pass road. A navigation system claims that the road user has only covered 4 LE: The map he used for computing purposes is flat; that is, it ignores the height differences covered and thus also the resulting lengthening of the path (in the numerical example: 5 LE instead of 4 LE).

photography

Leica I - 35mm camera (1927) with a rangefinder inserted into the accessory shoe

In photography it is necessary to adjust the lens used to the correct distance to the subject. There are a number of approaches to technically implement this problem. Optical range finders are often used to determine the recording distance. Many are built directly into the viewfinder or are reflected in the viewfinder image.

Flight distances

Radar system

With radar waves, distances between a few centimeters and several million kilometers can be measured, which is why they are often used in aviation. The antenna sends short impulses at a frequency of a few gigahertz (10 ⁹ Hertz ) and measures the time it takes to receive the signal reflected from the target. In addition to the distance, the speed and direction of the object can also be measured.

Instead of radio waves, various types of optical distance measurement are also used. There are flash lamps , lasers and light-emitting diodes are used. Examples are the cloud height measurement with reflected lightning signals from a flashlight, the laser pistol as well as LIDAR and laser triangulation , which also works with a light-emitting diode if the requirements are low.

Underwater and in the ground

Distances under water are usually determined with sound waves , for example with a sonar or echo sounder . For seismographic investigations inside the earth one uses u. a. Compressed air impulse sound sources or explosive charges and determines the running times to several microphones.

Atoms and elementary particles

Atoms and elementary particles fill the space below a nanometer (10 ⁻⁹ meters). The physicists determine the size of atoms and elementary particles with the help of scattering experiments or sophisticated instruments such as atomic force microscopes . If, however, small changes in length are involved, even with very large reference distances , the methods of interferometry can be refined to the same extent as was done in the GEO600 experiment, with an inaccuracy of only 6 · 10 ⁻¹⁹ meters for a reference distance of 600 Meters is one of the most accurate measuring instruments in the world.

Micro and nanometer range

The visible world is limited by the wavelength of light . Things that are smaller than about half the light wavelength of around 0.5 micrometers can no longer be directly observed. However, direct measurements can still be made very well up to this limit. To do this, measuring microscopes are used for vertical measurements or measuring eyepieces for lateral measurements, as well as microscopic scales , so-called object micrometers , which are compared directly with the object size.

The optical distance measurement offers many different methods that are impractical in the field to one micron and even significantly less. The phase shift method enables distance measurements of up to a hundredth of the light wavelength and is used in interferometers or white light interferometers .

Land surveying

For distances of a few kilometers, as must be determined in the national survey , geodetic triangulation is used . The accuracy of the angle measurement and the length of the benchmark determine the achievable measuring accuracy. A triangulation mesh can have a relative accuracy of one millionth (0.000 0001) of the measured length. A survey of Germany , which is around 1000 kilometers long from north to south, would therefore have an error of around one meter. However, the scale error introduced by the reference scale must also be taken into account.

Triangulatory distance sensors work according to the principle of laser triangulation , often a light-emitting diode is used as a beam source if the requirements are low .

Solar system

For very large distances that extend beyond the earth, astronomy has developed a large number of measurement methods, some of which are tailored to very special applications:

Removal of the moon

The laser reflector that was stationed on the moon by the Apollo 11 crew (NASA).

The 2.7 meter Harlan J. Smith telescope of the McDonald Observatory (USA) is used to measure the reflectors stationed on the moon with a laser. (Credit: McDonald Observatory)

The moon has a special position in the distance measurement among the celestial bodies in the solar system.

The major semiaxis of the lunar orbit with about 384,000 km has been known since about 1900 , around 1965 the value was corrected to 384,400 km - also due to more precise earth parameters from satellite geodesy . Long-term changes in the lunar orbit can now be recorded to within a few decimeters.

Since the first moon landing in 1969, an extremely precise determination of the distance has been possible. At that time, the crew of Apollo 11 set up an arrangement of retroreflectors , the so-called lunar laser ranging retroreflector array , on the surface of the moon in Mare Tranquillitatis (see picture). This makes it possible to determine the distance of the moon to within a few centimeters. For this purpose, a laser beam is emitted from the earth's surface (see picture), which is aimed precisely at the reflector on the moon's surface. Due to its structure, the laser beam is reflected exactly back to the transmitter. Normally, only a few photons , sometimes even just one, come back from the emitted signal and can be detected. The distance can then be precisely determined from the time that elapses between the transmission and the return of the reflected signal.

In addition to the reflector from the Apollo 11 mission, four other laser mirrors were later installed on the lunar surface: two by NASA during the Apollo 14 mission (1971 north of Fra Mauro crater ) and the Apollo 15 mission (1971 east at the Hadley groove ). In addition, the two Soviet Lunochod moon vehicles each contained a laser reflector; but unlike Lunochod 2 , which was stationed in 1973 during the unmanned lunar mission Luna 21 in the lunar crater Le Monnier , the reflector of Lunochod 1 was unable to use the reflector from Lunochod 1 as part of the unmanned Luna 17 mission for decades after activities in the Mare Imbrium in 1970/1971 were completed Laser echo can be received more. In March 2010, Luna 17 and Lunochod 1 were finally discovered on images taken by the Lunar Reconnaissance Orbiter . This also made it possible to calculate the parking position: 38.2473 ° N; 325.002 ° E for Luna 17 and 38.32507 ° N; 324.9949 ° E for Lunochod 1. After the recalculation of the parking position, the reflector could be used again. On April 22, 2010, laser measurements were successfully carried out by the Apache Point Observatory .

The measurements, which have been going on for more than 35 years, not only made it possible to determine the distance to the moon extremely precisely, but also gained knowledge in many other areas. So could z. For example, it can be determined that the moon is moving about 3.8 cm away from the earth per year. The reason for this is the tidal friction , which reduces the earth's angular momentum . By precisely measuring the distance, the numerical value of the gravitational constant could also be calculated very precisely. Since the start of the measurements, the values determined in this way have only differed by a factor of 10 ⁻¹⁰ . The validity of the general theory of relativity could also be confirmed by the exact distance measurements. The APOLLO (Apache Point Observatory Lunar Laser-ranging Operation) was founded in 2003 : With the 3.5-meter telescope at the Apache Point Observatory in New Mexico , the accuracy of the data collected so far has been in the millimeter range since 2006.

Radar measurements

For the inner planets Mercury , Venus and Mars as well as the asteroids , astronomers can use active time of flight measurements. In doing so, they use a refined radar distance measurement. However, this fails with objects that are further away because the signal propagation time is too long and the reflected energy is too small.

the Milky Way

For distances that extend beyond the solar system, a method that is also used in land surveying helps first : triangulation , which in astronomy is referred to as trigonometric parallax . With it distances of up to 10 ¹⁹ meters can be determined. That is enough to measure the distance between the neighboring regions of the Milky Way .

The galaxies and the universe

For distances over 1 zettameter (around 100,000 light years) beyond the Milky Way, astronomers use the photometric parallax . So-called " standard candles " are used to calibrate these procedures . These are stars that have a known absolute brightness , from which the distance can then be determined by measuring the apparent brightness . The absolute brightness is known, for example, from the Cepheids , because they have the so-called period-luminosity relationship .

The distance from globular clusters can be determined by means of their color and brightness and the color-brightness diagram .

In order to finally measure the extent of the universe , the redshift of the galaxies is determined. It covers an area of up to around 10 ²⁶ meters.

swell

↑ "The instruments for indirect or optical distance measurement are called distance meters or distance meters or telemeters." P. Werkmeister: Topography: Guide for topographical recording . 1st edition. Springer, Berlin 1930, ISBN 978-3-642-47322-7 , pp. 11 ( limited preview in Google Book search).
↑ Dave Finley, David Aguilar: Fastest Pulsar Speeding Out of Galaxy, Astronomers Discover , National Radio Astronomy Observatory, 2005 html
↑ Karl Stumpff , H.-H. Vogt: The Fischer Lexicon Astronomy . Revised 8th edition. Fischer Taschenbuch Verlag, Frankfurt / Main 1971.
↑ Website of the Geodetic Observatory Wettzell at the BKG : Laser Ranging
↑ AM Abdrakhimov, AT Basilevsky: Lunokhod 1: The position of the first soviet rover. Laboratory for Comparative Planetology, March 17, 2010, accessed March 31, 2010 .
↑ Decades-Old Soviet Reflector Spotted on the Moon ( Memento of April 29, 2010 in the Internet Archive )
↑ Russian classic cars are "revived", FAZ .
^ UCSD Physicists Locate Long Lost Soviet Reflector on Moon , lunarscience.arc.nasa.gov, accessed April 29, 2010.
↑ Measuring the Moon's Distance , LPI Bulletin, No. 72, August, 1994. On-line document .
↑ Homepage of the Apache Point Observatory .

literature

Fritz Deumlich , Rudolf Staiger: Instrument science of measurement technology. 9th edition. Wichmann, Heidelberg 2001, ISBN 3-87907-305-8 .
Walter Grossmann: Angle and distance measuring devices. de Gruyter, Berlin / New York 1983, ISBN 3-11-009601-3 .
Karl Kraus : Photogrammetry . de Gruyter, 2004, ISBN 3-11-017708-0 .
Thomas Luhmann: Close-range photogrammetry . Wichmann, Heidelberg 2003, ISBN 3-87907-398-8 .
McGlone, Mikhail, Bethel (Eds.): Manual of Photogrammetry. 5th edition. ASPRS, 2004, ISBN 1-57083-071-1 .
Rudolf Sigl : Geodetic Astronomy. Wichmann, Karlsruhe 1975, ISBN 3-87907-041-5 .
Hans Zetsche: Electronic distance measurement. Konrad Wittwer, Stuttgart 1979, ISBN 3-87919-127-1 .

Web links

Overview of distance measurement methods in astronomy

[1] "The instruments for indirect or optical distance measurement are called distance meters or distance meters or telemeters." P. Werkmeister: Topography: Guide for topographical recording . 1st edition. Springer, Berlin 1930, ISBN 978-3-642-47322-7 , pp. 11 ( limited preview in Google Book search).

[fastpulsar-2] Dave Finley, David Aguilar: Fastest Pulsar Speeding Out of Galaxy, Astronomers Discover , National Radio Astronomy Observatory, 2005 html

[3] Karl Stumpff , H.-H. Vogt: The Fischer Lexicon Astronomy . Revised 8th edition. Fischer Taschenbuch Verlag, Frankfurt / Main 1971.

[4] Website of the Geodetic Observatory Wettzell at the BKG : Laser Ranging

[5] AM Abdrakhimov, AT Basilevsky: Lunokhod 1: The position of the first soviet rover. Laboratory for Comparative Planetology, March 17, 2010, accessed March 31, 2010 .

[6] Decades-Old Soviet Reflector Spotted on the Moon ( Memento of April 29, 2010 in the Internet Archive )

[7] Russian classic cars are "revived", FAZ .

[8] UCSD Physicists Locate Long Lost Soviet Reflector on Moon , lunarscience.arc.nasa.gov, accessed April 29, 2010.

[LPI_1994-9] Measuring the Moon's Distance , LPI Bulletin, No. 72, August, 1994. On-line document .

[10] Homepage of the Apache Point Observatory .