Geometric figure

from Wikipedia, the free encyclopedia

A geometric figure is a term from geometry that is used inconsistently and often remains undefined. This is often understood to mean certain subsets of the plane or three-dimensional space . Sometimes only figures are meant that are composed of simple parts such as straight lines and circles , sometimes complex subsets such as fractals are also included. The term is used in both Euclidean geometry and non-Euclidean geometry .

Definition and demarcation

Example of a geometrical figure that cannot be clearly represented as a subset: A segment together with a point on it

In geometry, spaces such as the two-dimensional plane or the three-dimensional space are understood as sets of points . A geometric figure is then a subset of such a space, i.e. a set of points .

Not covered by this definition as a subset are further structures such as B. an ordered pair of points, because for two points the sets and are equal.

Another example: A range with a point on . Two different choices for lead to the same subset of the plane, namely the line , are therefore identical as figures in the sense defined above.

Overview and examples

Flat geometric figures

In addition to individual points in the plane and the entire plane itself, the simplest figures are the straight lines . In affine geometry , points and lines are referred to as affine subspaces and a dimension is assigned to them. Points are then zero-dimensional and lines are one-dimensional subspaces of the two-dimensional affine plane. Certain subsets of straight lines also play an important role in geometry, namely the lines between two points and the half-straight lines .

Examples of flat geometric figures: pentagon , square , triangle and circle

The figure class of the polygons is obtained by connecting at least three points by stretching them. Even the simplest polygons, the triangles , allow rich geometric definitions and sentences (see also triangle geometry , trigonometry ). Triangles also play an important role because polygons with more than three corners, i.e. squares , pentagons , hexagons , etc., can always be broken down into triangles.

Often considered special cases of polygons can be defined by additional conditions for distances and angles. In the case of the regular polygons , all sides are of the same length and, moreover, all angles between adjacent sides are the same. With three corners there are equilateral triangles , with four corners squares . Overturned regular polygons, such as the pentagram , are also called stars . Other special types of triangles are the isosceles with two sides of equal length and the right-angled ones with a right angle . A square with four equal (and then necessary right) angles rectangle called a quadrilateral having four sides of equal length diamond . A parallelogram is a square in which the opposite sides are parallel .

Circles can also be defined with the aid of the concept of distance , namely as a set of all points that are at a fixed distance from a given point. Since constructions with compasses and rulers are of great importance in classical geometry , circles, along with straight lines, are among the fundamental figures in geometric problems. Like the circle, the other conic sections , namely ellipses , parabolas and hyperbolas , can also be defined by elementary geometric distance conditions. For example, the ellipse is the set of all points for which the sum of the distances to two given points is equal.

The conic sections can be described in coordinates by polynomial equations of the second degree: They are so-called quadrics . Examples of curves defined by higher-order equations are the Cartesian leaf or the Cassinian curves . Alternatively, curves can also be described as paths using parameters . This display form can be used, for example, to examine different types of spirals or cycloids . The latter are created geometrically by rolling circles on straight lines or other circles.

Spatial geometric figures

Spatial curves (here a helix ) are also spatial geometric figures

As in the plane, the affine subspaces (points, straight lines and planes) in three-dimensional Euclidean space are the simplest geometric figures together with lines and half-lines. As subsets of levels in space, all level figures can also be understood as figures in space. Lines can also be combined to form closed or open spatial polygons . In general, one can also consider curves in three-dimensional space, such as the helix or node .

Examples of spatial geometrical figures: sphere , pyramid , cube , torus , hollow cylinder , circular cylinder , cone and a knotted torus

The two-dimensional polygons correspond to the polyhedra in space , that is, geometric bodies that are only limited by flat side surfaces. The most regular polyhedra are the Platonic solids , which are characterized in that all of their faces are congruent regular polygons. The mathematicians in ancient Greece already knew that there are exactly five Platonic solids: tetrahedron , cube , octahedron , icosahedron and dodecahedron . Another class of regular polyhedra with high symmetry are the Archimedean solids , such as the cuboctahedron . The complete classification of all strictly convex bodies with exclusively regular polygons as side surfaces was only possible in the 20th century with the Johnson bodies .

Other popular types of polyhedra are pyramids and prisms . A straight prism with a rectangle as its base is called a cuboid . An oblique prism with a parallelogram as its base is called a parallelepiped or spatula.

Generalizations of pyramids and prisms on non-polygonal bases are cones and cylinders . The straight circular cone and the straight circular cylinder are examples of another important class of figures, the solid of revolution . They also include the torus , which is created by rotating a circle around an axis located in the plane of the circle.

The three-dimensional analog of the circle, i.e. the set of all points in space that are the same distance from a given point, is the sphere . It can also be generated as a solid of revolution, namely by rotating a circle around a diameter. The sphere is the most important case of a quadric in three-dimensional space. Other quadrics are the ellipsoids , paraboloids and hyperboloids , which are also called second-order surfaces . The geometric properties, particularly the curve characteristics, general surfaces are in the mathematical subfield of (elemental) differential geometry examined. Areas can be specified as a solution set of equations or by means of parametric representations.

Non-Euclidean geometric figures

This article or section needs to be revised: instead of defining non-Euclidean geometry, something should be said about geometrical figures such as hyperbolic triangles.
Please help to improve it , and then remove this flag.

Spherical triangle as an example of a non-Euclidean geometric figure

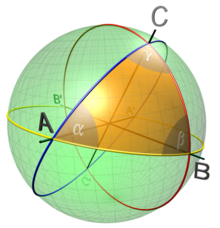
In the non-Euclidean geometries , which are specializations of absolute geometry , but in which the axiom of parallels does not apply, the geometrical figures have partly different properties. The interior angle sum of a spherical triangle is more than 180 ° and it can also contain three right angles. A "square" on a spherical surface is defined by four equal sections of great circles. Its sum of angles is always greater than 360 °.

A "square" on a spherical surface with four angles of 120 ° each.

Polygons in hyperbolic space or the hyperbolic plane have an angle sum smaller than in Euclidean geometry.

Fractal geometric figures

Set of Mandelbrot as an example of a fractal geometric figure in the plane
  • The Koch curve is generated by the infinite iteration that starts with a single segment. This is replaced by a figure composed of four lines. Each of the smaller parts of the route is replaced by a reduced copy of this figure. If this process is continued indefinitely, the Koch curve is finally created.
  • The Gosper curve is created according to a similar principle . But here it is always replaced by a seven-sided figure.
  • The kite curve describes the shape you get if you always fold a long strip of paper in the same direction in the middle and then make every bend to a right angle when unfolding it. Like the Koch curve, it can also be generated by repeated replacement.
  • To get the Sierpinski triangle , you start with an equilateral triangle and divide it into four smaller equilateral triangles by connecting the side centers. Remove the middle triangle and proceed with the other three triangles in exactly the same way as with the starting triangle.
  • The Menger sponge is constructed almost like the Sierpinski triangle, but instead of a triangle, a cube is divided into 27 smaller cubes, from which the six middle cubes on the sides and the central cube are removed. Proceed in exactly the same way with the 20 remaining cubes.
  • For the Mandelbrot set , a recursive sequence for many steps is calculated. If the value (of the complex number ) does not approach infinity (a finite limit is chosen for practical calculations), then this complex number belongs to the fractal.

Similar issues

  • Impossible figures are graphically two-dimensional, allegedly three-dimensional constructs that cannot physically exist.

literature